
Opinionated History of Mathematics
Intellectual Mathematics
History of mathematics research with iconoclastic madcap twists
- 56 minutes 39 secondsTorricelli’s trumpet is not counterintuitive
There is nothing counterintuitive about an infinite shape with finite volume, contrary to the common propaganda version of the calculus trope known as Torricelli’s trumpet. Nor was this result seen as counterintuitive at the time of its discovery in the 17th century, contrary to many commonplace historical narratives.
Transcript
Torricelli’s trumpet is not counterintuitive. Your calculus textbook lied to you.
You’ve probably heard of this cliché, Torricelli’s trumpet: an allegedly “paradoxical” shape that has infinite area but finite volume. It’s staple example in calculus textbooks. Well, there’s nothing to it, in my opinion. It’s a propaganda lie. Let’s revisionist-history the heck out this thing.
I will tell you why there’s nothing counterintuitive about this result. Then I’ll argue that it was not seen as counterintuitive at the time, in the 17th century, contrary to what everyone tells you. Then I will explain, in terms of the sociology of the mathematical community, why this myth is still so popular. That is to say, why it is such a comforting myth to so many people, despite being wrong.
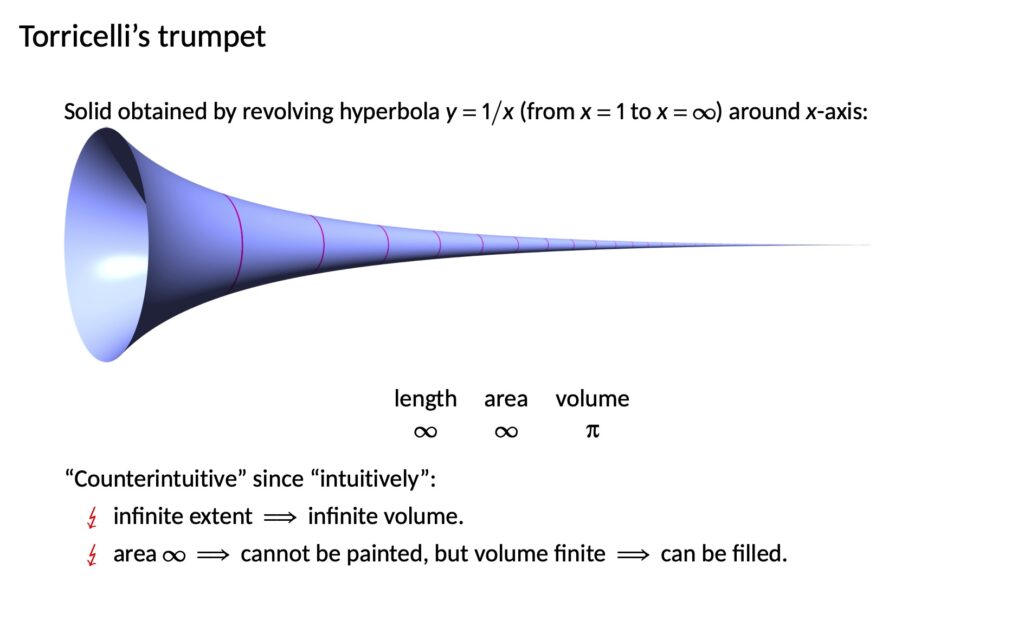
So, the trumpet. You take the hyperbola y=1/x and you rotate it about the x-axis. It makes a trumpet shape, a kind of funnel that becomes infinitely narrow the further you go.
Also known as “Gabriel’s Horn.” Actually I tried to look up the origin of this silly name but I couldn’t find it. I guess it was perhaps coined for the American market? “Torricelli” is bit too “Euro” isn’t it? Now, “Gabriel’s Horn” on the other hand, there you have a nice pious biblical name.
Anyway, whatever you want to call it. The volume of the funnel is finite. For example from x=1 onwards. Despite its area and its length being infinite.
There are two supposed contradictions here. On the one hand, finite volume with infinite extent (infinite length) could be regarded as two contradictory properties that it would be surprising to find in the same solid, allegedly. Alternatively, finite volume with infinite area could also be seen as a clash of two incompatible properties: a shape having those two properties at the same time is supposed to be contrary to “intuition,” allegedly. Sometimes it is put like this: Such a solid cannot be painted, since it has infinite area, yet it can be filled with paint, since it has finite volume. But I think this can be a misleading move that mixes the issue of Torricelli’s trumpet with general issues of how infinite processes correspond to everyday experience, which is perhaps a separate source of so-called “counterintuitive” phenomena. The fact is that if Torricelli’s trumpet is supposed to be a surprising result, then the source of the contradiction is supposed to be the properties of volume, area, and length of this shape, and not some secondary intuitions about infinite processes in general.
In my opinion, the properties of Torricelli’s trumpet are not “counterintuitive.”

First, is it counterintuitive for a finite amount of paint to cover an infinite surface? Of course not. Let’s put it like this. Suppose you have a can of paint. Obviously it contains a finite volume of paint, such as one liter. Now, open the can and pour the paint on the floor. Think of a big floor, like a basketball court. Get a spatula and start spreading the paint across as much area as you can.
Where do you think this process will stop? This is mathematical paint. You can spread it as thin as you like. How much area can you cover, if you can spread the paint thinner and thinner and thinner?
What does your “intuition” tell you? Does your “intuition” say that this spreading process with terminate after a certain number of square meters of the floor painted? Of course not. That would be idiotic.
And yet that is precisely what the standard account of Torricelli’s trumpet would have you believe. It is supposed to be “counterintuitive”, the story goes, for a finite volume of paint to cover an infinite area. Well, we have just seen that that premise is idiotic. Obviously a finite amount of paint can spread further and further, as long as you make it thinner and thinner. There is nothing “counterintuitive” about that.
Why would “intuition” say that the process of spreading the paint thinner and thinner would suddenly terminate at some finite bound? Why on earth would it? What would be the “intuitive” reason for why you could spread paint thinner, and then thinner, and then thinner, and then thinner, and then not thinner all of a sudden? Where would this invisible upper bound come from? Why would “intuition” stipulate the existence of such a ghost? It makes no sense.
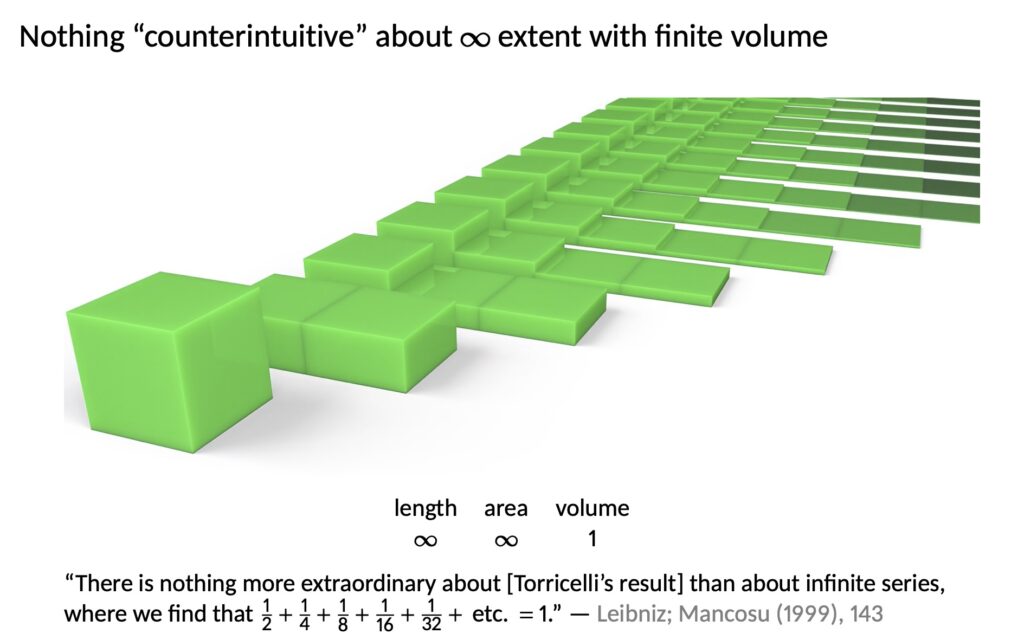
Here’s another thought experiment that proves the same thing. We’re not using paint anymore, but rather a cube. You have a cube of unit volume in front of you. You cut it in half, horizontally. Like a sandwich. Then you place the top half side-by-side with the bottom half. Next cut the top half in half again in the same manner, and bring the new top slice down next to the bottom slice. So now you have a kind of stairway with three steps.
Continue in the same way: you keep bisecting the last piece and placing all the pieces in a row. All the slices have a length and a width of 1, the original dimensions of the cube, because you are always cutting horizontally. You are cutting the heights in half, leaving the width and the breadth of all the pieces the same throughout.
So you get a row of blocks that each have a length and width of 1, and whose heights are 1/2, 1/4, 1/8, 1/16, and so on. The total volume remains 1 throughout the process of course, because you only moved the existing volume around without adding to it or taking anything away. Meanwhile, both the length and the area of the combined shape clearly approaches infinity, as is intuitively clear.
So with this simple intuitive argument we get infinite extent with finite volume. So clearly infinite extent with finite volume is by no means contrary to intuition.
Note that this simple thought experiment also shows that Torricelli’s trumpet is not a case of a technical mathematical result being philosophically or qualitatively different from simple common-sense examples, contrary to what calculus teachers like to pretend. So the standard story is wrong in two ways. It claims that advanced calculus proves intuition wrong. But that’s doubly a lie. First, intuition is not wrong, and furthermore you don’t need fancy calculus to show any of this anyway. The simple thought experiments of the repeatedly bisected cube or the painted basketball court, which you can explain to a 5-year-old, contain everything that is relevant. There is nothing qualitatively new added by the use of calculus.
These things were also well understood historically. For example, Isaac “Barrow … clearly saw that [Torricelli’s] theorem can be intuitively explained by the fact that ‘the infinite diminution of one dimension compensates the infinite increase of the other’.” This fits with the basketball court example. Although Barrow was talking about the relationship between volume and length, not volume and area. But the point is the same.
The equivalent of the bisected cube example was also well understood. For example, Leibniz rightly remarked that “there is nothing more extraordinary about [Torricelli’s result] than about infinite series, where we find that 1/2 + 1/4 + 1/8 + 1/16 + 1/32 etc. = 1.” Exactly. Nothing to see here. Just as Leibniz says.
Let’s turn to history then. Let’s see what people thought back then, besides Barrow and Leibniz. Torricelli published his result in 1641. In fact, all this stuff about area plays no part in the 17th century. The whole thing is about volume only. Torricelli’s trumpet has finite volume and infinite extent, that is to say, infinite length. That’s it. No area, no paint, no painting an infinite area. Nobody said anything about that in the 17th century. Just volume and length.
Still, even then many people today say that this was a big paradox back then. Lots of mainstream accounts of the history of mathematics repeat this story. For example, here is a quote from Simmons’s book Calculus Gems: “That a solid can have finite volume even though it has infinite extent … caused great astonishment at the time.” Another example, from Burton’s History of Mathematics: “The result seemed so counterintuitive and astonishing that at first some of the leading European mathematicians thought it impossible.” And here’s another example, from the book When Least Is Best by Nahin: “Prior to this discovery it was commonly accepted that a surface extended to infinity would necessarily have to be of infinite size, that is, enclose infinite volume.”
So, that’s what everybody says. But here at this podcast we are not in the business of repeating boring clichés like some people, so let’s have a go at it. All of these people are wrong, in my opinion.
I’m going to base my discussion on Paolo Mancosu’s book Philosophy of Mathematics and Mathematical Practice in the Seventeenth Century. This is by far the most complete and authoritative historical account of all of this, of the reception of Torricelli’s trumpet in the 17th century. Mancosu covers the relevant historical sources quite exhaustively. I am going to use the same sources, but I am going to interpret them differently.

Mancosu himself is of the same opinion as all these other guys I quoted, team cliché. According to Mancosu, I quote him, Torricelli’s result “seemed so counterintuitive and astonishing that, at first, some of the leading mathematicians thought it impossible.” “There are good intuitive grounds for holding that an infinitely long solid cannot have a finite volume,” and that is what gave rise to “the intuitive, widespread, and erroneous inference from infinite length to infinite volume.”
So Mancosu is wrong like everybody else, in my view. Let’s look at his evidence and refute it all one by one.

First, Mancosu’s chapter on this stuff is off to an interesting start. He says: “Even eighty years later Bernard de Fontenelle commented: ‘One apparently expected, and should have expected, to find [Torricelli’s solid] infinite [in volume].’”
Ha! Did you catch that rhetorical trick? “Even eighty years later …” “Even”! In fact, instead of “even eighty years later” it would be more accurate to say: not until eighty years later is this allegedly common view actually explicitly asserted, and then by an essayist and not a mathematician. It’s neither here nor there what some non-mathematician wrote 80 years later. He could very well have been subject to the same biases as the modern proponents of the story.

So, let’s look further back in time. What were Torricelli’s own words? Indeed, Torricelli called his result “as it were paradoxical,” in Mancosu’s translation. The Latin is “ut ita dicam paradoxicum.” I think maybe a more literal translation would be “so to speak paradoxical.” “So to speak”: that is to say, not actually.
It’s not a paradox but it’s kind of like a paradox if you squint a bit, Torricelli seems to be saying. Of course that seems to give some credence to the standard interpretation, perhaps the strongest evidence that exists for this view. Fair enough. There is some connection to paradox, like the standard story says. On the other hand, Torricelli’s phrasing is also a notably guarded formulation coming from the discoverer himself. You would think that Torricelli, the very discoverer of this result, would be inclined to play up, if anything, the philosophical significance of his own theorem. Despite this incentive, Torricelli evidently felt the need for the qualifier “so to speak paradoxical.” The result is not actually paradoxical, in other words.
Torricelli then goes on to explain why the result is “so to speak” paradoxical: “The reason is that ‘if one proposes to consider a solid … infinitely extended, everybody immediately thinks that such a figure must be of infinite size’.” Again, this fits to some extent with the standard narrative. But on the other hand it is striking how much more restrained this wording is. The Latin for “immediately” is “statim,” which arguably means something like “the very instant that,” or in other words: before thinking about it for more than a few seconds. Quite plausibly, Torricelli has chosen his words carefully since he is well aware of examples such as that of the repeatedly bisected cube that I described before. Perhaps Torricelli knows well that if he asked a mathematician colleague whether a solid with infinite length must necessarily have infinite volume then they would likely very soon think of such counterexamples and realise that the answer is no. Perhaps that is why Torricelli does not say that his theorem contradicts what everybody believes, or that it contradicts intuition. Instead he merely says, much more cautiously, that his result is “so to speak” surprising since it is different from the first example of a solid with infinite length that pops into someone’s head the very split second that they are asked to imagine such a shape.
Quick! Think of a shape with infinite length! Ok, maybe then you think of a shape that also has infinite volume. But that is not the same as to say that “intuition” says that it must have infinite volume.
Let’s listen to Torricelli’s words again: “if one proposes to consider a solid infinitely extended, everybody immediately thinks that such a figure must be of infinite size.” That is to say, in that moment, when you are asked to picture such a shape, your “immediate” impulse is to think of one that has infinite size. That’s your “immediate” reaction. Not your considered reaction, not your mathematician’s intuition, not what you think after a bit of thought. Only “immediately.” Torricelli has chosen his words wisely, so that it seems like he is saying a lot but in fact he is carefully choosing a formulation that is not subject to refutation based on thought experiments like the ones I gave before, because Torricelli is only talking about “immediate”, split-second visualisation, and he wisely says nothing about what somebody who thought about it for two minutes would think. In this way Torricelli stops well short of asserting the standard view that I quoted from so many modern historians. Torricelli seems to be going in that direction but he doesn’t go anywhere near as far as those statements from the modern literature that I quoted.
That’s all we have to go on in terms of Torricelli’s own words. Now let’s turn to the reaction of Torricelli’s contemporaries.

It is true, as the standard story says, that a number of mathematicians at the time reacted with some surprise to Torricelli’s result. But here we must ask ourselves: Why were they surprised? The standard story assumes that they were surprised because the result was counterintuitive. Therefore contemporary expressions of surprise are often cited as self-evidently supporting the standard story. But in fact there are other good reasons for contemporary mathematicians to have been surprised that have nothing to do with intuitions about infinite shapes.

Torricelli’s work showed that infinite curvilinear figures are mathematically tractable. That in itself was novel and surprising, quite apart from the specific result and whether it was intuitive or not. Indeed, Torricelli explicitly makes this point himself: “Among all the solids of which ancient and modern authors have determined the measures with much effort, none, as far as I know, has an infinite extension.”
Exactly. It was rare indeed for geometers at this time to be able to go qualitatively beyond what the ancient masters such as Archimedes had done, as opposed to supplementing and extending their results with more of the same. In fact, the very nature of the ancient methods for determining areas and volumes, which was still the gold standard in Torricelli’s time, and which he still relied on for proving his result in the most rigorous way, would seem to preclude application to infinite figures, as Torricelli himself noted: “In introducing the proof by exhaustion, Torricelli remarked that it would seem an impossible task to inscribe in another figure a figure of infinite length, or to circumscribe another figure around such a figure.”
In fact, therefore, when we have historical evidence that certain 17th-century mathematicians found Torricelli’s result to be “impossible,” we cannot infer that they therefore regarded the volume result as counterintuitive. Instead, the impossibility in question could be what Torricelli himself called the seemingly “impossible task” of applying rigorous exhaustion methods to infinite figures. And that is a methodological matter entirely independent of the specific content of Torricelli’s theorem and its intuitiveness or lack thereof.
There are indeed some historical testimonies that are ambiguous in this respect. For example, according to Torricelli himself, “Roberval considered this proposition false and impossible; actually, when Father Mersenne came by here he told me that Roberval, having thought about it for some time wrote I don’t know what demonstrations or speeches in order to prove that my proposition was absurd and impossible.” This is as consistent with the standard intuition-centred narrative as with the alternative intuition-independent narrative that I have proposed. Maybe Roberval thought the result was impossible because of his intuition about infinite shapes, or because he though it was not methodologically possible to apply rigorous geometry to such shapes. After all, why had Archimedes not done it, if it could be done?
Other 17th-sources are inconclusive in the same way. For example, Cavalieri said about Torricelli’s result that it is “extraordinary that that could be.” Again a generic expression of surprise. That is not enough to establish a link to any intuition or common conviction that solids of infinite extent ought to have infinite volume. Indeed, Cavalieri wrote to Torricelli that “I do not know how you fished out its measure so easily in the infinite depth of that solid.” So here Cavalieri is clearly highlighting that it is the new reach of the mathematical methods that is surprising rather than the result itself.

There is another sense too in which Torricelli’s result could have been seen as surprising in a way that has nothing to do with geometrical intuitions about infinite volumes. Namely, that the theorem invokes the notion of an actual infinity. Classically, statements involving infinity were often studiously avoided in favour of equivalent finitistic formulations. For example, instead of “there are infinitely many primes,” Euclid says “prime numbers are more than any assigned multitude of prime numbers” (Elements IX.20). But no such finitistic paraphrase was forthcoming in the case of Torricelli’s theorem. As Mancosu says, “The notion of actually infinite length is present in the very statement of the theorem. Moreover, the proofs themselves, as they stand, make sense only if the hyperbolic solid is given as infinitely long in actu.” But “Torricelli’s result is boldly infinitistic,” and efforts by purists “at reducing it to a finitistic framework appear destined to fail.”
One such finitism purist was Hobbes. In his view, as summarised by Mancosu, “any consideration of infinity, both geometrical and arithmetical, ought to be banished. Hobbes claimed that when mathematicians use the word ‘infinite’, what they usually mean, or ought to mean, is ‘indefinite’, that is, as great, or small, as one pleases.” “In Hobbes’ mathematical universe …, to talk about a solid ‘whose sides are extended out to infinity is absurd’.”

This is certainly a crucial background to keep in mind when interpreting Hobbes’s comments on Torricelli’s result. Hobbes claimed that “to understand [Torricelli’s theorem] for sense, it is not required that a man should be a geometrician or a logician, but that he should be mad.”
This striking quote is often connected with the intuition narrative. For example, The Cambridge Companion to Hobbes says: No, it is not necessary to be mad to accept Torricelli’s result. “Rather, of course, it is required that one should see, as Hobbes sometimes failed to do, that mathematics can raise and resolve problems where physical experience is lacking and physical intuition may deceive.” That’s the opinion of The Cambridge Companion to Hobbes, which is in line with the standard view.
But note indeed that Hobbes is not claiming that the theorem is contrary to “physical intuition.” Instead he says that it has no “sense.” This is in line with the standard finitistic position in philosophy that statements involving infinity have meaning (or “sense”) only when they can be, and are, reformulated in finitistic terms.

But, this point aside, there is one final passage in Hobbes that Mancosu regards as an outright explicit affirmation of the standard intuition-centred interpretation, leading Mancosu to confidently assert that “Hobbes believed that natural light teaches us that an infinitely long solid, however subtle, must exceed in volume any finite solid.” “Natural light” is indeed a synonym for intuition, so, if substantiated, this would make a strong case for the standard narrative. The Hobbes passage in question is the following.
“It has been demonstrated, they say, by Torricelli that a certain acute hyperbolic solid, in this sense of infinite [namely, greater than any assignable measure], is equal to a [finite] cylinder. … The distance which Torricelli supposes infinite is to be understood as indefinite. Nor could it be understood differently by him, who in quite many demonstrations uses Cavalieri’s principle of indivisibles, which are such that their aggregate can be equated to whatsoever given magnitude. Therefore, a proposition as absurd as this that the infinite is equal to the finite, must not be attributed to Torricelli. In fact, as it is clear by the natural light, there cannot be a solid so subtle which does not infinitely exceed every finite solid.”
So those are Hobbes’s words, and according to Mancosu’s interpretation he is saying that Torricelli’s result is counterintuitive.
But if you parse the quote you see that there a number of problems with Mancosu’s reading of this passage. For one thing, note that Hobbes clearly connects his conclusion to Torricelli’s use of Cavalieri’s principle, that is to say, his method of proof, by means of infinitesimals or indivisibles. But if Hobbes is trying to say that the result itself is counterintuitive, then why does it matter what method was used to prove it? Hobbes is saying that something is contrary to “natural light,” which means contrary to intuition, but what exactly? The passage is not so clear, but apparently the point is connected to the method of proof, and not merely to the content of the theorem itself.
Indeed, the crucial final sentence of Hobbes’s passage is difficult to parse altogether. It appears to be nonsensical on the face of it. The thing that is evident by “natural light” or intuition according to Hobbes is that: “there cannot be a solid so subtle which does not infinitely exceed every finite solid.”
This sounds crazy. It appears to say that any “subtle” (that is to say, small) solid must be infinite, which is obviously nonsense. I checked Hobbes’s original Latin but unfortunately that’s just as ambiguous and it doesn’t clarify anything.
For Mancosu’s reading to work, it seems that this crucial sentence, about any “subtle” body being infinite, must be interpreted as follows.
The passage starts:
“There cannot be a solid…”
Here, for Mancosu’s reading, we should think of Torricelli’s trumpet as the solid in question.
“There cannot be a solid so subtle…”
“Subtle” here then means thinning out or tapering off, as the trumpet does when it goes to infinity.
“There cannot be a solid so subtle which does not infinitely exceed every finite solid.”
So, first of all, for this not to be nonsense we must, for Mancosu’s reading to work, we must insert an extra clause that must be regarded as implicit in Hobbes:
Instead of: “There cannot be a solid so subtle which does not infinitely exceed every finite solid.”
We must read: “There cannot be a solid [that is infinitely extended and yet is] so subtle which does not infinitely exceed every finite solid.”
If we twist the passage like this then indeed it does say that the volume of Torricelli’s trumpet must exceed any finite volume. That is to say, it must be infinite. That is how Mancosu wants to read it.
I don’t agree. I will propose a different interpretation. But first let’s summarise the problems with Mancosu’s interpretation. First there is the connection to the method of proof, that is to say to the use of infinitesimal slices. Hobbes says: Torricelli used Cavalieri’s method and “therefore” etc. Hobbes’s conclusion is a consequence of the method of proof used. Which is not reflected in Mancosu’s interpretation of the concluding sentence. Mancosu’s interpretation only has to do with the statement of the theorem and not with its proof.
Furthermore, for Mancosu’s reading to work we had to insert that the solid Hobbes was talking about had to be infinite in extent even though Hobbes doesn’t say that. We would have to regard that as implied by context. Which is certainly questionable.
And one more thing: I don’t know if you caught this but Hobbes used the phrase “infinitely exceed every finite solid.” In Mancosu’s reading he’s saying: Any infinitely extended solid, no matter how thin it gets or how much it tapers off, will “infinitely exceed every finite solid.”
Why “infinitely”? That’s completely redundant isn’t it? You could just as well have just said: it exceeds every finite solid. If you exceed every finite solid you are infinite already. Of course if you only finitely exceed some finite solid then you are obviously yourself finite. So of course you cannot then exceed every finite solid. So if you exceed every finite solid, then you also automatically exceed every finite solid by infinitely much, so to speak. Because if you exceeded one of them only by a finite amount then you couldn’t also exceed all the other ones.
So Mancosu’s reading doesn’t explain why Hobbes uses this redundant word “infinitely” which doesn’t add anything and doesn’t change the meaning in any way. This word could have been omitted and the sentence would have meant exactly the same thing, according to Mancosu’s interpretation. Although Mancosu himself doesn’t mention that, of course.
Now I am going to propose my alternative reading, which avoids all of these problems.
Hobbes’s sentence starts:
“There cannot be a solid…”
Here I claim that we should not in fact think of Torricelli’s trumpet. Instead, the solids Hobbes is thinking of here are the infinitesimal components dV, the Cavalieri slices, of the trumpet volume. In other words, the slices that are used to calculate the volume, basically calculus-style. These dV’s, these infinitesimal components of volume, are what Hobbes is speaking about, I claim.
Let’s continue reading Hobbes’s sentence:
“There cannot be a solid so subtle…”
That is to say, so small: dV = epsilon. We are doing a finitistic paraphrase of a calculus argument. A limit-style paraphrase. Where the volume element dV is some very small real number epsilon.
Back to Hobbes:
“There cannot be a solid so subtle which does not infinitely exceed every finite solid.”
There cannot be any epsilon that is so small that it does not, when taken infinitely, that is to say added to itself any number of times, does not eventually exceed any given real number. This is the Archimedean property of the real numbers, in other words.
This is why we need the word “infinitely” that didn’t serve a purpose in Mancosu’s interpretation. The little number epsilon, the dV, is, taken singly, just a very small thing of course, but taken “infinitely” (that is to say added together over and over and over again) it eventually becomes greater than anything.
This reading explains why the link with the Cavalierian method of proof is crucial to the point, just as Hobbes seemed to indicate. It also explains why the phrase “infinitely exceed,” as opposed to merely “exceed,” is needed. It also does not require a crucial phrase that dramatically impacts the meaning to be postulated as implicit.
According to my reading, Hobbes’s statement is in effect simply an assertion of the Archimedean property of the real numbers, which was a well-known axiom that many greater mathematicians than Hobbes did not hesitate to regard as evident by “natural light.” Therefore Hobbes’s claim, on this reading, is correct and unproblematic, and does not have anything to do with physical or geometrical intuitions about infinite volumes.
Nor is Hobbes’s point an impactful critique of Torricelli’s reasoning for that matter, since Torricelli does not rely on non-Archimedean assumptions in the manner Hobbes seems to be trying to suggest. But that is beside the point for our purposes.

This concludes my analysis of the historical reception of Torricelli’s theorem. I think there is very little evidence to say that it was regarded as counterintuitive at the time, contrary to the standard narrative in the literature. What I have discussed is essentially all the evidence. I have systematically gone through basically all passages from 17th-century sources that are commonly cited in support of the standard narrative. There’s nothing more. There is no smoking gun anywhere. There are only these passages, all of which can be interpreted very differently than in the standard story, as I have shown.
Now I will add a little speculation about why I think the myth of Torricelli’s trumpet is so popular. Basically, I believe this is ultimately because the way we teach calculus is poorly motivated.
Modern calculus textbooks are monstrosities. They mix classical calculus with modern real analysis as if they were one and the same subject, which they are not.
One moment you are doing applications of integration by parts, and the next moment you are thinking about whether continuity implies differentiability.
These are both great subjects. I love calculus: going nuts with integration by parts, applying it to a bunch of differential equations and physics and stuff. Wonderful.
And I love real analysis. Such an elegant, unified theory that brings such razor clarity to so many tricky questions. “Baby Rudin” is a work of art. It is stylistic perfection.
But these are two separate subjects. Modern calculus textbooks don't want you to know this. Modern calculus textbooks pretend that real-analysis-style rigor is the correct and appropriate lens through which to understand classical calculus. Which it is not.
Mathematicians who teach calculus pretty much know this in their heart of hearts, even if they try to repress it. They know that the need for real-analysis-style rigour in calculus serves no reasonable purpose in that course. They know that you could do the entire calculus course completely unrigorously and it would be perfectly fine.
Unlike real analysis, of course. You couldn’t teach that course intuitively or informally. Doing it formally is precisely part of the core of what makes the subject great. Formal rigour in real analysis is very well motivated. It is a beautiful course for that reason.
But formal rigour in calculus is largely pointless and serves pretty much no purpose within calculus itself. The so-called rigorous approach to calculus didn’t exist for the first 200 years of calculus, when all the best applications of calculus were worked out by all the best mathematicians of the day who were the most accomplished calculus practitioners the world has ever seen, perhaps more so than anyone alive today because there are no research professors of calculus anymore.
Mathematicians who teach calculus know all of this. The know that the calculus has always thrived without rigour. They know that every single one of the great applications of calculus were developed with complete disregard for real-analysis-style rigor. At some level they know this, at least subconsciously. They may try to suppress this knowledge because it makes their life easier.
They have to teach real-analysis-style proofs in calculus courses, so it would be easier for them if doing so was a good idea. Otherwise they are torturing students with lots of difficult technicalities that are poorly motivated.
You can see now why Torricelli’s trumpet is a godsend to these people. Supposedly, Torricelli’s trumpet shows that intuition cannot be trusted. In other words, it motivates taking much more formal and rigorous approach to calculus.
Phew! As an instructor you had seen your students suffering from the overly formal approach, and you had felt bad about it. Maybe in a moment of weakness you had even wondered to yourself why indeed we have to teach calculus this way, although you would certainly keep those kinds of speculations to yourself.
It is a weight on your conscience as a calculus instructor. Am I just torturing my students with vastly excessive technicalities for no good reason?
What you need to be able to sleep well at night is a nice self-rationalising story that tells you that you are doing the right thing. “Ah, well, you see, this rigour may be a bitter medicine for some but you need it! It’s for the best! It’s for your own good!”
And how do we prove that rigour in calculus is actually a good thing? Oh well, a good example is Torricelli’s trumpet, you see.
These people desperately need the standard story of Torricelli’s trumpet to be true. It is exactly the self-rationalising justification they need to keep teaching the way they do and convince themselves that their conscience is clear. It is a necessary myth.
That is why so many people do not want to know the real history of Torricelli’s trumpet. They would rather have the comforting myth that flatters the status quo.
30 December 2024, 10:54 am - 1 hour 27 minutesDid Copernicus steal ideas from Islamic astronomers?
Copernicus’s planetary models contain elements also found in the works of late medieval Islamic astronomers associated with the Maragha School, including the Tusi couple and Ibn al-Shatir’s models for the Moon and Mercury. On this basis many historians have concluded that Copernicus must have gotten his hands on these Maragha ideas somehow or other, even though no direct evidence for such transmission has been found. Let us consider the evidence as to whether Copernicus plagiarized these Arabic sources or not.
See PDF slides for figures and references.
29 November 2023, 10:32 pm - 1 hour 16 minutesOperational Einstein: constructivist principles of special relativity
Einstein’s theory of special relativity defines time and space operationally, that is to say, in terms of the actions performed to measure them. This is analogous to the constructivist spirit of classical geometry.
Transcript
Oh no, we are chained to a wall! Aaah! This is going to mess up our geometry big time. Remember what Poincaré said: self-motion is the essence of geometry. We understand that part of the environment to be geometrical that we can cancel through self-motion, through a change of perspective.
Suppose you are looking at a chair, let’s say, and somebody tips it over so that it’s laying on its side, or somebody moves it to the other end of the room. Those are geometrical transformations: rotations and displacements in space. They are the equivalence relations of space; the isometries: things you can do without changing metric relationships.
You know that these are geometrical equivalence transformations because you can cancel them through self-motion. When the guy knocks the chair over, you can tilt your head 90 degrees, and you have restored the original visual impression of the chair. And if the guy moves the chair five meters that way, then you yourself can move five meters in the same direction and once again the chair makes precisely the same visual impression on your retina as it did before.
This is how you know that rotations and displacements are geometrical equivalence transformations. The more you accumulate experience with these kinds of scenarios, the more you begin to grasp the group of geometrical transformations as a whole. You get a global sense of what kinds of transformations are possible, how they combine and interact, and so on. This process might lead you to Euclidean or non-Euclidean conceptions of space depending on your experiences. You get to know space and what kind of geometry it has by getting to know its transformation group: that is to say, what kinds of rotations and displacements exist, what happens if you do one after the other, and so on.
Now, what about the scenario when we are chained up? We must imagine that we have been chained to this wall for life. We don’t know any other reality than this.
Our sense of what geometrical transformations are possible will be very different. There is still geometry because there are still visual impressions that we can cancel through self-motion. If an object is moving across our field of view, we can keep the retinal impressions the same by tracking it with a motion of our eyes. So we understand the geometry of sideways motion well since we can move our eyes from left to right, or point our gaze in different directions.
We also understand the geometry of depth to some extent. If an object is moving away from us, we can keep track of that through self-motion also, but of a very different kind. They eye has a lens in it. The curvature of the lens is variable and is controlled by a muscle. Depending on whether you need to focus on objects that are near or far, the muscle will pinch or pull the lens so that it is more round or more flat in order to have the right focal distance for the object you are looking at. In this way you can keep track of how much an object has moved in depth by recording how much the lens needs to be adjusted to restore focus. So this gives you the data to develop a geometry of depth.
So our chains do not deprive us of geometry altogether. We can still develop the geometry of width and the geometry of depth. But these are separate geometries to us. A free person will know that width and depth are merely two dimensions of the same kind of thing. They are both spatial dimensions. They are interchangeable and homogenous. The free person will know that since they can turn width into depth by self-motion. They just need to go stand over there and the old width is the new depth and vice versa.
But we who are chained are deprived of this experience. So to us width and depth remain qualitatively different kind of things altogether. Indeed, we measure distance in width and distance in depth completely different units. We count distance in width by the direction in which our eyes are pointing, so the unit is degrees for example. An object is 30 degrees to the left of another, for example, we might say. But we count depth by how much the lens needs to be bent to achieve focus. So the unit is something like a unit of force corresponding to the muscular effort involved. That’s a completely different kind of thing altogether, and cannot be compared with our degree measures that we used to quantify position in the width direction.
It’s not so strange that width and depth would be qualitatively different things. You already treat various measurements of the same object as qualitatively different in your everyday life. For example, suppose somebody asked you: Is this building wider than it is old? Of course that doesn’t make any sense. You cannot compare a distance in space with a duration in time. Because those quantities are determined in fundamentally different kind of ways, they are measured in completely different kinds of units, and so on. Well, just as you think time and space are not comparable, so the chained person thinks depth and width are not comparable. Samesies.
In fact, maybe you are are just as delusional as the chained guy, and for much the same reason. Actually time and space are a lot more comparable and interchangeable than you think, as Einstein’s theory of relativity says. We don’t realise this in our everyday experience, because relativistic effects become significant only at high speeds, somewhat close to the speed of light. Compared to the speed of light you have practically been standing still your whole life, even when flooring it on the highway. So you might as well have been chained to a wall. The sum total of all your visual and sensory impressions are severely and systematically impoverished just like the guy chained to a wall. Just as he doesn’t realise the fundamental unity of width and depth, so you don’t realise the fundamental unity of time and space. And for the same reason: you are both essentially standing still.
I took this example from Feynman’s famous lectures on physics. Why don’t we listen to his version as well? The classic Feynman lectures on physics are nowadays available for free at a Caltech website, audio recordings and all.
“When we look at an object, there is an obvious thing we might call the ‘apparent width’, and another we might call the ‘depth’. But the two ideas, width and depth, are not fundamental properties of the object, because if we step aside and look at the same thing from a different angle, we get a different width and a different depth, and we may develop some formulas for computing the new ones from the old ones and the angles involved. … If it were impossible ever to move, and we always saw a given object from the same position, then this whole business would be irrelevant—[width and depth] would appear to have quite different qualities, because one appears as a subtended optical angle and the other involves some focusing of the eyes …; they would seem to be very different things and would never get mixed up. It is because we can walk around that we realize that depth and width are, somehow or other, just two different aspects of the same thing.
[In Einstein’s theory of special relativity] also we have a mixture---of positions and the time. … In the space measurements of one man there is mixed in a little bit of the time, as seen by the other. Our analogy permits us to generate this idea: The ‘reality’ of an object that we are looking at is somehow greater (speaking crudely and intuitively) than its ‘width’ and its ‘depth’ because they depend upon how we look at it; when we move to a new position, our brain immediately recalculates the width and the depth. But our brain does not immediately recalculate coordinates and time when we move at high speed, because we have had no effective experience of going nearly as fast as light to appreciate the fact that time and space are also of the same nature. It is as though we were always stuck in the position of having to look at just the width of something, not being able to move our heads appreciably one way or the other.” (I.17-1)
I love this thought experiment with the chained guy. Plato’s cave 2.0. And it is perfect for our purposes today. This is going to be the concluding episode of my history and philosophy of geometry story arc, and the theme will be how everything goes full circle and the beautiful ideas from days of old are as relevant as ever to us self-absorbed moderns as well. The guy chained to a wall is a perfect backward-looking example, and a perfect forward-looking example. Back to the operationalism of Greek geometry, and forward to Einsteinian modernity.
We started, way back when, with the Greeks and their ubiquitous ruler and compass. Always with the making, those guys. Lines and circles are nothing but the things you get when you draw with these tools. Not abstract things, not axiomatically defined things. Lines and circles are operations. They are things you do.
The Greeks realised that this was the rigorous way to do mathematics. The epistemological humility of the maker is far superior to hubris of the philosopher who think they can concoct a perfect theoretical system in the abstract using the power of their mind alone. People are not as good at that as they think. Time and time again, somebody’s pretentious abstract theory has proved to contain various unintended contradictions and unnoticed assumptions. As the Greeks knew all too well: the works of Plato and Aristotle do little else than poke holes in other people’s bad theories. So we should stop trying to philosophise about essences, which we are so bad at, and instead roll up our sleeves and build stuff. Clear the junk off the table in your garage, put your tool belt on, and let’s double some cubes.
To know is to do. And this runs all the way through history. In physics, we can only know relative space, not absolute space, said Descartes and Leibniz, because we can measure the distances between things, but we cannot measure any such thing as the absolute “coordinates” of any one thing in itself without reference to anything else. So, if we believe in the virtue of the humble maker and the hubris of the speculative philosopher, then it follows that we must base our physics on relative space, not absolute space. A very reasonable conclusion, which Newton abandoned to the dismay of many at the time.
So if we stick to the classical point of view then space is what you can make, and what you can measure. What you can experience, in other words. This is a good philosophy of space. And no wonder. The Greeks, Descartes, Leibniz—back then mathematical and philosophical sophistication went hand in hand to a rare degree. So it’s no wonder they had some good ideas.
But don’t take my word for it. What makes a philosophy good? Not the say-so of some podcaster, that’s for sure. But we can prove that the classical operationalist perspective was good philosophy, by considering how it fared in the face of entirely new developments. Bad philosophy is always back-pedalling. As soon as new facts come in you have to go: uh, well, actually what I mean was… Or just descriptive: some people think they have a philosophy of something when they are just describing its basic features and making up a name for each part. But good philosophy is not that. Good philosophy is a perspective that makes you think in new ways. It gives you tools that you can use to try to understand conceptually challenging new problems. Philosophy is good if it is a fruitful way to think in challenging new situations.
Such as non-Euclidean geometry, for example. A rather counterintuitive new world; we could really use some philosophy to find our feet here. What philosophy is going to help us? Maybe Aristotle’s four different names for four different kinds of causes? Yeah right. But thinking of space and geometry in terms of operations: now that’s a philosophy. And it will prove its worth by the way it interacts with these new developments.
How do we know whether we live in a Euclidean space or a hyperbolic space? Not by developing these two geometries abstractly and axiomatically, and then testing them by their angle sum theorems or whatever. No thank you, that would be that hubristic assumption again, that we could develop geometry purely in the abstract, in the mind alone. Geometry should come from experience. But how? Modern mathematics has told us exactly how. A geometry is defined by its group of equivalence transformations, as Felix Klein said in his famous Erlanger Program. And a group of equivalence transformations can be defined in terms of experience. That is what Poincaré explained: equivalence transformations are the transformations you can cancel through self-motion. Perfect! In this way the difference between Euclidean and hyperbolic geometry emerges organically from experience itself. There is no need to postulate a hubristic ability of the human mind to develop axiomatic systems in the abstract.
Later we can go on to do more conventional abstract axiomatic mathematics as well, of course, but we do that by building on the concrete substrate developed first. We are not born with general-purpose abstract reasoning skills. We have domain-specific innate abilities such as that of acquiring a geometry by extracting the group of equivalence transformations of the space we live in from our sensory experience. And, insofar as we eventually succeed at general abstract reasoning, that is because we have mobilised our domain-specific skills and modes of thought to simulate abstract general-purpose thought. This is the point of view that I associated with Poincaré and Chomsky if you recall.
The chained guy is a perfect example to illustrate this entire tradition on geometry going all the way back to the Greeks. Restrict the operations a guy can perform, and you restrict his geometry.
I think maybe Feynman didn’t realise that his thought experiment perfectly illustrates this historically rich point of view. If we assume that Feynman came up with this through experiment himself, it seems that he started with Einstein’s relativity theory and asked himself how he could illustrate it using an analogy. Then the idea that the concepts of a physical theory depend on the kinds of experience one has, or the kinds of measurements one can make, comes off looking a bit like a kind of quirky side-effect of relativity theory. Rather than a methodological axiom built in to it from the very beginning, and indeed an axiom already strongly established long before relativity theory was even conceived.
We can see this in another one of Feynman’s remarks, in another lecture. Let’s listen to this, and pay attention to what causes what. What comes first: the physics or the philosophy?
“One of the consequences of relativity was the development of a philosophy which said, ‘You can only define what you can measure! Since it is self-evident that one cannot measure a velocity without seeing what he is measuring it relative to, therefore it is clear that there is no meaning to absolute velocity.’” (I.16-1)
One could argue that it was the other way around. This way of thinking was not a consequence of relativity theory, as Feynman says.
“One of the consequences of relativity…”
If anything, relativity theory was a consequence of this way of thinking.
“The physicists should have realized that they can talk only about what they can measure.”
Yes, they should have realized that, and they did! Not from Einstein but thousands of years before.
Indeed, Einstein read a lot of that stuff in his youth, including Ernst Mach and Poincaré. And he made no secret of how much those things influenced him. Relativity theory was a philosophy-driven scientific development to an unusual degree.
Without Poincaré’s beautiful philosophy of space, no Einstein maybe. Feynman thought the guy chained to a wall was a perfect thought experiment to describe Einstein’s theory. Yes, but it is equally perfect to describe Poincaré’s philosophy of space, which came before Einstein’s theory.
Actually Feynman was right too, when he said that the success of Einstein’s theory led to these operationalist philosophical conclusions. It did indeed. But not because science developed in its own autonomous, technical way, and then after the fact people went: huh, I wonder if we can draw some philosophical conclusion from this new science? It wasn’t like that. It was a revival of old philosophy rather than a new start stimulated by new science. Einstein’s theory didn’t so much rectify the course of philosophy, as much as it showed that the philosophers had been right all along, somewhat embarrassingly.
Remember how Newton’s absolute space was criticised. By Leibniz for example, but also others at the time. Only relative space makes epistemological sense. Only relative space is knowable. Because only relative space is measurable. Or in other words, only relative space can be operationalised.
Operationalisation is a way to ensure consistency, as the classical constructivist tradition in geometry knew. There are two ways to introduce objects in mathematics: construction, or wishlist to Santa Claus.
“Let ABC be the figure you get when you …” This is how to introduce objects by construction. “First I raise this perpendicular to that line, then I cut off a length here equal to that length over then, then I connect these two points” etc. That is the honest way to do things. The object is defined by the recipe for making it. An object is nothing but the outcome of certain operations that you perform yourself.
The other way is lazier and easier. “Let ABC be a figure such that…” This is a wishlist to Santa Claus. You state what it is that you want: “Let ABC be an equilateral triangle.” “Let ABC be a triangle with three right angles.” “Let me have a flying car and unicorn pony.” You state the properties that you want an object to have, and like a spoiled child you assume that you are thereby entitled to the object in question.
Newton was like the spoiled child asking for a unicorn. His new physics demanded absolute space and time, which were merely postulated, or wishlisted really, and cannot be operationalised.
So people like Leibniz objected, very reasonably. Newton’s physics is built on concepts that are unknowable. And it is exposed to the risk of containing inconsistencies and contradictions, since it is not susceptible to operationalisation, which has been the best way to ensure consistency since the days of Euclid. There are no unicorn ponies or triangles with three right angles, but a spoiled child wouldn’t know that, would he? Because he is not constrained by what is actually doable. So maybe Newton’s physics is ultimately incoherent since it has not taken steps to ensure otherwise.
This critique of Newton was philosophically sound, but it soon looked absolutely ridiculous. Newton’s physics was a runaway success like the world had never seen. And then you had these ridiculous little philosophers going: “well, actually, that’s actually bad science because blah blah blah.” Who would listen to such clueless nitpicks? Read the room, nerds. Newton has already won. Nobody cares about your stupid “well, actually.”
Let’s quote David Hume, for example, who was one of those philosophy losers in the 18th century. “[A] notion … beyond what we have instruments and art to make, is a mere fiction of the mind, and useless as well as incomprehensible.” Such as absolute space, for example. We have no “instruments and art”—that is to say, no physical experiments or observations—that can detect absolute space. So it must be a “useless fiction of the mind.”
Here’s another passage where Hume says the same thing: “When we entertain, therefore, any suspicion, that a philosophical term is employed without any meaning or idea (as is but too frequent), we need but enquire, from what impression is that supposed idea derived? And if it be impossible to assign any, this will serve to confirm our suspicion.” Indeed, we cannot assign any sensory impressions to the notion of absolute space, so therefore the “term is employed without any meaning.”
Hume also explains why we should insist on this criterion of meaning. “If we carry our enquiry beyond the appearances of objects to the senses, I am afraid, that most of our conclusions will be full of scepticism and uncertainty.” Meanwhile, “As long as we confine our speculations to the appearances of objects to our senses, … we are safe from all difficulties, and can never be embarrass’d by any question.” In particular, we can never run into any self-contradictions stemming from “fictions of the mind.” To be “embarrass’d” is to have contradictions in your thinking exposed to you. But just stick to the senses and “what we have instruments and art to make” and you will be fine.
Actually I kind of hate David Hume. Hume is the Galileo of philosophy: an overrated false idol who erroneously gets credit for trivial ideas that had long been obvious to mathematically and scientifically competent people.
These quotes that I just read from Hume, they are fine philosophy, or rather, they were fine philosophy a hundred years before Hume, when the same ideas were advocated by better philosophers. I have argued that those notions were well known already to Greek mathematicians. Well, we can’t prove that, because we don’t have the source evidence to know for sure one way or the other what the Greek mathematicians thought about such things. But in any case, those ideas were obviously well understood by Descartes and Leibniz for example, which is why they insisted that all geometry must be constructive, and also why they insisted that only relative space makes any philosophical sense and absolute space is a cardinal sin that must be banished from the face of the earth.
When Descartes and Leibniz said these things, they were scientifically viable ideas. Descartes and Leibniz put their money where their mouth was. They backed up their philosophy with detailed, technical scientific works, that contained both technical progress on advanced mathematical problems as well as a programmatic vision of how scientific and mathematical practice can move forward in harmony with philosophical and epistemological principles.
After Newton, that dream is dead. Philosophy lost. And all scientifically competent people knew as much. So only the scientifically ignorant, such as David Hume, kept beating this dead horse. As one historian has put it, Hume was a “dour scientific dilettante” with “almost unparalleled ignorance of the science of his day.”
Indeed, in the 18th century, only people like that could still defend this old philosophy. People with scientific integrity knew that could not in good conscience advocate for such a philosophy anymore, because that would mean that they would have to give a philosophically coherent physics that worked as well as Newton’s absolute-space-based physics, which no one could do.
So the only people who could still repeat those old philosophies that were no longer scientifically credible were now the scientifically airheaded like Hume who wouldn’t know good mathematics if it hit them in the face. That was the sad state of this once proud philosophy in the 18th century.
No wonder this anti-Newtonian philosophy became a laughing stock for centuries. And then, plot twist. They were right! Einstein’s theory exactly vindicates what these people had been saying for more than two hundred years. If you try to do science with Newton’s Santa Claus concepts of space and time, then you are doomed to run into inconsistencies. Exactly as these guys had been warning. And the way out of these hopeless inconsistencies is: operationalise everything! Exactly what these philosophy nerds had been saying all along.
Unbelievable. Imagine insisting that the most successful scientific theory of all time, that has proved itself again and again for centuries, is bound to lead to inconsistencies and self-contradictions any day now. That must be one of dumbest predictions of all time, you would think. What stubborn and oblivious people would keep embarrassing themselves by saying such silly things? And then, those guys, those very archetypes of the utter irrelevance and pointlessness of philosophy—those guys of all people—hit the cleanest home run you will ever see, with Einstein’s relativity theory. Insane.
Let’s do a bit of relativity theory here to show this. We’re on a cruise ship now. Let’s go below deck. Here there is a tennis court. Oh boy, tennis is fun! Time flies though. While we’ve been playing, has the ship reached its destination and anchored in port? Or are we still moving?
**
You can’t tell. That is the principle of relativity. Everything in the tennis room will the same whether the ship is moving at a constant speed or standing still. Even though one of us may be playing against the direction of travel and the other with it, that doesn’t mean that our serves and smashes will be boosted one way and slowed down the other. If we hit equal serves at the same time, they will reach the net in the center of the court at exactly the same time.
That feels natural to us in the room because we are so absorbed in the game and we are not paying any attention to whether the ship is moving or not. We are using the room as our frame of reference, or coordinate system, so to speak. That is the center of our universe at the moment.
For example, let’s say we can hit tennis serves of 50 meters per second, and it’s about 12.5 meters to the net, so it will take a quarter second for the serve to get there. That’s the science of tennis that is relevant to us when we are absorbed in the game, not whatever the ship is doing.
But the same thing works also if seen from the outside. Some guy is standing on the shore, watching us go by. And he’s a science nerd, it turns out. He happens to have one of those speed cameras that the police use to catch cars going above the speed limit.
So, according to his measurements, the ship is going 10 meters per second, and he also measures the speed of our serves somehow. Those were all 50 meters per second according to ourselves, but that’s not what the readings will say on the speed camera of the guy on the shore.
The velocities will behave additively: the speed of a projectile = the speed at which the projecter is moving + the firing speed. So when I’m serving with the ship, in the direction of travel, that’s 10+50 meters per second. So 60 is the speed measured by the observer on the shore with his speed camera. And the serve going the other way will have velocity 10-50, so 40 meters per second in the opposite direction.
So that guy disagrees with us about the speeds, but he still agrees with us that the two tennis serves will reach the net at the same time. Because the one that’s faster has further to go, since the ship is moving while the serve is in the air. Remember, it took a quarter second for the serves to reach the net. So the ship will have moved 2.5 meters in that time. So the 60 meters per second serve will have 12.5+2.5 meters to go, that’s 15 meters. And the slower serve of 40 meters per second, it has the net coming toward it so it only has to go 12.5-2.5 meters, so 10 meters before reaching the net. Indeed, going 15 meters at a speed of 60 takes the same time as going 10 meters at a speed of 40.
So the fact that the motion of the ship is undetectable to us inside the room is basically equivalent to the principle of additivity of velocities as seen from some other fixed vantage point.
Ok, yeah yeah, boring old high school physics. Who cares? That’s all trivial, right? Not really. It’s not so trivial. In fact, it’s wrong and inconsistent with other parts of physics, as we shall see.
So-called trivial things can be quite profound. We remember this from Euclid, for example. Recall for instance the construction of a square in Proposition 46. At first sight you might go: “What a big commotion about nothing. I guess Euclid wrote for kids in middle school or something. Obviously a research mathematician does not need to have squares explained to them step by step. That’s silly and trivial.”
You might have thought that, but you would have been wrong. In fact, there are no squares in spherical or hyperbolic geometry, so carefully tracking the fundamental assumptions on which the existence of squares is based is deep theoretical question. Euclid knew that. He didn’t write for middle-schoolers. He wrote for highly sophisticated mathematicians who had thought a lot about the foundations of geometry.
It’s the same with the so-called trivial physics in our tennis room. Let’s see how we can re-analyse this “trivial” situation in operationalist terms. “Let two tennis balls be fired at the same time…” Oh no, you don’t. That’s like saying “let ABCD be a square.” We can’t have that. We have to operationalise it.
It was all very easy with Newton’s absolute time, or unicorn time if you like. If this one universal absolute time is given to you by Santa Claus, then the tennis players can just use that to coordinate their serves, no problem.
But if we don’t want to rely on Santa we have to coordinate the tennis serves ourselves. Ok, so I’ll just count it off, right? “1… 2… 3… go!” Then we both serve at the same time. No, not really, because I hear “go” the instant I say it but you have to wait for the sound to travel across the room before you hear it. So we’re not really synchronised that way after all.
And it’s the same with any light-based signal of coordination, like a green light switching on, or both of us looking at the clock on the scoreboard. The speed of light is not instantaneous either, so it matters whether the sources of the signal is closer to one of us than the other.
So it looks like we are going to have to make our definition of same time depend on distance. Indeed, Einstein does precisely this. I will quote to you from the book Relativity: The Special and General Theory, of 1916. This is Einstein’s popularised presentation of his theory. Einstein says:
“We … require a definition of simultaneity such that this definition supplies us with the method by means of which, … [we] can decide by experiment whether or not [two events] occurred simultaneously. As long as this requirement is not satisfied, I allow myself to be deceived …, when I imagine that I am able to attach a meaning to the statement of simultaneity.”
Right, indeed. We can’t just say: we both checked our iPhones and it said the same time, so it was simultaneous. Who gave you those iPhones anyway? Santa Claus again. We need a do-it-yourself option. And Einstein has one for you. Here is what he says:
“[We] offer the following suggestion with which to test simultaneity [of two events, one occurring at point A and one at point B]. … The connecting line AB should be measured up and an observer placed at the mid-point M of the distance AB. This observer should be supplied with an arrangement ([such as] two mirrors …) which allows him visually to observe both places A and B at the same time. If the observer perceives the two flashes of lightning at the same time, then they are simultaneous.”
So there you have an operational definition of what it means for two things to happen at the same time. It is interesting that this operationalisation of simultaneity involved finding the midpoint of a line segment. Einstein didn’t need to explain that any further since that part was itself operationalised by Euclid, if you recall. Proposition 10 of the Elements: to find the midpoint of a given line segment.
So Einstein’s definition of simultaneity, of what it means for two things to happen at the same time, is very much in the spirit of the operationalist tradition, and the kind of physics advocated by Descartes and Leibniz. Fundamental physical notions need to be formulated in terms that show how they are knowable. Such as simultaneity being knowable or accessible to observation by defining it in terms of looking at two events using mirrors and seeing if the two events coincide or not observationally.
If it’s just about tennis, then this doesn’t really matter. That’s indeed why this way of doing physics didn’t really go anywhere for two hundred years. People just used Newton’s Santa Claus time so they didn’t have to worry about any of that stuff with the mirrors and so on.
The philosophical subtleties about what simultaneous means only become relevant at speeds comparable to the speed of light. The speed of light does not behave additively. Unlike tennis balls. If I’m standing on a moving ship and fire a tennis ball, then I add speed to the ball in addition to the speed that it already had from moving along with the ship. But if I turn on a flashlight it will go at exactly the speed of light, a natural constant, regardless of however I was traveling. It’s not the speed at which it was already going plus the new speed. It’s always the same speed of light, regardless of whether it is going with or against whatever motion of some ship or whatever.
That light behaves this way seen experimentally, not long before Einstein’s theory. The constancy of the speed of light is also embedded in the theory of electromagnetism. Maxwell’s equations of electromagnetism from the mid-19th century were hugely successful and remain a cornerstone of physics today. As Maxwell said, “light is an electromagnetic disturbance, propagated in the same medium through which other electromagnetic actions are transmitted” (Treatise on Electricity and Magnetism, 786). So light is the same kind of thing as the WiFi signal for your phone and so on.
And that was a discovery and not an assumption. As Maxwell said: “I made out the equations in the country, before I had any suspicion of the nearness between the two values of the velocity of propagation of magnetic effects and that of light.” He did that “in the country”, that is to say, at the rural family estate in the Scottish countryside. So working in isolation, in other words, and only later being able to test the theory against lab data and such things. So the fact that light can be absorbed into the theory of electromagnetism was not an assumption built in to the theory but rather something that was independently confirmed later, when Maxwell returned from “the country.”
For our purposes the point is that the constancy of the speed of light was not just an isolated experimental fact: it was a result intertwined with core physical theory already before Einstein. Indeed the title of Einstein’s famous 1905 paper on special relativity is “On the Electrodynamics of Moving Bodies”, for precisely this reason.
So it’s not so strange to give the constancy of the speed of light a big role in operationalising time and space. This axiom that the speed of light is constant is indeed built into Einstein’s definition of simultaneity. It takes light equal time to travel equal distances. If the speed of a light ray depended on the speed of the object it was coming from, then Einstein’s definition of simultaneity wouldn’t make much sense. But because of the constancy of the speed of light we can count on equal distances in equal times.
Ok, but throwing this new ingredient into the mix seems to ruin everything we said before with the tennis stuff. We already saw that the principle of relativity—that we can’t tell in the tennis room whether we are moving or not—goes hand in hand with the principle of additivity of speeds, because it was the additivity principle that made the calculations come out equivalently for different observers. So, if light speed does not behave additively, that should mean that relativity will fall as well. We should be able to exploit the constancy of the speed of light to detect the motion of the ship from inside the tennis room. Precisely what was impossible using tennis balls should now be possible using light.
Namely: You and I stand at opposite ends of the tennis court and we each have a flashlight. We turn on our flashlights at the same time, and we see which light ray reaches the net in the middle of the court first. Since the speed of light is a universal constant regardless of whether it was fired with or against the ship’s motion, the two light rays will take a different amount of time to get to the net, since the net will will have travelled some millimeters along with the ship while the light rays are in the air.
So the principle of relativity is false: it is detectable by physical experiment from within a closed room whether the ship is moving or not. Right?
No! This is precisely an example of how the naive assumptions of Newtonian physics leads to errors and inconsistencies. We turn on our flashlights “at the same time,” I said. Here I was thinking like a Newtonian. The “same time,” according to the absolute time given to us by Santa Claus. But there is no such thing. You can’t just say: let these things be done at the same time.
It is precisely by trying to operationalise the concept of time that we see how naive this Newtonian Santa Claus perspective is. From the perspective of Newtonian absolute time, either the light rays were fired at the same time or not, and either they reach the net at the same time or not. Because of absolute time, those are straightforward raw facts as it were. And they are two independent facts: fired at the same time; reaches the goal at the same time. Two separately things. Hence it makes sense to test experimentally whether those two things are coordinated or not.
But when we operationalise we see how naive we have been. As we saw above, when we followed Einstein, the only way we could define the concept of two things happening “at the same time” operationally was to say: two events happened at the same time if the light signals from them coincide when they reach the midpoint between the two locations. So we cannot independently check whether two light rays fired at the same time reach the midpoint at the same time or not. If they reach reach the midpoint at the same time, then they were fired at the same time, by definition. If they don’t reach the midpoint at the same time, they were not fired at the same time, by definition. With the humble and honest operational notion of time, that’s all we can say.
So the principle of relativity remains valid after all. There is no experiment we can do in the tennis room that shows whether the ship is moving or not. As we see when we think operationally. Despite the fact that light speed does not behave additively, which we thought was equivalent to the principle of relativity before, when we were thinking classically, in terms of Newtonian absolute time.
So how does that work in terms of the outside observer then? The guy on the shore. When we compared these two classically it was the additivity of speeds that made everything work: the speed of the ship plus the speed of the tennis serve. But now we don’t have additivity anymore since we’re dealing with light. So what happens then?
From the perspective inside the room, we fired two light rays that hit the midpoint at the same time. We concluded that they were fired simultaneously. By definition: that was the only way we were able to define the concept of simultaneity operationally.
Now, the guy outside the ship, looking at the same thing, he’s going to come to a different conclusion. Because he will have a different opinion about what the midpoint is between the two firing positions. To us on the ship, the midpoint was the net in the middle of the tennis court. But the guy on the shore thinks the net is moving, so he doesn’t think it makes any sense to use that as a reference point. Instead he will put his finger at a point that is stationary with respect to the shore. This point will initially coincide with the position of the net but as the ship moves the net will move away from this fixed point.
Because of this, events that are simultaneous as seen from within the ship are not simultaneous as seen from outside the ship. With the net on the court as the reference midpoint, the light rays arrived at the same time, and hence were by definition fired simultaneously. That was the point of view of us on the ship. But if the light rays reach that point at exactly the same moment, then they cannot also reach the different reference point selected by the outside observer at the same moment also. So they must reach that guy’s reference point at different moments, and hence they must by definition have been fired at different times, not simultaneously, according to the outside observer’s point of view.
So, when time is defined operationally, time is different to different observers. There is no God-given absolute time that tells you whether two events really happened at the same time or not. One observer thinks one thing, another observer something else. And there is no way to decide who is “right.”
If you are reading along in Einstein’s book that I mentioned, what I have just described is his section “IX. The Relativity of Simultaneity.” After that comes section “X. On the Relativity of the Conception of Distance.” If two observers disagree about time they also disagree about distance, as we shall see. In order to be able to tackle such questions we first need to define distance operationally. Let’s see how Einstein does this.
In order to do this we have to switch our imagery. Instead of people playing tennis on a ship we are going to go with lightning hitting a train, which is Einstein’s example. The thing with people playing tennis inside a ship is a good picture in some respects. Better than the train. The idea that the motion of the ship is undetectable inside the room is quite intuitive in the case of a very large ship going at a perfectly steady speed. More so than the same thing for a train, I think. Also it was nice that a tennis court has its midpoint conveniently landmarked with a net. That helped us as well to make the prominent role of the midpoint in Einstein’s definition of simultaneity more vivid in our minds, I think.
But actually this image started to break down a bit when we tried to explain the simultaneity experiment from the point of view of the guy on the shore. He was supposed to select a midpoint between the two tennis players that was stationary with respect to the shore. Which is not very practical at all. How would you hold such a point fixed, and keep your mirrors there? And besides, how does the guy on the shore determine the midpoint anyway? I mentioned that you can find the midpoint in the manner of Euclid, for instance. Fine, that works for the observer on the ship. But not the guy on the shore. He has to measure two moving targets at the same time, and somehow fix their midpoint in space at some instant, and he doesn’t even know yet what the same instant for each point even means because that is what the experiment with the mirrors placed at the midpoint is supposed to reveal.
So the ship thing doesn’t really work from a practical point of view. But we can fix these problems by switching to the train scenario instead. Alright, a train is travelling at a constant speed. It is struck by lightning at two places. Did the two lightning strikes occur at the same time or not? Well, we know how to check that. They occurred at the same time if the light from both of them reaches the midpoint at the same time. Of course, in order to check this you would have had to have your double mirrors already set up at the midpoint to begin with, as the lightnings struck. So you had to know in advance where the lightning was going to strike. But maybe that’s doable. Let’s say that the train has two lighting rods, so lightning will only strike where the lighting rods are.
In fact, we don’t really need a train as such. We can take away the walls and the roof of the train. The train is just a moving floor and it has one lightning rod at either end, and it has a pair of mirrors set up exactly halfway between them where we can see both lightning rods at the same time and judge whether the light signals from both coincide in time or not.
It doesn’t have to be lightning either. It can be a man-made thing like a light bulb, or an explosion going off. But lightning is a pretty good image in some ways. Because it conveys the idea that it is the light from the event that is the important thing, and it also conveys that the event happens at one particular instance. It is not an ongoing thing, like a light bulb staying on. It’s bam, split second, done.
And here’s another great thing about lightning that will help us a lot. It leaves a mark, a kind of burn mark, an imprint, where it struck. We don’t need that on the train, because we already have the lightning rods marking those positions. But what about the positions of the lighting strikes as seen from a stationary outsider? This gave us quite a bit of trouble with our ship example. The guy on the shore was supposed to reference things to a stationary reference frame from his point of view, but it was hard to make that concrete in that case: how do you freeze certain positions on a moving tennis court in space? You can’t. So we could only imagine that theoretically.
Now with the lightning marks this is going to work better. The train is passing a stationary train platform. A train ”station” indeed: it is stationary. When lightning strikes, it leaves a burn mark with a certain impact radius, so it also burns the platform right next to the lightning rod at that instant. Perfect! Now we have the positions where the events took place burn-marked into the stationary platform itself. So now there is no problem anymore to talk about the midpoint of the two events with respect to the stationary frame of reference. It is just the midpoint between the two burn marks. We don’t have to chase any moving targets to determine that.
So, as we said, the two observers will disagree about whether the lightning strikes were simultaneous or not. We can now quote Einstein’s explanation of this, which is the same as what I already said about the ship, but expressed in terms of the train and lightning.
“When we say that the lightning strikes A and B are simultaneous with respect to the platform, we mean: the rays of light emitted at the places A and B, where the lightning occurs, meet each other at the mid-point M of the length AB of the platform. But the events A and B also correspond to positions A and B on the train. Let M’ be the mid-point of the distance AB on the travelling train. When the flashes of lightning occur, this point M’ naturally coincides with the point M but it moves … with the velocity v of the train. If an observer sitting in the position M’ in the train did not possess this velocity, then he would remain permanently at M, and the light rays emitted by the flashes of lightning A and B would reach him simultaneously, that is, they would meet just where he is situated. Now in reality (considered with reference to the railway platform) [this observer on the train] is hastening towards the beam of light coming from B, whilst he is riding on ahead of the beam of light coming from A. Hence the observer will see the beam of light emitted from B earlier than he will see that emitted from A. Observers who take the railway train as their reference-body must therefore come to the conclusion that the lightning flash B took place earlier than the lightning flash A. We thus arrive at the important result: Events which are simultaneous with reference to the platform are not simultaneous with respect to the train, and vice versa (relativity of simultaneity). Every reference-body (co-ordinate system) has its own particular time; unless we are told the reference-body to which the statement of time refers, there is no meaning in a statement of the time of an event.”
Right, so that’s the relativity of simultaneity, or relativity of time. Relativity is a consequence of operationalisation. And the relativity of time is in turn going to imply the relativity of space, or relativity of length, as we said. Let’s now look at that.
Let’s quote Einstein’s words here about how to compare distances across the two different reference frames. First Einstein gives a very operational definition of how distance is defined within a given reference frame, for example within the train.
“Let us consider two particular points on the train travelling along the platform with the velocity v, and inquire as to their distance apart. … An observer in the train measures the interval by marking off a measuring-rod in a straight line (… along the floor …) as many times as is necessary to take him from the one marked point to the other. Then the number which tells us how often the rod has to be laid down is the required distance.”
Great to see Einstein crawling around on all fours, laying down measuring rods. Remember Euclid in Rafael’s fresco of the School of Athens? Hunched-over with his geometry tools. We have gone full circle with Einstein. Not only does he have the same hairstyle as Rafael’s Euclid, he also has the same philosophy. If you want to understand the geometry of space you have to get operationalising, tools to the ground.
So that’s how you define distance, or operationalise distance. How many sticks long is it? How many times do I have to put a stick down to cover the whole thing, to get from one end to the other? That number is the length. And half of that number is the midpoint.
Now it gets trickier if the guy on the platform wants to measure this length. How are you supposed to do this thing with the sticks if everything is moving? One stick, two sticks, … Oh crap, the train is already long gone. That didn’t work.
The solution is to transfer out an imprint of the train, a kind of freeze-frame version of it imprinted on the platform, which we can then measure in peace and quiet after the train has left.
Kind of like the lightning marks, except the marks have to be made at the same time, and that’s precisely where it gets complicated. The same time according to whom? Were the lightning strikes simultaneous or not? Different observers disagreed about that. So when we say “make two marks on the platform corresponding to the endpoints of the train” we have to be careful about how we time the two marking events so that they are simultaneous.
Here is how Einstein puts it:
“The following method suggests itself. If we call A’ and B’ the two points on the train whose distance apart is required, then both of these points are moving with the velocity v along the platform. In the first place we require to determine the points A and B of the platform which are just being passed by the two points A’ and B’ at a particular time t, judged from the platform. These points A and B of the platform can be determined by applying the definition of time given [above]. The distance between these points A and B is then measured by repeated application of the measuring-rod along the platform. It is by no means certain that this last measurement will supply us with the same result as the [measurement performed by the observer on the train]. Thus the length of the train as measured from the platform may be different from that obtained by measuring in the train itself.”
We can picture it like this. At each end of the train there’s a guy with a paint brush. As the train passes the platform, the two guys each draw a mark on the platform at the same time. Then the length between the marks is the length of the train, surely.
But now we used the idea of “at the same time” again. So if the painters on the train draw the marks “at the same time” according to themselves, then the observer standing on the platform will go: “Noo! What are you doing?! You mistimed it! The guy at the front of the train drew his mark later than the guy at the back of the train.”
This is the direction in which the two observers disagree about simultaneity, as we saw already in the lightning examples. If the light rays from the two events to reach the midpoint of the train at the same time, then, at that moment, the guy standing at the midpoint between the two marks on the platform will already have seen the signal from the event at the back of the train, but not yet the signal from the front of the train. So simultaneous according to “train time” means that the event at the front of the train was delayed, according to “platform time.”
And the faster the train is going, the greater the delay will be. So the distance between the two painted marks will be greater for a faster train. I mean the distance between the marks drawn simultaneously according to train time. So in other words, the length of the train takes up a bigger portion of the platform. If the train covered 50% the platform when it was at rest, it will cover 80% of the platform when swishing by at a large constant speed. According to the observer on the train, that is, because we made the marks according to his simultaneity.
So “the train has grown,” you might say. Or rather, the platform has shrunk, of course. That’s how the guy in the train will interpret it. In his view, the train is the thing that remains fixed. To him the train of course has the same length all the time. For example, it takes him the same amount of effort to walk from one end of the train to the other. And it is as many sticks long, the same stick he used to measure it when the train was parked.
So when he’s swishing by the platform and makes his “simultaneous” paint marks corresponding to the length of the train, and the marks take up a greater part of the platform than before, he will say: “Huh, I guess the platform has shrunk since last time I was here.”
That is the famous length contraction phenomenon. Things that move shrink. Or rather: things that move relative to an observer appear to shrink according to that observer. The train guy thinks the platform shrunk, as we saw.
And the platform guy thinks the train shrunk, which we didn’t see directly the way we described the experiment but it must be that way because the roles of the platform and the train are interchangeable. The guy on the train can say: “I’m not moving. You’re moving!” And no physical experiment can prove him wrong. That is an axiom of relativity theory. So therefore if one guy thinks the other one shrunk, then the second one must think the first one shrunk. Because there can’t be any asymmetry, as long as they are moving at constant relative speed.
But ok, enough physics. We’re not going to become a physics podcast. My point was not to do an intro lecture on special relativity. My point was to emphasise how operational that theory is.
Time is something you do. Just as a triangle is something you do. I put a ruler on a piece of paper, I draw three intersecting lines, bam bam bam, that’s a triangle. “Oh no, that can’t be a triangle because it’s not perfect, actually. A perfect triangle must be a Platonic blah blah blah.” No. You haven’t learned the lesson of relativity theory.
Einstein didn’t use operational definitions of time and distance because he wanted his theory to be applicable in practice. He defined everything in terms of doing because the more theoretical alternative was philosophically naive and untenable.
For the same reason the Greeks reasoned in terms of constructions. Not because they were practically oriented and childlike and pre-theoretical, but precisely because they were more theoretically sophisticated, not less. Just as Einstein’s mirrors and sticks are more foundationally sophisticated than the precious abstract theory of Newton.
 23 July 2023, 9:12 am
23 July 2023, 9:12 am - 52 minutes 4 secondsReview of Netz’s New History of Greek Mathematics
Reviel Netz’s New History of Greek Mathematics contains a number of factual errors, both mathematical and historical. Netz is dismissive of traditional scholarship in the field, but in some ways represents a step backwards with respect to that tradition. I argue against Netz’s dismissal of many anecdotal historical testimonies as fabrications, and his “ludic proof” theory.
Transcript
A new book just appeared: A New History of Greek Mathematics, by Stanford Professor Reviel Netz, Cambridge University Press. Let’s do a book review.
It will be a critical review. The main theme will be the sciences versus the humanities. Note the title of the book: “a New History.” Netz’s “New History” represents the new humanities-centred dominance in the field. As opposed to the “old” histories written by more mathematically oriented people. In my opinion, “new” does not mean better in this case. And I will tell you why.
Let’s start by attacking a city. The enemy are hunkering down behind their city walls. We are going to have to scale the walls with ladders. How long should we make the ladders? The ancient historian Polybius has the answer:
“The method of discovering right length for ladders is as follows. … If the height of the wall be, let us say, ten of a given measure, the length of the ladders must be a good twelve. The distance from the wall at which the ladder is planted must, in order to suit the convenience of those mounting, be half the length of the ladder, for if they are placed farther off they are apt to break when crowded and if set up nearer to the perpendicular are very insecure for the scalers. … So here again it is evident that those who aim at success in military plans and surprises of towns must have studied geometry.”
Great stuff. But Netz gets it wrong, in my opinion. Here is how he concludes:
“And then, of course, we are supposed to apply – Polybius leaves this implicit – Pythagoras’s theorem.” (223)
I don’t think so. I don’t think that’s what Polybius intended.
Sure enough, you can solve for the length of the ladder using the Pythagorean Theorem, but that is a clumsy and inefficient way to do it. If you did this the modern way you would need to do some algebra followed by some calculation involving a square root. They didn’t have calculators on their phones back then, you know. Do you expect carpenters in the military to be able to calculate square roots by hand?
In fact, Polybius has already told you everything you need to know with his numerical example. If the wall is 10, the ladder should be 12, he says. But it scales! So what Polybius is really saying is that, whatever the height of the wall is, the ladder is always 20% longer than that. That’s all you need to know. No Pythagorean Theorem needed.
Those numbers are a rule of thumb. You can also do it more exactly if you want, according to Polybius’s more theoretical characterisation of the optimal length. But you don’t need the Pythagorean Theorem for that either. There’s a much better way, that you can easily teach to an illiterate carpenter in five minutes.
Draw an equilateral triangle, just as Euclid does in Proposition 1 of the Elements. Cut it down the middle. Now you have a right-angled triangle, where the base is exactly half of the hypothenuse. This corresponds precisely to Polybius’s rule: the distance along the ground is half the length of the ladder.
So now we have a scale model of what we want. The height down the middle of the equilateral triangle represents the city wall; the side of the equilateral triangle represents the ladder, and it is precisely half its own length from the foot of the wall, exactly as Polybius says it should be for optimal stability.
So if we are given that the height of the wall is for example 10 meters, then we divide the height of the triangle into ten equal parts. We take a blank ruler and mark those ten marks on it. Then we take this ruler, with this length unit, and measure the hypothenuse of the triangle. However many marks long it is, that’s how many meters our ladder needs to be.
Piece of cake. Easy to improvise in the field without any specialised knowledge or tools. While Netz is busy trying to teach his carpenters the algebra of quadratic expressions and how to extract square roots, I have already scaled his walls using my much quicker methods. That is what you get when you put humanities people in charge of mathematics.
So I wouldn’t trust Netz when it comes to mathematics, even when he says “of course,” as he does here.
Here is another example: Did you know that parabolas are pointier than hyperbolas? At least if we are to believe Professor Netz. This claim occurs in a discussion of Archimedes. Archimedes studied solids of revolution obtained by rotating a conic section around its axis. Here are Netz’s words:
“In the case of a parabola, this will be of a more pointed shape; in the case of the hyperbola, this may be more bowl-like.” (140)
This is BS. Parabolas are not “more pointed” than hyperbolas.
This is clear for example from the following fact: you can draw a hyperbola having any two given lines as asymptotes and passing through any given point. So in other words, you can draw a V, an arbitrarily pointy letter V, and then pick an arbitrary point inside that V, for instance a point super close to the vertex of the V. Then there is always a hyperbola that fits inside the V and that passes through the designated point. You can hardly get any pointier than that, now can you? Yet parabolas are nevertheless “more pointed”, somehow, Netz apparently believes.
By the way, this fact I just mentioned, about constructing a hyperbola within a given V (that is to say, with given asymptotes), that is Proposition 4 of Book II of the Conics of Apollonius.
Or is it? Here we have another interesting point. It seems that this proposition was actually not in the original version of the Conics. Because Eutocius, in late antiquity, needs this theorem at a certain point and he says he better prove it since it’s not in the Conics of Apollonius. But then in the text we have of the Conics, what we call Apollonius’s Conics today, this proposition clearly is there, with the exact same proof.
And in fact the standard text that we call Apollonius’s Conics today comes to us only through that very same author, Eutocius, who wrote a commentary on the Conics and also preserved the text at the same time. So it seems that Eutocius inserted this proposition into Apollonius’s original text, because he had noticed in other works that it was a useful thing to prove.
Netz describes this correctly, which is all the more reason why he should know that a hyperbola can be as pointy as you’d like, since this follows immediately from this proposition that he discusses at length.
But anyway, there is another kind of error here in Netz’s discussion of this. The point that this proposition of the Conics is an insertion by Eutocius — that insight, says Netz, is due to Wilbur Knorr, Netz’s predecessor as a classics professor at Stanford.
“No one noticed that prior to Knorr” (431-432), says Netz.
But that is not true. Wilbur Knorr was not the first to discover this. In fact, Knorr clearly says so in his own article, the very article cited by Netz, which Netz has evidently not read very carefully. Already in the 16th century, Commandino, in his Latin edition of the Conics, very clearly and explicitly made the exact same point as Knorr, using the exact same evidence and arguments. And this in turn was cited in a 19th-century German edition of the Conics, as Knorr himself says. So Knorr didn’t discovery anything except what people had already known for hundreds of years.
This is not such an innocent mistake. How are we supped to trust anything Netz says if he makes blatantly false statements that are clearly and unequivocally seen to be factually incorrect by simply glancing at the very article that Netz himself cites in support of his own claims?
But it’s even more problematic than that. Because it’s clearly not just a random mistake. It is an ideologically driven error. By saying that Stanford humanities professor Wilbur Knorr was the first to make this important scholarly discovery, Netz is obviously indirectly boosting the impression that his own claims are important and novel, since he too is a Stanford humanities professor.
Netz is not only saying that Wilbur Knorr was the first to discover this particular thing. He is implicitly saying that earlier generations of scholars missed important insights, and that only people like him — Stanford humanities professors — are true experts.
That is of course the point of the title of the book: A *New* History of Greek Mathematics. In the past everybody did it wrong, and we need people like Netz to finally do it right. There is indeed a lot of explicit posturing to this effect throughout the book.
Let’s look at another example of this. Let me read a passage where Netz is attacking Thomas Kuhn’s account of the history of astronomy. Thomas Kuhn wrote in the mid-20th century and he worked on the history of science even though his PhD was in physics. So that is exactly the kind of people Netz wants to denigrate. He wants to say that only specialised humanities professors, with their “new” histories, are actual experts in the field.
Here is what Netz says about Kuhn: “Like most nonspecialists, Kuhn supposed …” See? I told you. It’s not just that Kuhn was wrong. It is that Kuhn epitomises the kind of people (people with a PhD in physics, for example) who need to be eliminated from the field because they make so many hopelessly naive assumptions without even realising it. Anyway, let’s continue with the quote:
“Like most nonspecialists, Kuhn supposed that Aristotle was broadly canonical from the beginning and that although the ancients offered various astronomical variations, these had all to agree with the Aristotelian framework. … This is wrong. In fact, Aristotle was not canonized throughout most of antiquity; Greek philosophers were in continuous, ever-shifting debate; the very practices of astronomy went through several stages in antiquity before they became stabilized through the ultimate canonization of Ptolemy – and of Aristotle – in Late Antiquity.” (487)
Indeed, I agree with Netz that mathematicians and scientists would have ignored Aristotle. Netz says it very well:
“In the second century BCE itself, Aristotle was marginal even within philosophy, let alone to a scientist such as Hipparchus. It is quite likely that Hipparchus never even read Aristotle’s Physics.” (346) Reassessing ancient science in this light, “we come close to imagining a very Galilean Hipparchus” (347).
Yes, perfect, I agree. That is exactly what I have said before about ancient science as well. Go Team Netz on that one.
But what about poor Kuhn whom Netz uses as a punching bag? Was he really so stupid? No.
I went to my copy of Kuhn’s book on the Copernican Revolution to check Netz’s accusations, and here is what I found. Here is a quote from Kuhn’s book:
“The great Greek philosopher and scientist, Aristotle, whose immensely influential opinions *later* provided the starting point for most medieval and much Renaissance cosmological thought.” (Kuhn, Copernican Revolution, 78)
So Kuhn says exactly the opposite of what Netz accuses him of “supposing”. *Later* Aristotle provided the starting point of scientific thought. Not “from the beginning.” Later. Exactly as Netz himself argues.
Here is another quote from Kuhn’s book that says the same thing:
“Aristotle said a great many things which later philosophers and scientists did not have the least difficulty in rejecting. In the ancient world there were other schools of scientific and cosmological thought, apparently little influenced by Aristotelian opinion. Even in the late centuries of the Middle Ages, when Aristotle did become the dominant authority on scientific matters, learned men did not hesitate to make drastic changes in many isolated portions of his doctrine.” (Kuhn, Copernican Revolution, 83)
There is no way you can read this and say that “Kuhn supposed that Aristotle was broadly canonical from the beginning,” that is to say, from his own lifetime onwards. Kuhn clearly says the opposite.
Netz’s accusation is just slander. So it’s the same in both the Knorr case and the Kuhn case: Netz makes false assertions and then cites sources that clearly and explicitly say the exact opposite of what Netz alleges.
At least in these cases Netz bothered to provide references at all. More often he doesn’t even do that. He allows himself the licence to make assertions at will, which readers are supposed to accept on his authority alone. Consider for example the following rant about the alleged bias of some unnamed “past scholarship”:
“In past scholarship, this Babylonian achievement [in astronomy] was sometimes dismissed as ‘merely’ practical, the Babylonians unfavorably compared with the Greeks in that they did not produce a geometrical account of the sky, hence no physical model, so, unlike the Greeks, ‘not real science’. This is obviously an absurd special pleading, where one defines as scientific whatever it is that the Greeks do and then reprimands the non-Greeks for failing to be Greek. The Babylonian theory is in fact directly analogous to the Greek mathematical theory of music – whose scientific significance no one doubts.” (326)
Well, no wonder that we need a “new” history of Greek mathematics then, amirite? That darn “past scholarship,” you know, they couldn’t think straight back then because they were so biased in favour of the Greeks. Or why sugarcoat it, why not just come out and say it: They were all racist back then, weren’t they? Thank God we have proper humanities-trained experts like Netz at last to save us from all of that. “A New History of Greek Mathematics”. Basically code for: The First non-Racist History of Greek Mathematics.
Well, yes, the argument that Netz refutes is indeed idiotic. But what is this so-called “past scholarship” that allegedly made this idiotic and basically racist assertion that Babylonian astronomy is “not real science” because it’s not geometrical? Who ever said that? No one I ever heard of.
Maybe Thomas Heath? If Netz is the “new” history of Greek mathematics, then Heath’s famous book is obviously the old one, written more than a hundred years ago.
But no. I looked it up. Even old Heath explicitly uses the phrase “Babylonian science” with approval (History I, 8). Of course it was “science”. Perhaps Thales, in his travels, learned of “Babylonian science”, for example, Heath says (Aristarchus of Samos, 18), in exactly those words.
So who, then, is Netz arguing against, except straw men that he has made up to present himself as the anti-racist saviour? I don’t know.
But enough bickering about that. Let’s turn to a big issue of major interpretative importance.
According to Netz, “Thales and Pythagoras did no mathematics whatsoever” (17). According to Netz, earlier generations of scholars naively believed in such fairy tales because they blindly trusted a single source:
“My predecessor Heath and many historians – up until the last generation – gave credence to the view according to which Thales, and then Pythagoras, made lasting contributions to mathematics. This derives almost entirely from Proclus’s commentary, which, because of its overall sobriety, was taken seriously even for such obviously unfounded assertions.” (423)
First of all, it is not true that this “derives almost entirely from Proclus’s commentary.” It is disturbing that Netz makes this false and self-serving statement. Just read Heath, whom Netz names in this very rant. Read Heath’s chapter on Thales. Heath goes through the sources explicitly. There are several sources about Thales as a mathematician that predate Proclus. And several of those testimonies, as well as passages in Proclus, are explicitly attributed to various specific earlier authors.
So it is not the case that earlier generations of scholars uncritically and blindly relied “almost entirely” on a single biased source, as Netz dishonestly and falsely claims.
Let’s look at Thales and Pythagoras in turn. Let’s start with Thales.
I have spoken before about how the idea of Thales as the originator of formal geometry makes good sense. The way I told it was based on two theorems attributed to Thales.
The first theorem is that a diameter cuts a circle in half. I described how one can show that using a very neat proof by contradiction. The appeal, obviously, would not have been the theorem as such, but the realisation that that kind of thing can be established by a very elegant and satisfying type of reasoning, namely a rigorous argument based on paying careful attention to the definitions of concepts such as circle and diameter, and the remarkable power of proofs by contradiction for proving this kind of thing. That is exactly the same aesthetic that one finds on the first pages of almost any modern mathematics textbook in abstract algebra, for example: proofs of basic results driven by carefully formulated definitions and tidy proofs by contradiction. It makes sense that people would fall in love with this aesthetic that has stood the test of time, and it makes sense that it would have begun with a basic theorem such as that the diameter bisects a circle. Just as ancient sources suggest.
A second theorem attributed to Thales is that a triangle inscribed in a circle with the diameter as one of its sides must be a right triangle. It is natural to arrive at this insight by playing around with ruler and compass. And the aha-moment would then have been that one can prove such things. Make a rectangle, draw a diagonal, draw the circumscribing circle. Now you are in business. From playing with shapes, you have arrived at a proof of a universal truth. Pretty cool. It makes sense that the idea of proving geometrical theorems might have started with something like that, as some ancient sources suggest.
I told my own version of this story, but in broad outline something like that is a pretty standard and well-known point of view. But Netz acts as if he has never heard of any of that. He pretends that people who believe that Thales initiated geometry are simply blindly taking Proclus’s word for it without having thought it through at all. Netz says so explicitly. Here are his words:
“I suggest here that Hippocrates’s works were among the earliest pieces of Greek mathematics ever to be written.” (48)
Ok, so that’s Hippocrates, considerably later than Thales, famous for a very technical and detailed argument about the areas of lunes, a kind of shape composed from circles. This looks a lot more like a specialised piece of technical geometry from a quite mature geometrical tradition. It seems like a very odd and obscure place to start with geometry altogether. In reply to this, Netz says:
“This might seem surprising. Could mathematics emerge like that – springing forth from Zeus’s head? Would we not expect mathematics to emerge in a more rudimentary form? In fact, I think this is precisely how we should expect mathematics to emerge: from Zeus’s head, fully armed. What would be the alternative? … Of course the very first mathematical works in circulation would contain remarkable, surprising results. Why else would you even bother to circulate them? I suspect that the counterfactual is sometimes not sufficiently carefully thought through here. Just what would a more rudimentary piece of mathematics look like? Would it prove some truly elementary results, such as, say, the equality of the angles at the base of an isosceles triangle? Why would anyone care about such a treatise, proving such a result?” (48)
It is baffling that Netz allows himself to make this lazy argument, as if no one had ever though those things through. He states these rhetorical questions as if no one had ever thought of any of that. But of course people have thought about that and they have compelling answers to Netz’s questions.
I just told you what the alternative to Netz’s narrative is and why it would make sense. And I am not the first person to say this. But Netz is too lazy to engage with alternative views seriously, so instead he dishonestly says that no one has ever thought through any alternative to his view.
So that’s Thales. Netz rejects a plausible interpretation of the Thales testimonies in ancient sources by dishonestly mischaracterising as hopelessly naive any scholars who adhere to such views.
Now Pythagoras. “Heath … had three full chapters on the mathematics of Thales and Pythagoras!” (22), Netz says triumphantly, suggesting that this is proof that his “new” history of Greek mathematics is sorely needed.
Anyone who believes in Pythagorean mathematics is stupid, according to Netz, and for this he relies on a famous book by Burkert. Here is how Netz describes it:
“[Burkert’s book] Lore and Science in Early Pythagoreanism … was a more careful, professionalized classical philology, keen to understand the authors we read not as mere parrots, repeating their sources, but instead as thoughtful agents who shape and retell the evidence as suits their agenda. Pythagoras, under such a reading, crumbles to the ground: almost everything … comes to be seen as the making of later authors from Aristotle on. Never mind: the historians of mathematics went on as before.” (23)
We hear the ideological overtones here. Burkert is Netz’s kind people: he is hailed as “professionalized.” By contrast, “the historians of mathematics went on as before”. That is to say, the mathematically trained people working on history of mathematics were a bunch of fools who didn’t even realise what fools they were, and we would be much better off if “professionalized” experts such as Burkert and, presumably, Netz himself, would be given a monopoly on expertise status in the field.
I do not agree with this, neither in terms of content nor ideology.
Regarding Pythagorean mathematics, since Netz doesn’t go into any more depth, I will now analyse Burkert’s book itself, which Netz accepts as gospel truth. A book review within a book review! Here we go.
According to Burkert, “the apparently ancient reports of the importance of Pythagoras and his pupils in laying the foundations of mathematics crumble on touch”. Not that phrase: “the foundations of mathematics.” I am going to criticise Burkert, and I am going to say that Burkert makes a naive and anachronistic assumption about what “the foundations of mathematics” are. (For page references for the quotes from Burkert, see my Operationalism article.)
When Burkert speaks of “the foundations of mathematics,” he takes for granted the traditional view that a core pillar of Greek geometry was its Platonist detachment from the physical world. As Burkert says, “Greek geometry assumed its final form in the context of [Plato’s] Academy … after Plato had … fixed its position as a discipline of pure thought.”
Indeed, Burkert’s arguments against Pythagoras’s mathematical significance are really arguments that he did not advocate a proto-Platonist philosophy of mathematics. Burkert’s overall thesis is that “that which was later regarded as the philosophy of Pythagoras had its roots in the school of Plato.” And indeed he proves convincingly that there was a clear tendency to distort history in this way in Platonic sources that is not consistent with more reliable sources outside this tradition.
For example, Burkert shows that when Proclus mentions Pythagoras in his “catalogue of geometers,” and attributes to him “a nonmaterialistic procedure” in mathematics, this, unlike the rest of the catalogue of geometers, is not based on the highly credible Eudemus. Instead it is copied from Iamblichus, that is to say, from the biased Platonic tradition.
From this it does not follow, as Burkert tries to argue, that Eudemus did not mention Pythagoras as a geometer at all. It follows only that Eudemus in this place likely did not associate Pythagoras with proto-Platonic views. This is enough to give Proclus the motivation to supplement his account with phrases from Iamblichus, even if Eudemus had mentioned Pythagoras in the original.
Burkert also observes that “Aristotle [says] expressly of the Pythagoreans [that] ‘they apply their propositions to bodies’---bringing out the distinction, in this regard, between them and all genuine Platonists.” Eudemus and Aristotle are clearly much more credible than the much later, more biased, and less intellectually accomplished Iamblichus and Proclus.
Thus Burkert’s arguments that Pythagoras’s alleged proto-Platonist philosophy of geometry is a fabrication of biased sources are quite convincing. However, it does not follow from this that the Pythagoreans did not take a profound theoretical and foundational interest in geometry altogether.
Burkert conflates these two conclusions, because he sees no alternative path to theoretical mathematics than through Platonic-style abstraction and detachment from physical considerations. Burkert believes that early work on geometrical constructions “is still not doing mathematics for its own sake”; rather, the “discovery of pure theory” was a later “accomplishment,” in his words.
If you have followed what I have said in the past you know that I reject this. Burkert is naive to assume a dichotomy between constructions and “pure theory.” Constructions were not the opposite of theory, and hence the opposite of “the foundations of mathematics,” as Burkert erroneously assumes. On the contrary, constructivism *was* the foundations of mathematics.
Once we admit that possibility, there is every reason to think that earlier mathematicians, such as the Pythagoreans, could very well have made profound and foundationally sophisticated contributions, while at the same time rejecting Platonising tendencies in the philosophy of geometry.
Indeed, when going beyond his convincing case against Pythagoras the Platonist, to the more general case of trying to minimise the significance of Pythagoras and his followers in the history of geometry, Burkert find himself on the back foot. He is forced to try to explain away Aristotle’s compelling statement that “the so-called Pythagoreans were the first to take up mathematics; they advanced this study, and having been brought up in it they thought its principles were the principles of all things.”
Burkert’s thesis leaves him little choice but to dismiss the centrality of mathematics implied by this statement as “a psychological conjecture of Aristotle, which the historian is not obliged to accept.” That Proclus was wrong is plausible enough, but having to postulate that Aristotle was wrong comes at a considerably higher cost. And while Burkert was able to discredit Proclus’s mention of Pythagoras in the catalogue of geometers, he cannot deny that numerous attributions of mathematical discoveries to Pythagoreans made by Proclus are indeed based on Eudemus and hence credible, by Burkert’s own admission. Thus even Burkert must admit that “Pythagoreans made significant contributions to the development of Greek geometry.” Yet he hastens to add: “but the thesis of the Pythagorean foundation of Greek geometry cannot stand.”
Once again Burkert’s argument is based on tacitly assuming a monolithic conception of what “the foundations of Greek geometry” consisted in. The constructivist reading of Greek geometry problematises this assumption. It shows that one cannot simply take for granted that “the foundations of geometry” means what modern authors think it should mean. Constructivism offers an alternative vision, according to which much early Greek geometry may very well have been eminently foundational, but in a sense different from that commonly assumed by modern observers. This at the very least raises the possibility that early traditions such as that of the Pythagoreans may have been more foundationally significant than Burkert’s argument admits.
So much for Burkert, whose judgement Netz accepts unconditionally. Far from being an unequivocal triumph of “professionalized” expertise over previous naiveté, as Netz would have it, Burkert’s account is itself naive and by no means unquestionable.
So Netz is fond of dismissing what the ancient sources say. All the stories about Thales and Pythagoras, that’s just so much fiction. To be sure, the sources are highly imperfect and definitely contain a lot of misinformation. Nevertheless, it is surely better to try to save some meaning in these stories than to almost take it as a point of pride to dismiss as much of it as possible, as if the more sources you dismiss the more sophisticated a historian you are.
In fact, Netz continues in the same vein for later Greek geometry as well. “The stories [about Archimedes] probably are fabricated,” (128) we are told.
Stories such as Archimedes’s use of the principles of hydrostatics to detect a fake gold crown, because it did not have the right density properties. That is the “Eureka!” story.
“Biographers concoct anecdotes, based on the contents of the authors’ works. This is clearly the case here. The story of the crown is a clear echo of Archimedes’s study of solids immersed in liquids, On Floating Bodies.” (129)
Now, how would this work exactly? Let us “think through the counterfactual,” as Netz admonished others to do above.
Ok, so Archimedes wrote a sophisticated technical work on floating bodies. For some reason. Certainly not because of fake gold crowns and such things, because those are just “concocted anecdotes.” I guess Archimedes just woke up one day as said to himself: I think I will prove a bunch of theorems about hydrostatics, which nobody has done before, because I’m a mathematician and I just do things arbitrarily for no reason with no connection to the real world.
So he wrote a detailed, hyper-mathematical treatise on floating bodies. Theorem-proof, theorem-proof.
And then, maybe hundreds of years later or whatever, another guy told himself: Hey hey, I’m a writer! I’m going to write about the history of mathematics, but I won’t find out actual facts about the history of mathematics. Instead I’m going to pour over these extremely technical treatises that very few people can understand, and I’m going to master their content in great depth, to the point where I will be able to invent out of thin air real-world scenarios that involve realistic, sophisticated applications of the complicated technical results found in these treatises. And my goal in doing so is to concoct a one-paragraph anecdote about for example Archimedes making a discovery in the bath that made him run naked through the streets. Haha, what a funny image to imagine him running and screaming eureka like that. Totally worth all those probably hundreds of hours that I had to spend studying very complicated mathematics and then designing and working out my own research-level applied mathematics problem just so that I could make this little joke about Archimedes running from the bath.
Well, that’s apparently what happened if we are to believe Netz.
I very much doubt that story tellers were ever that good. The story about Archimedes and the crown is really very good scientifically. The connection with the technical details of Archiemdes’s treatise is the real deal. If this is a “fabricated” anecdote “concocted” by a biographer, as Netz says, then that biographer was not only a story teller but one of the leading scientists of their age.
Look, I teach calculus regularly, and I always try to get students to think about the physical meaning of mathematical notions and interpret results in the context of a real-world scenario. And I can tell you that that is an uphill battle to say the least.
I don’t think Netz teaches calculus so I think he underestimates how hard it is to make up stories that simultaneously make perfect scientific sense.
It is quite easy, on the other hand, to make up stories that do *not* make scientific sense. And that bring us to another one of Netz’s theories.
Netz has another book called “Ludic Proof”. Ludic as in play, playfulness. According to this theory, mathematicians borrowed stylistic approaches from poets. Poets had a fondness for cleverly constructing narratives that led to surprising twist reveals. Mathematicians shared the same aesthetic, according to Netz.
Netz, in all seriousness, proposes that this could be the main reason why Archimedes did calculus-style calculations of areas at all, and why he even turned to mathematical physics at all. The root cause is supposed to be not ordinary scientific or mathematical motivations, but Archimedes’s desire to do mathematics in the style of the poets: mathematics was “written, always, against the background of wider literary currents, emphasizing subtlety and surprise” (218).
According to Netz this is why Archimedes did calculus-style calculations of areas and volumes:
“Archimedes … picked up a particular technique, first offered by Eudoxus, because its subtlety … made a certain kind of surprise especially satisfying. Hence the infinitary methods.” (218)
And this is also what made Archimedes apply mathematics to physics:
“[Archimedes] saw the possibilities of applying geometry to a seemingly unrelated field – the study of centers of the weight in solids … – because there was a particular payoff of subtlety and surprise to be obtained by the bringing together of apparently irreconcilable, maximally distinct fields of study. This was rather like Callimachus’s poetry! Hence the mathematization of physics.” (218)
So there you go, calculus and mathematical physics are just side effects of mathematicians pursuing their true goal, which was to imitate the poets. That is some tin-foil-hat level of crackpottery, in my opinion.
It is one thing that Netz previously advanced his bizarre theory in a specialised monograph. Of course it must be possible for scholars to try out unconventional ideas. But to put this crazy stuff in a survey history with a straight face, as if this was objective information that any beginner in the field needs to learn, that is quite irresponsible, in my opinion. Certain chunks of this book are not an introduction to the history of Greek mathematics, but an introduction to the pet theories of Reviel Netz that no one but him believes.
Let’s look at some specific mathematical examples that are allegedly all about surprise, according to Netz.
For example, Archimedes found the area of one revolution of the Archimedean spiral. How do you think he’s going to prove this? Well, you have probably already seen how Archimedes found the area of a circle. Naturally readers of his more advanced treatise on spirals would already have read his more basic treatise on the circle.
Archimedes found the area of a circle by cutting it into wedges, as it were. Equal-angle pizza slices all the way around.
Naturally it makes a lot of sense to try the same idea for the spiral. The Archimedean spiral is like a circle but with a linearly growing radius. In polar coordinates, the radius r is proportional to the angle theta.
So when we apply the method we used for the circle to the spiral we get a bunch of equal-angle wedges that gradually get bigger and bigger. The radius grows linearly with the angle, so the radii of the wedges form an arithmetic progression. For every equal increment of the angle, the radii increase by the same amount, let’s say alpha. And the Archimedean spiral starts with radius zero, so the radii go: alpha, 2 alpha, 3 alpha, etc.
To get the area of the spiral we have to add up all the wedges. Obviously the areas scale like the square of the radii. Linear scaling of distances means square scaling of areas. So since the radii went alpha, 2 alpha, 3 alpha, the areas will be proportional to alpha^2, (2 alpha)^2, (3 alpha)^2, and so on.
So to get the area we have to add up a series of squares, the squares of numbers in an arithmetic progression. Indeed, Archimedes has a theorem that does exactly this. That is his Proposition 10.
Did you find any of this “surprising”? Hardly. It was a predictable extension of the idea used for the circle. And the trick of getting a complicated area or volume by an infinite series sum of simpler components is also a well established trick. Archimedes used the same trick for the area of a parabolic segment, for example, and Euclid used it too, for example for the volume of a tetrahedron. The sum of a geometric series was the key ingredient in those cases, and now for the spiral we need the same kind of theorem but for the squares of numbers in an arithmetic progression. Very predictable and business as usual for a Greek geometer.
But Netz doesn’t think so. According to Netz, the reader of Archimedes’s treatise is not supposed to have been able to see those things and instead they are supposed to have been baffled by the introduction of Proposition 10, that is to say, the sum of the series. They are not supposed to have been able to realise that this series is obviously the same kind of area calculation by series that had been well-known at least since Euclid, and that the particular terms of the series obviously correspond to the most natural way of cutting up the spiral area.
Here is what Netz says:
“Archimedes aims at surprise. The key point is that as proposition 10 is introduced, Archimedes makes all efforts to disguise its potential application. … The key observation – that the sectors in a circle behave as the series of squares on an arithmetical progression – is not asserted in advance. Instead, the application of proposition 10 is postponed and revealed only at the very last minute when, introduced in the middle of proposition 24, it finally makes sense of the argument. … Everything is designed for the sake of this denouement where, finally, the narrative of the treatise would make sense in a surprising turn. Ugly, misshapen proposition 10 is really about sectors in spirals: the duckling was a swan all along!” (149)
I think this is nonsense. I don’t think Archimedes’s readers would have been surprised at all by any of this.
Today we teach our mathematics students: when you read a theorem, before you look at the proof, take a few minutes to think about how you would prove it. Then when you read the proof you will understand it much better. You will know which parts are easy and obvious, because you have already thought of those yourself. And you will appreciate the difficult parts because you have realised when trying to prove it yourself that certain steps would have to involve some real work.
I bet Archimedes’s readers did the same. They get a treatise by Archimedes, a key result of which is the area of a spiral. Indeed, the treatise comes with a prefatory letter by Archimedes himself where he highlights the key results, so obviously you know where it’s heading. You don’t just start reading cold from A to Z.
And if you follow the elementary advice that we teach all our undergraduates, without which you will never get far in mathematics, to try to prove it yourself before reading the solution, then you will very quickly realise that the obvious approach is to cut the spiral area into wedges and sum the components, which will obviously lead to a series of squares of numbers in an arithmetic progression. So when you get to Archimedes’s Proposition 10 you will be far from surprised. On the contrary, you knew all along that he would have to do this sum.
Let’s look at another example of a so-called “ludic proof.” If you point a parabolic mirror at the sun, all the rays are reflected toward a single point, the focus of the parabola. Diocles proved this, and the “ludic” part is that he first proved some properties of tangents and normals of a parabola, and only then introduced a line parallel to the axis, which represent the rays of the sun. Surprise! It was about rays of the sun all along. Who could ever have guessed that saying something about the tangent first would be relevant to this! Except of course someone who has read the title of the treatise and has basic mathematical competence.
Here is how Netz describes it:
“[Diocles’s proof of the focal property of the parabola is] palpably Archimedean. The same emphasis on subtle surprise – down to the intentional delay in the construction of the parallel line, so that, throughout the argument, we do not yet see the relevance of any of it for the optics of rays of the sun.” (215)
So the “surprise” is that basic properties of the tangent of the parabola are relevant to the optics of rays of the sun. What a shocking reveal! Since the solar ray had not been drawn yet, there is no way we could have known this, according to Netz.
Once again, any mathematically competent person who looks at this problem for five seconds will realise that of course it is going to involve the tangent. The notion that mathematically competent readers would not have been able to see the relevance of theorems about tangents for the optics of rays of the sun is ridiculous. And yet that notion is the corner stone of Netz’s ludic proof interpretation of this episode.
There is another bit of nonsense here as well. Diocles talks about the tangent of a parabola, but Archimedes also talked about the tangent of a parabola. Aha! Therefore Diocles’s proof “is really a brilliant variation on an Archimedean theme” (215), in Netz’s words.
This is a way of thinking that perhaps makes sense in literary history. Poets and playwrights like to draw inspiration from earlier masterpieces and rework their themes in a new way. Netz tries to do the same thing for mathematics, but in my opinion the results are nonsensical.
What Netz is saying is like saying that if Person A gives a mathematical argument involving the derivative of a quadratic function, and then Person B gives a completely different argument that has nothing to do with the first one except that it too involves the derivative of a quadratic function, then Person B’s argument is a variation on Person A’s theme.
That’s rubbish. Of course tangents of parabolas show up regularly in mathematics. That doesn’t mean that anyone who talks about the tangent of a parabola is subtly reworking what earlier authors have done. That may be how literature works, but it is not how mathematics works.
So, in this case as in so many others, Netz’s “new history” is what you get when you look at Greek mathematics through eyes attuned to the humanities but not to mathematics. Indeed, Netz’s description of the mathematics is factually wrong as well. Archimedes and Diocles both state the tangent theorem in terms of “the intercept between tangent and ordinate” (215), according to Netz. No, that’s not right. It’s the intercept between the tangent and the axis. Not ordinate, axis.
But it is not my goal to catalogue all the mathematical errors in Netz’s book. If you take a humanities professor as your guide to mathematics then you have only yourself to blame anyway.
 11 October 2022, 6:49 pm
11 October 2022, 6:49 pm - 32 minutes 22 secondsThe “universal grammar” of space: what geometry is innate?
Geometry might be innate in the same way as language. There are many languages, each of which is an equally coherent and viable paradigm of thought, and the same can be said for Euclidean and non-Euclidean geometries. As our native language is shaped by experience, so might our “native geometry” be. Yet substantive innate conceptions may be a precondition for any linguistic or spatial thought to be possible at all, as Chomsky said for language and Kant for geometry. Just as language learning requires singling out, from all the sounds in the environment, only the linguistic ones, so Poincaré articulated criteria for what parts of all sensory data should be regarded as pertaining to geometry.
Transcript
The discovery of non-Euclidean geometry in the early 19th century was quite a wake-up call. It showed that everybody had been a bit naive, you might say.
Here’s an analogy for this. Suppose we had all been speaking one language, let’s say English. And we were all convinced that English is the only natural language. In fact, that question didn’t even arise to us; we simply assumed that English and language is the same thing. We even had philosophers “explaining why” English is a priori necessary. These philosophers had “proved,” they thought, that without English the very notion of linguistic communication or thought is impossible.
And then we discovered that there are French speakers and Chinese speakers. Oops. Very embarrassing. English is not necessary after all. It is not innate, it is not synonymous with language itself. For thousands of years we made those embarrassing mistakes because we were not aware of the existence of other languages.
That’s how it was with geometry. What I said about English corresponds to Euclidean geometry. For thousands of years, nobody thought of Euclidean geometry as one kind of geometry. Everybody thought of it as THE geometry. Geometry and Euclidean geometry was the same thing. Just as an isolated linguistic community thinks their language is THE language.And the philosophers I spoke of, Kant is an example of that. He argued that Euclidean geometry was a necessary precondition for having spatial experience or spatial perception at all, which is like saying that English is necessary for any kind of linguistic expression.
And already long before Kant, many people had been convinced by how intuitively natural and obvious the axioms of Euclidean geometry feel. Descartes for instance and many others made a lot of this fact. Remember how important it was to Descartes that our intuitions were truths implanted by God in our minds.
Well, we all think our native language is intuitive. And we think other people’s languages are not intuitive. This feeling is so strong that we think it must be objective. When we try to learn a foreign language, it feels impossible that anyone could think that was intuitive. And yet they do.
So apparently our intuitions can deceive us. We feel that our native grammar is much more natural than everyone else’s, but that’s a delusion. It felt like an objective fact, but it turned out to be subjective.
Could it be the same with geometry? Could the alleged naturalness and intuitiveness of Euclidean geometry turn out to be just an arbitrary cultural bias, like thinking English feels more natural than French?
So, ouch, we took quite a hit there with the discovery on non-Euclidean geometry. It exposed our insularity. It showed that things we had thought we had proven to be impossible were in fact perfectly possible and every bit as viable as what we had thought was the only way to do geometry.
And yet there is hope as well. The language analogy doesn’t just expose what an embarrassing mistake we made, or how non-Euclidean geometry hit us where it hurts. The language analogy also suggests a way out; a way to rise from the ashes.
We were wrong about the specific claim that Euclidean geometry is innate, because that’s like saying that English is innate. Nevertheless we were right too, one could argue.
Language is impossible without something innate. Everybody learns their native language with incredible fluency. Every child learns it somehow, with hardly any systematic teaching; they just pick it up naturally. And they do so at a very early age, when their general intelligence is still very limited.
Meanwhile, no animal comes anywhere near achieving the same feat. Nor any adult human for that matter. I’m better than a three-year-old child at any intellectual task, except learning French. Somehow the child is super good at that.
So clearly something about language is innate. The ability of language acquisition is innate. Some kind of general principles of language are innate.
Perhaps geometry is like language in this way. We have some innate geometry. Not specific Euclidean propositions or axioms maybe, but some kind of “geometryness” nonetheless. Some sort of more structural or general principles of geometry than specific propositions, just as our innate linguistic ability doesn’t contain anything specific to any one language but instead has to do with “languageness” in general.
In linguistics, this is called “universal grammar.” Every language has it own grammar, of course, but there are some more general or structural principles of language that are the same for all human languages. This common core is the universal grammar.
Here’s an example of such a principle that belongs to universal grammar. Consider the statement “the man is tall.” It is a declaration, an assertion. You could turn it into a question: “Is the man tall?” We turned the assertion into a question by moving the “is” to the front of the sentence. That’s how you make questions from statements: start with assertions—“the man is tall”—and move the “is” to the front—“is the man tall?” So that’s a recipe for making questions.
But consider now the statement “the man who is tall is in the room.” How do you form the corresponding question? There are two “is”’s in the sentence. So the rule “to form a question, move the is to the front” is ambiguous. Which of the is’s should we move?
It could become: “is the man who is tall in the room?” That works. But if we move the other is to the front we get nonsense: “is the man who tall is in the room?” Well, that didn’t work. It didn’t make a question, it just made gibberish. Even though we followed the same rule as before: move the “is” to the front.
If language was nothing but a social construction then it would be perfectly reasonable for children trying to form questions to come up with the gibberish one. If the child simply extracts general rules from a bunch of examples, it would have been perfectly reasonable for the child to have guessed that the general rule is: move the first “is” to the front of the sentence to form a question. Which would lead to the nonsense question “is the man who tall is in the room?”
In fact, however, “children make many mistakes in language learning, but never mistakes such as [this].” Apparently, “the child is employing a ‘structure-dependent rule’” rather than the much simpler rule to put the first “is” in front. Why? “There seems to be no explanation in terms of ‘communicative efficiency’ or similar considerations. It is certainly absurd to argue that children are trained to use the structure-dependent rule, in this case. The only reasonable conclusion is that Universal Grammar contains the principle that all such rules must be structure-dependent. That is, the child’s mind contains the instruction: Construct a structure-dependent rule, ignoring all structure-independent rules.” So although each language has its own grammar, there are some general principles like that that are universal: common to all languages. Those principles are hard-wired into the mind at birth.
This stuff about there being an innate “universal grammar” is the view Chomsky, the leading 20th-century linguist. The example and explanation I just quoted are from his book Reflections on Language. There are many debates about Chomskyan linguistics, but I’m going to assume Chomsky’s point of view for the purposes of this discussion, because its parallels with geometry are very interesting.
You might say that this view of language is Kantian in a way. We saw that Kant put a lot of emphasis on the necessary preconditions for certain kinds of knowledge. Geometry, for example, is not an external, free-standing theory that we can analyze with our general intellectual capacities. Rather, the fundamental concepts of geometry are bound up with the very cognitive structure of our mind itself.
Some things are purely learned through experience and convention, such as how to play chess or how to dance the tango. But some things are not like that. For instance, they way we experience color. The mind is made to see red and blue and to not see infrared and so on. That’s a fixed, domain-specific property of how our mind works. You can neither learn nor unlearn that through general intelligence. Color experience is just one of those basic things hardwired right into the brain.
From the Chomskyan point of view, language is like that as well. Language is not merely a social construct with man-made rules like chess or tango. Nor is it explicable in terms of general intelligence only.
Chess or tango you can learn by general intelligence. That is to say, if you spend enough time looking at people playing chess or dancing, you can eventually figure out what the rules are by general rational thinking such as pattern recognition, and forming preliminary hypotheses about how you think it works and then observing some more to check if you maybe need to revise the hypothesis to take into account some other possible circumstances or cases.
Color experience is not like that. You don’t learn to experience redness by watching other people. It just is. And if you’re not born with it, then you can’t learn it by general intelligence, like you can learn chess.
Language is similar to color and not similar to chess. You don’t learn color perception by watching others and using general intelligence to figure out the patterns and rules. General intelligence is not sufficient to sustain such a thing.
Many people overestimate the potential of general-purpose intelligence. Both Kant and Chomsky agree about this. Remember the tile of Kant’s work: a critique of pure reason. “Pure reason,” or general-purpose intelligence, is not by itself capable of generating human linguistic capacity or geometric experience.
The capacities of our mind depend much more than people realize on domain-specific conceptions. It is obvious that color experience is a hardwired specific domain of our cognitive structure and isn’t merely the outcome of some pattern-recognition process of general-purpose intelligence. But it’s less obvious that geometry is like that, or that language is like that. But Kant and Chomsky maintain that they are. According to them, we underestimate the extent to which basic geometrical and linguistic conceptions are intertwined with the very nature of our mind and our cognitive capacities.
So the wrong way to think about it would be like this. The human brain is a general-purpose thinking machine. Imagine a person in a prehistoric hunter-gatherer society. This person’s general-intelligence mind might think to itself: Well, it’s great that I’m so smart. I can learn many things, like which plants are poisonous; I can figure things out like how to make fire, how to use tools and so on. But gee, wouldn’t it be handy if I could communicate my thoughts to others. Then we could organise collaborations, learn from each other’s experiences, and so on. I know, let me invent language, that will work for this.
From the Chomskyan point of view this story is wrong because it overestimates the general-purpose mind. In fact, note that I described what the pre-linguistic mind was thinking by using language. But I was talking about a hypothetical stage in history in which there was no language. Does it even make sense to imagine such a thing as thought without language? No, according to Chomsky. The very nature of thought itself cannot be separated from language like that.
The story of the hunter-gatherer inventing language is no more plausible than the story that he invented color experience by discovering that certain wavelengths of electromagnetic radiation were associated with grass, others with fruit, and so on.
Instead of thinking of the mind as starting from general-purpose intelligence and then inventing domain-specific things like color and language, we should perhaps think of it exactly the other way around. The mind is made up of the domain-specific skills. Those are the fundamental cognitive starting-points. Insofar as we have any general-purpose intelligence, that comes from piecing together the domain-specific skills. Not the other way around.
From an evolutionary point of view, the human mind perhaps evolved by adding domain-specific modules one by one: first color, then a hundred thousand years later geometry, then a hundred thousand years later language, and so on. We don’t have general-purpose intelligence. We only have the sum of our modular parts. But eventually these modules became so advanced, and combined in such fruitful and powerful ways, that we fool ourselves into thinking that we have general intelligence, “pure reason.” But at bottom our precious “pure reason” actually still depends more than we realize on domain-specific preconceptions hardwired into our cognitive capacities. That’s what Kant said about geometry and that’s what Chomsky said about language.
So in this way we can “save Kant.” The discovery of non-Euclidean geometries was a blow to Kant’s idea of the innateness of geometry. Kant associated the intuitiveness of Euclidean geometry with its innateness. But native languages are intuitive, yet they are not innate. And geometry could be the same, because just as there are many languages there are many geometries. This shows that intuitive and innate is certainly not the same thing, so it calls into question the Kantian story that the mind is constrained by pre-programmed conceptions.
We save Kant with the rebuttal that in fact language too is innate after all. Even though there are many languages that all differ in fundamental respects, nevertheless there is some universal languageness that is common to all and without which language learning would be impossible in the first place.
Same with geometry. Instead of focusing on the differences between Euclidean and non-Euclidean geometries and concluding from this that no one geometry could be a necessity of thought, we should instead focus on the more fundamental and structural preconceptions common to all geometries, without which any kind of geometry would be unthinkable at all.
Or we can put it like this. Thought presupposes language. When you think, you think in terms of words and sentences. Of course thought does not presuppose any specific language. You can think the same thing in English or German. Nevertheless thought does presuppose that you use some language. There is no “pure thought,” or hardly any, that does not involve words.
It’s funny: thought cannot exist without language, yet you can switch the entire language and still have the same thought. So there’s both dependence and independence.
Kant says basically the same thing but for geometry. You can’t have spatial perception or spatial reasoning without geometrical presuppositions. Just as you can’t think without presupposing some language, so you can’t geometrize without presupposing some geometry.
The choice of which language or which geometry you take as the basis for thought is arbitrary. As Kant says, it’s a synthetic a priori, not an analytic a priori. That is to say, it is not logically necessary that we must use Euclidean geometry as the presupposition for all our spatial experience. But it is necessary that we must make some such presupposition.
Remember, as Kant said, we don’t have direct access to objective physical reality. We only know the outside world through perception which is always necessarily interpreted. The presuppositions of that interpretation are arbitrary—in fact, it’s arbitrary in two ways one might say: one good and one bad. It’s arbitrary in a “bad” way in that it is subjective. It lacks objective justification. But it’s also arbitrary in a “good” sense, namely that it doesn’t necessarily matter all that much which interpretation we choose.
Just like language. It is arbitrary that I’m speaking English. There’s no objective or logical reason for why English is any better than any other language. But it’s also arbitrary in that it doesn’t matter. I could have said the same things in some other language. And in fact it’s only because of my choice of some arbitrary language that I am able to say anything at all.
Same with geometry. Our minds think in terms of Euclidean geometry even though that has no absolute logical justification. Yet it would be a mistake to criticize this as arbitrary subjectivity. Because it is only because I have some geometrical preconceptions at all, no matter how subjective, that I am able to reason spatially and have spatial perception and experience in the first place.
The analogy that geometry is like language is suggestive in other respects as well. Here’s one interesting question. When a child is learning their native language by picking up the speech of their parents and their environment, how does the child know which sounds are language and which sounds are other kinds of noises? It’s a pretty difficult problem, isn’t it?
Suppose you had to program a computer to detect and recognize speech. What criteria could you define by which the computer could tell if any given sound is linguistic or not? Words come in many forms: you can scream them, whisper them, sing them. Those are very different as sounds, but somehow you have to be able to tell that they are all words. And you have to be able to tell that other sounds are not linguistic, such as a doorbell, a barking dog, the sizzling of a frying pan, and so on.
You have the same problem in geometry. Among all the sensory impressions we are bombarded with every second, which ones should be regarded as geometrical, and which not? If geometry is like a language, a child must have some criteria by which to answer this. Just like the child somehow picks out linguistic sounds from the environment and lets that shape their native language, so also the child must pick out geometric features of the environment and let that shape their native geometry. This is how their intuitive geometry can become either Euclidean or non-Euclidean depending on the environment, just as their native language can become English or Russian or whatever.
So: What parts of all our sensory impressions have to do with geometry? You must know that first, before you can start thinking about whether those impressions are Euclidean or non-Euclidean.
Poincaré had a very elegant solution to this problem. Here’s his criterion for telling geometry from non-geometry. It goes like this: Among all sensory impressions, those are geometrical that you can cancel through self-motion.
Let me explain what this means by an example. I have a piece of paper. One side is white and the other side is red. I hold the paper up with the white side facing toward you. Then I rotate it so that the red side is facing you. This is a geometrical transformation: it has to do with rotation, with position. You know that it was geometrical because you could walk around and stand on the other side and then you would see the white side of the paper again. So you could cancel the transformation in impressions, you could restore the original sensory impression, through self-motion. By moving yourself. Not by manipulating the environment, but only by moving around in it.
There are many transformation of sensory impressions that are not like that. That are not cancelable or reversible through self-motion. Including other kinds of switches from white to red. Pour a white liquid, like a lemon sports drink, into a glass. And then pour in something very red, like beet juice or some strawberry syrup. The liquid in the glass went from white to red, just like the paper did when I flipped it over.
But the liquid is different, because you can’t cancel it this time by moving around and looking at it from another point of view. This is precisely why it is not geometrical. The paper example should be interpreted in terms of geometry. If someone asks: what happened? Then for the paper example you would give an explanation in geometrical terms: the object rotated 180 degrees. But for the liquid example you would give an explanation in non-geometrical terms: the red liquid “colors over” the white one by some kind of, I don’t know, chemistry somehow; not geometry anyway.
So there you have a very clear criterion for selecting from the environment which things are to be accounted for in terms of geometry and which not. Cancelability through self-motion.
Before a child can tell if their parents speak French or Russian, they must be able to distinguish which sounds are linguistic at all. And before we can tell if the space around us is Euclidean or non-Euclidean, we must first be able to distinguish which sensory impressions have to do with geometry at all. Poincaré’s criterion in terms of self-motion answers this problem.
So this suggests that it is only through motion that we can impose a geometric interpretation on our visual impressions. It may feel to us as if our sense of sight is inherently geometrical: geometry is visual, it lives in the eyes. But Poincaré’s perspective suggests that it’s more complicated than that.
Vision becomes endowed with geometry only through its interaction with self-motion. If we could not move ourselves or our eyes, our sense of sight would be as un-geometrical as our sense of taste or smell. It would be just a bunch of qualitative impressions with no particular structure.
With sense and smell, you can tell when one thing is different from another, but you can’t do much more than that. There is no “Pythagorean Theorem of taste” that allows you to calculate the taste-distance between wine and beer if you know the distances between beer and water and water and wine. Taste impressions don’t have geometrical structure or any comparable kind of structure. And if we didn’t have self-motion then sight would be like that as well.
There’s a passage in Rousseau’s Emile that fits this perspective. It goes like this:
“It is only by our own movements that we gain the idea of space. The child has not this idea, so he stretches out his hand to seize the object within his reach or that which is a hundred paces from him. You take this as a sign of tyranny, an attempt to bid the thing draw near, or to bid you bring it. Nothing of the kind, it is merely that he has no conception of space beyond his reach.”
So imperfect capacity for self-motion goes with imperfect understanding of space, it seems, in the child. Of course Rousseau was writing long before Poincaré. I used Poincaré as the point person for this perspective about the role of self-motion in geometry but indeed the basic ideas go back centuries before. Poincaré explains his view very well in his book La Valeur de la Science of 1905. But that’s the culmination of a tradition of more than two centuries.
For example, many philosophers had debated the following question: Suppose a person who has been blind all their life has an operation that makes them able to see. Can they then, from visual impressions alone, tell for example a cube from a sphere? They already knew the difference by touch, but could they then automatically make the connection between that and sight, or would they have to learn to recognize things by sight through experience?
This is the so-called “Molyneux’s question.” Molyneux raised it in 1688. Obviously it has a lot to do with the question of whether geometry is innate, or whether it is learned by experience.
This thing about a blind person becoming sighted was not just a thought experiment. It could be done through surgery in some cases. Let me read to you a report of the experiences of such a person. This is from the Philosophical Transactions of 1728. A boy who was 13 years old and had been blind all his life got his sight back through a surgical procedure. And his reactions were as follows.
“When he first saw, he was so far from making any Judgment about Distances, that he thought all Objects that he saw touch’d his Eyes, (as he express’d it) as what he felt, did his Skin.”
“He knew not the Shape of any Thing, nor any one Thing from another, however different in Shape, or Magnitude; but upon being told what Things were, whose Form he before knew from feeling, he would carefully observe them, that he might know them again; but having too many Objects to learn at once, he forgot many of them. One Particular only (tho’ it may appear trifling) I will relate; Having often forgot which was the Cat, and which the Dog, he was asham’d to ask; but catching the Cat (which he knew by feeling) he was observ’d to look at her stedfastly, and then setting her down, said, So Puss! I shall know you another Time.”
“He was very much surpriz’d, that those Things which he had lik’d best, did not appear most agreeable to his Eyes, expecting those Persons would appear most beautiful that he lov’d most, and such Things to be most agreeable to his Sight that were so to his Taste.”
“We thought he soon knew what Pictures represented, which were shew’d to him, but we found afterwards we were mistaken; for about two Months after he [became sighted], he discovered [that] they represented solid Bodies; when to that Time he consider’d them only as Party-colour’d Planes, or Surfaces diversified with Variety of Paint; but even then he was no less surpriz’d, expecting the Pictures would feel like the Things they represented, and was amaz’d when he found those Parts, which by their Light and Shadow appear’d now round and uneven, felt only flat like the rest; and ask’d which was the lying Sense: Feeling or Seeing? Being shewn his Father’s Picture in a Locket at his Mother’s Watch, and told what it was, he acknowledged a Likeness, but was vastly surpriz’d; asking, how it could be, that a large Face could be express’d in so little Room, saying, It should have seem’d as impossible to him, as to put a Bushel of any thing into a Pint.” (That is to say, a larger volume into a smaller.)
That’s quite entertaining but also quite significant evidence for the debates we have been considering. Clearly, learning the geometry of sight was a bit like learning a language for this person who became sighted. He didn’t immediately understand the geometrical structure of visual impressions, so clearly all of that is not completely innate. So it speaks against a Kantian account that takes Euclidean geometry to be a precondition of any geometrical thought or geometrical sensory perception.
But the story of the boy who became sighted fits quite well with a Poincaré-type account in which the geometry of sight can only be developed gradually through experience and coordination with self-motion.
Nevertheless, you can still say that Kant was right in a way. Poincaré is in a sense neo-Kantian. According to Poincaré, Euclidean geometry is not innate, but some geometrical notions are. The mind is predisposed to discern geometrical aspects of its surroundings. Hardwired into the mind are not all of Euclid’s axioms but still a good bit of geometry, such as the categorisation of which perceptions are related to geometry at all, and perhaps related to this some concepts such as displacement, rotation, and so on.
So, those are the ways in which geometry is like language. Both are part innate and part shaped by the environment. To adopt a particular language or a particular geometry is to fit your thoughts into an arbitrary and subjective framework. But that’s a good thing because there are no objective frameworks, and without some such conceptual framework, thinking could never even get off the ground in the first place.
 20 May 2022, 6:58 am
20 May 2022, 6:58 am - 30 minutes 40 seconds“Repugnant to the nature of a straight line”: Non-Euclidean geometry
The discovery of non-Euclidean geometry in the 19th century radically undermined traditional conceptions of the relation between mathematics and the world. Instead of assuming that physical space was the subject matter of geometry, mathematicians elaborated numerous alternative geometries abstractly and formally, distancing themselves from reality and intuition.
Transcript
The mathematician has only one nightmare: to claim to have proved something that later turns out to be false. There are thousands of theorems in Greek geometry, and every last one of them is correct. That’s what mathematical proofs are supposed to do: eliminate any risk of being wrong. If this armor is compromised, if proofs are fallible, then what’s left of mathematics? It would ruin everything.
But the nightmare came true in the 19th century. What had been thought to have been proofs were exposed as fallacies. Top mathematicians had made mistakes. Mistakes! Like some commoner. It’s going to be hell to pay for this, as you can imagine.
I’m referring to Euclid’s fifth postulate, the parallel postulate. Euclid’s postulate had rubbed a lot of people the wrong way even since antiquity. It sounds more like a theorem.
The earlier postulates were very straightforward: there’s a line between any two points, stuff like that. Very primitive truths. It makes sense that the bedrock axioms of geometry should be the simplest possible things, such as the existence of a line between any two points.
The parallel postulate, by contrast, is not very simple at all. It’s not a primordial intuition like the other postulates. It states that two lines will cross if a rather elaborate condition is met. That’s the kind of thing theorems say. This particular type of configuration has such-and-such a particular property. That’s how theorems go in Euclid. Very different from the other postulates, which are things like: any line segment can be spun around to make a circle.
So, people tried to prove the parallel postulate as a theorem. Many felt that it should not be necessary to assume the parallel postulate as Euclid had done. The parallel postulate should be a consequence of the core notions of geometry, not a separate assumption.
Many people tried to “improve” on Euclid in this way. From antiquity all the way to the 19th century. Around 1800, people like Lagrange and Legendre proposed so-called proofs of the parallel postulate. Those are big-name mathematicians. Their names are engraved in gold on the Eiffel Tower. Lagrange was even buried in the Panthéon in Paris. Elite establishment stuff.
But even these bigwigs were wrong. Their proofs contain hidden mistakes. It’s astonishing that this was more than 2000 years after Euclid. People tried to improve on Euclid for millennia. And not a few claimed to have succeeded. But the fact is that Euclid was right all along. The parallel postulate really does need to be a separate assumptions, just as Euclid had made it. It cannot be proved from the other axioms, as so many mathematicians during those millennia had mistakenly believed.
The Greeks, you know, they were really something else. It’s so easy to make subtle mistakes in the theory of parallels. History shows that there are a hundred ways to make tiny invisible mistakes that fool even the best mathematicians. Top mathematicians who were never wrong about anything else stumbled on this one issue.
Somehow Euclid got it exactly right. He didn’t make any of those hundred mistakes that later mathematicians did. That’s not luck, in my opinion. Arguably, the Greeks were more sophisticated foundational geometers than even the Paris elite in 1800. Unbelievable but true. Euclid’s Elements is not a classic for nothing. Euclid is not a symbol of exact reasoning because of some lazy Eurocentric birth right. Euclid’s Elements really is that good.
When Euclid made the parallel postulate an axiom, he seems to be suggesting that it cannot be proved from the other axioms. And he was right. But, as I said, many people had a hunch that he was wrong about this. They thought it would be impossible for the other axioms to be true and the parallel postulate not true.
So many mathematicians figured they could prove this by contradiction: Suppose the parallel postulate is false. If we could show that that assumption would contradict other geometrical truths, then the assumption must be false. So this way we could prove that the parallel postulate must be true, by showing that it would be incoherent or impossible for it to be false.
Indeed, it was found that negating the parallel postulate had various strange consequences. For example, if the parallel postulate is false then squares do not exist. Suppose you try to make a square. So you have a base segment, and you raise two perpendiculars of equal length from the two endpoints of the segment. Then you connect the two top points of these two perpendiculars. That ought to make a square. In Euclid’s world it does.
But proving that this really makes a square requires the parallel postulate. If the parallel postulate is false, one can instead prove that this construction does not make a square but rather a weirdly disfigured quadrilateral. Because the last side of the “square” doesn’t make right angles with the other sides. So even though you made sure you had right angles at the base of the quadrilateral, and that the perpendicular sides were equal, the fourth and final side still somehow manages to “miss the mark” so to speak. It makes non-right angles.
It’s as if the sides are sort of bent. It’s as if you had four perfectly equal sticks of wood, but then you stored them carelessly and they were exposed to humidity and so on and they were warped. So now they’re kind of mismatched in terms of length and straightness, and when you try to piece them together to make a square they don’t fit right. They make some wobbly not-quite-square shape.
Doing geometry without Euclid’s parallel postulate feels a bit like that. It’s sort of bent out of shape and nothing fits the way it should anymore.
One person who investigated this was Saccheri. He wrote a big book discussing this misshaped square and other things like that, in 1733. Saccheri felt that he had justified Euclid’s parallel postulate by examples such as theses. The square that’s not a square and other such deformities, Saccheri declared to be “repugnant to the nature of the straight line.”
But one might say that he used this emotional language to compensate or cover up a shortcoming in the mathematical argument. He had indeed showed that if the parallel postulate is false then geometry is weird. Then you have squares that don’t fit, and other things that feel like doing carpentry with crooked wood.
But weird is not the same as self-contradictory. Despite their best efforts, mathematicians could not find a clear-cut proof that negating the parallel postulate led to directly contradictory conclusions. This is why Saccheri had to say “repugnant” rather than contradictory. You only get “repugnantly” deformed squares, not direct contradictions such as 2=1 or a part being greater than the whole. Those things would be logical contradictions and you wouldn’t need emotions like repugnance.
In fact, a hundred years after Saccheri, mathematicians came to accept that this strange non-Euclidean world of the warped wood is not contradictory. It is coherent and consistent. It is merely another kind of geometry. An alternative to Euclid.
People used to shout and scream that all kinds of things were repugnant, such as homosexuality, for instance. That doesn’t really prove anything except the narrow-mindedness of those accusers. Mathematicians had been equally narrow-minded. They had tried to justify the status quo for thousands of years. They had tried to prove that their way of doing things–their geometry–was the only right way. Only in the 19th century did they finally realize that it was much more productive to embrace diversity, to accept all the geometries of the rainbow.
For so many years mathematicians could not get away from the idea that the “straight” squares of Euclid were the only “normal” ones, and that the “repugnant” alternative squares of non-Euclidean geometry were birth defects. But they were wrong. Non-Euclidean geometry is as legitimate as any other. It was a creative watershed shift in perspective in mathematics to finally accept this instead of trying to prove the opposite.
Here’s how Gauss, the greatest mathematician at the time, put it in the early 19th century. Negating Euclid’s parallel postulate “leads to a geometry quite different from Euclid’s, logically coherent, and one that I am entirely satisfied with. The theorems [of this non-Euclidean geometry] are paradoxical but not self-contradictory or illogical.” “The necessity of our [Euclidean] geometry cannot be proved. Geometry must stand, not with arithmetic which is pure a priori, but with mechanics.”
Geometry has become like mechanics in the sense that it is empirically testable. The theorems of geometry are not absolute truths but hypotheses like the hypotheses of physics that have to be checked in a lab and perhaps corrected if they don’t agree with measurements.
For example, Euclid proves that the angle sum of a triangle is 180 degrees. But this theorem depends on the parallel postulate, just as Euclid’s proof reveals it to do. In non-Euclidean geometries, angle sums of triangles will be different. So that’s something testable. Measure some triangles to see which geometry is right, just as you drop some weights or whatever in a physics lab to see which law of gravity is right.
Let me quote Lobachevsky, one of the other discoverers of non-Euclidean geometry. Here’s how he makes this point in his book of 1855: “[Non-Euclidean geometry] proves that the assumption that the value of the sum of the three angles of any rectilinear triangle is constant, an assumption which is explicitly or implicitly adopted in ordinary geometry, is not a consequence of our notions of space. Only experience can confirm the truth of this assumption, for instance, by effectively measuring the sum of three angles of a rectilinear triangle. One must give preference to triangles whose edges are very large, since according to [Non-Euclidean geometry], the difference between two right angles and the three angles of a rectilinear triangle increases as the edges increase.” So you need big triangles to tell the difference, just as the earth is round but looks flat from where we’re standing because we only see a small part of it. In the same way we need big triangles to detect the nature of space. Therefore Lobachevsky recommends that we should use astronomical measurements for this: “The distances between the celestial bodies provide us with a means for observing the angles of triangles whose edges are very large.”
Let’s think about the logical structure involved in the realization that non-Euclidean geometry is possible. It used to be thought that Euclid’s parallel postulate was a necessary consequence of the other axioms. Although Euclid seems to have been wise enough to realize that it was not, others erroneously believed that this was a mistake rather than an insight on Euclid’s part.
So the question is: Does the parallel postulate follow from the other axioms? If the answer is yes, then the way to settle matter is to provide a proof, a deduction, starting from the other axioms and ending up with the parallel postulate. So that would be like adding another theorem to Euclid’s Elements.
On the other hand, suppose the answer is no, the parallel postulate does not follow from the other axioms. How then could we prove that? It’s very different in this case. It is no longer about proving a theorem. Rather it is about proving that something cannot be proved. It’s much more “meta” than just proving a particular theorem.
But here’s how you do such a thing. Consider this analogy. Suppose someone believes that all odd numbers are prime numbers. 3 is prime, 5 is prime, 7 is prime, and so on. So someone has become convinced that all odd numbers are prime numbers, and they set out to prove it. The start with what it means to be odd, and from that information they try to prove that that implies that it must be prime as well.
But this is of course wrongheaded. Trying to prove that being prime follows from being odd is just as futile as trying to prove that the parallel postulate follows from the other axioms of Euclid.
How could we set this mathematician straight? How could we prove that what he’s trying to prove is impossible to prove? The way to do this is not by some general proof, but by a specific example.
Look at the number 9. It’s odd, but it’s still not prime. Because it’s 3 times 3, so not a prime number.
The obvious way to interpret this is to say that the guy was wrong with his hypothesis. The claim that being odd implies being prime is false.
But from a logical point of view it is interesting to look at it in slightly different terms. Let’s not think about it in terms of right and wrong. Logic doesn’t care about right and wrong. Logic cares only about what follows from what. When logic looks at a proposition, logic doesn’t ask: is it true or false? Logic asks: does it follow from a particular set of axioms?
Logic is about entailment relations. What follows from what. Logic doesn’t care what assumptions or axioms you use. It only cares about what follows from those axioms.
So in terms of our example with the odd numbers, we shouldn’t focus on the question “are all odd numbers prime numbers?” Instead, from a logical point of view, the better question is: “does being odd entail being prime?” Or “is primeness a logical consequence of oddness?”
We had a counterexample: the number 9. From the logical point of view, we interpret this a bit differently. Not as proving the falsity of the conjecture, because we’re not interested in true or false. Instead, what the example of 9 shows is that it is not possible to derive the property of being prime from the property of being odd.
When we put it this way, we have an answer to that challenging meta question: How can we prove that it’s impossible to prove something? We just did! It’s impossible to prove primeness from oddness. Because if there was a proof that showed that any odd number must be prime, then that proof would apply to 9, since it’s odd, and it would prove that 9 is prime, which it is not. Therefore no such proof could exist.
It was the same in geometry. People thought the parallel postulate was a logical consequence of the other axioms. The way to prove this wrong is to exhibit an example in which the other axioms are true but the parallel postulate is false. Just as in the number theory case we had to find an example where oddness was true but primeness was false.
This is indeed what happened. Mathematicians discovered something that corresponded to the number 9. This proved the logical independence of the parallel postulate, just as the number 9 proves that primeness is not a logical consequence of oddness.
In the geometry case, the role of the number 9 was played by models of hyperbolic geometry. These are visualizations that prove that there are perfectly coherent worlds in which the parallel postulate is false while all the other axioms of Euclid are true.
Once mathematicians started thinking in these kinds of terms, it turned out to be not so difficult to find models like that. Mathematicians really could have done that a lot earlier. Even hundreds of years earlier, or even in Greek times. It’s a bit of an embarrassment that it took so long.
Imagine how embarrassing it would be to sit around for hundreds of years trying to prove that all odd numbers are prime numbers, and ranting about how the very idea of an odd non-prime is “repugnant to the nature of an odd number” only to then discover that, whoops, actually there’s a pretty straightforward counterexample right there, the number 9.
The mistake mathematicians made in geometry was of course not quite so glaring but still in a way it was quite similar. The counterexamples were not that difficult to find. Once mathematicians opened their minds to the possibility of such counterexample, they found them fairly easily.
Mathematicians had missed these rather simple counterexamples for thousands of years because of their closed-minded perspective and preconceived notions. Mathematicians had relied too much on emotions, intuitions, such as repugnance. And they had assumed that there can only be one reasonable geometry, because geometry must correspond to physical space.
Mathematicians could not afford to make those mistakes again. These mistakes are what made the nightmare come true, namely that what mathematicians had thought they had “proved” was actually false.
It was a time for soul searching and repentance. And the lessons from this whole embarrassment were quite obvious. The sources of error were intuitions, such as feelings about how straight lines “should” behave, as well as the notion that geometry means the geometry of the physical space around us.
Those ideas were the losers of the story. The winner was logic. The breakthrough had come by detaching geometry from intuition and reality. By abstracting geometry away to its logical structure only. That was the winning perspective.
To spell out what this means for geometry and its relation to the world, let me quote Einstein’s essay Geometry and Experience. Einstein wrote this is 1921, but he is really just summarizing a standard consensus that had been firmly established decades earlier. But why not use the words of the famous Einstein, they are as good as any to make this point. Here’s what Einstein says:
“How can it be that mathematics, being after all a product of human thought which is independent of experience, is so admirably appropriate to the objects of reality? Is human reason, then, without experience, merely by taking thought, able to fathom the properties of real things? In my opinion the answer to this question is, briefly, this: as far as the propositions of mathematics refer to reality, they are not certain; and as far as they are certain, they do not refer to reality. It seems to me that complete clarity as to this state of things became common property only through that trend in mathematics, which is known by the name of ‘axiomatics’.” That’s Einstein’s word for what I called the logic perspective. Same thing. Einstein continues:
“Let us for a moment consider from this point of view any axiom of geometry, for instance, the following: through two points in space there always passes one and only one straight line. How is this axiom to be interpreted in the older sense and in the more modern sense?”
“The older interpretation [is]: everyone knows what a straight line is, and what a point is. Whether this knowledge springs from an ability of the human mind or from experience, from some cooperation of the two or from some other source, is not for the mathematician to decide. He leaves the question to the philosopher. Being based upon this knowledge, which precedes all mathematics, the axiom stated above is, like all other axioms, self-evident, that is, it is the expression of a part of this a priori knowledge.”
“The more modern interpretation [is]: geometry treats of objects, which are denoted by the words straight line, point, etc. No knowledge or intuition of these objects is assumed but only the validity of the axioms, such as the one stated above, which are to be taken in a purely formal sense, that is, as void of all content of intuition or experience. These axioms are free creations of the human mind. All other propositions of geometry are logical inferences from the axioms (which are to be taken in the nominalistic sense only). In axiomatic geometry the words ‘point’, ‘straight line’, etc., stand only for empty conceptual schemata. That which gives them content is not relevant to mathematics.”
“‘Practical geometry’ [arises if we] add the proposition: solid bodies are related, with respect to their possible dispositions, as are bodies in Euclidean geometry of three dimensions. Then the propositions of Euclid contain affirmations as to the behavior of practically-rigid bodies. All length-measurements in physics constitute practical geometry in this sense, so, too, do geodetic and astronomical length measurements, if one utilizes the empirical law that light is propagated in a straight line, and indeed in a straight line in the sense of practical geometry. I attach special importance to the view of geometry, which I have just set forth, because without it I should have been unable to formulate the theory of relativity. From the latest results of the theory of relativity it is probable that our three-dimensional space is approximately spherical, that is, that the laws of disposition of rigid bodies in it are not given by Euclidean geometry, but approximately by spherical geometry.” That is to say, the angle sums of triangles are more than 180 degrees.
So all of that I quoted from Einstein. But Einstein speaks for basically the entire mathematical community here. He is describing what was, in his time, the standard view that almost everyone took for granted.
Indeed, these points about mathematics turning to pure axiomatics and so on, apply not only to geometry but to mathematics as a whole. Mathematicians took that lesson to heart and never looked back, basically. So the discovery of non-Euclidean geometry was the birth of modernity, you might say, in mathematics. It led mathematicians to conceive their field exclusively in terms of logic and formalism, and forget everything about intuition or the idea that mathematics is linked to physical reality. And that’s pretty much where we are today, almost two centuries later, with few exceptions.
In the 19th century, you could be forgiven for thinking that this was a case of straightforward progress. Mathematicians had simply discovered the right way to do mathematics, or the best way known so far anyway. The new logic perspective was simply better than the old intuitive or empirical stuff. We shedded the old errors like so many superstitions and became enlightened.
Around 1900, that was a pretty credible narrative. The logic perspective had gone from win to win, and done a clean sweep of mathematics. Everything it touched seemed to become instantly clearer and better. Hilbert was a leading mathematician at this time who may be taken as a symbol of this. He turned from field to field and made everything clear and clean and modern with this logical Midas’ touch.
But the winning streak did not last forever. With one knock-out win after another behind him, Hilbert turned to the foundations of the entire subject of mathematics and tried to do the same trick there. Many people were optimistic. The trick had worked every time before, and now the world’s greatest mathematician was going to use it to definitively settle all the questions of the foundations of mathematics, such as proving that mathematics is consistent.
But the trick broke this time, even though it had worked every time before. Hopes of a quick victory proved as delusional as the equally hubristic delusions of the war planners who were marching into the First World War at the same time.
The world came crashing down around the great Hilbert. He was German, and these were not good times for Germany. First the students and younger generation died in the war. Then the many prominent Jewish faculty were driven out of the country. Hilbert’s once vibrant university was quickly turned into a shadow of its former self. Hilbert himself contracted a rare decease for which the only treatment was eating lots and lots of raw liver every day.
1933 was a year of not one but two disasters. The Nazis took power, but there was an equal blow in the world of mathematics, when Gödel proved that the logician’s dream was impossible. Logical formalism could not prove its own consistency. In other words, the program of detaching mathematics from intuition and experience turned out to be inherently limited. Its utopian dream proved to be unreachable, and demonstrably so in fact.
Kant used a beautiful analogy that is relevant here. It goes like this:
“Deceived by the power of reason, we can perceive no limits to the extension of our knowledge. The light dove cleaving in free flight the thin air, whose resistance it feels, might imagine that her movements would be far more free and rapid in airless space.”
Which is of course not true. The dove may think that air causes nothing but resistance, but if all air is removed, the dove would quickly be taught a different lesson of course. Not only would the dove crash to the ground at once, it would also suffocate in seconds.
A similar fate awaited the movement to purge mathematics of intuition and physical content. People like Hilbert were so keen to remove the old dependence on intuition and the physicality of geometry as if these things were nothing but “air resistance” that prevented the flight of pure logic in a perfectly clean vacuum.
But birds cannot fly without air, and neither could mathematics. Gödel’s theorem of 1933 proved that logical formalism cannot prove it own consistency, which in terms of this analogy is like proving that the dove cannot fly in a vacuum.
This setback within mathematics was perhaps just as unnerving to Hilbert and other mathematicians as all those jarring disasters that were piling on in the outside world. It’s cruel joke of history that it had both these worlds collapse at the same time.
Maybe the parallel extends further. World War One was a horror of horrors, but that didn’t prevent us from doing it all over again soon thereafter. And we still don’t know how to get rid of war.
Mathematics has a similar attachment to formalism and logic. As with war, the romantics among us are not too happy about formalistic mathematics. Its power cannot be denied. Some, or maybe even many, of its victories were for the best. But still it does not feel right in one’s heart to drill young people into an army of formalists. Seeing mathematics as nothing but logical inferences from arbitrary axioms is as heartless as realpolitik. It reigns to this day, despite a now checkered record, because the only alternatives are hippie fantasies with no realistic prospects of ruling. Modern mathematics and modern politics are alike in this regard.
Well, that makes for a bleak ending. Perhaps non-Euclidean geometry does not deserve to be associated with all this misery. It’s not non-Euclidean geometry’s fault that mathematicians had made mistakes about the parallel postulate. Nevertheless the impact of the discovery of non-Euclidean geometry on the mathematical psyche was dramatic and long-lasting. It sent mathematicians on a soul-searching bender, the hangover of which is still felt today.
 20 February 2022, 11:00 pm
20 February 2022, 11:00 pm - 30 minutesRationalism 2.0: Kant’s philosophy of geometry
Kant developed a philosophy of geometry that explained how geometry can be both knowable in pure thought and applicable to physical reality. Namely, because geometry is built into not only our minds but also the way in which we perceive the world. In this way, Kant solved the applicability problem of classical rationalism, albeit at the cost of making our perception of the world around us inextricably subjective. Kant’s theory also showed how rationalism, and philosophy generally, could be reconciled with Newtonian science, with which it had been seen as embarrassingly out of touch. In particular, Kant’s perspective shows how Newton’s notion of absolute space, which had seemed philosophically repugnant, can be accommodated from an epistemological point of view.
Transcript
Rationalism says that geometrical knowledge comes from pure thought. Empiricism says that it comes from sensory experience. Neither is very satisfactory, because geometry is clearly both: it’s too good a fit for the physical world to be only thought, and it relies too much on abstract proofs to be only experience.
So it seems the “right” philosophy of mathematics must be a little bit of both. But how? Rationalism and empiricism mix like oil and water. They are so different, so opposite, that it seems impossible to find any sort of middle ground that has half of each.
Nevertheless there is a golden mean of sorts. The philosophy of Kant. Immanuel Kant, the late 18th-century philosopher. His view of geometry is in a way the best of both worlds: combining the best rationalism with the best of empiricism. Let’s see how he pulled that off.
Kant’s theory is another innateness theory. Geometry is innate; it is hardwired into our minds. That’s what the rationalists said too, of course. Innateness was why the uneducated boy in Plato’s Meno could reach substantial geometrical insights without instruction. The innate intuitions of geometry are reliable because God is not a deceiver, said Descartes. And they apply to the physical world, because the Creator put into our minds the same ideas he had used to design the universe, said Kepler and others.
But Kant’s innateness is different. He lived a century and a half after Kepler and Descartes, and the reliance on God to support the rationalist worldview had become much less fashionable during the intervening years. And indeed Kant does away with it.
By taking geometry to be innate, Kant automatically inherits the best of rationalism. That is to say, he is able to account for the prominent role of pure reason in geometry in a convincing way, just as the earlier rationalists had done before him.
But Kant solves the weakness of rationalism very differently. If geometry is innate and susceptible to purely theoretical elaboration, then why does it always agree so well with experience? Not because “God was a geometer,” as Plato and Kepler said. No, Kant’s solution is: Not only are geometrical principles innate in our thoughts, they are also innate in our perceptions.
Aha, plot twist! The so-called success of mathematics in the real world is no miracle. It’s a rigged game. Our eyes and our senses are biased. They can only see Euclidean geometry.
It’s an illusion to think that we can compare our mathematical deductions with reality. We think we can “test” whether theoretically established theorems are true or not in the physical world; for example, by measuring the sides of right-angle triangles and comparing the results to what Euclid says it should be. But we were naive to think that these two things were really independent. Just as our thoughts are shaped by our innate intuitions, so also our perceptions of physical reality are shaped by the same intuitions.
We don’t have direct access to “raw data” about the physical world. When we think we observe the world, we really observe an interpreted version of the world. The mind can only process information that is interpreted or converted to fit a particular format. In terms of geometry, that means Euclidean geometry.
We see the world through Euclid-colored glasses. Like those pink sunglasses that John Lennon used to wear. Of course if you wear pink glasses, then everything looks pink. But of course John Lennon could not say: aha, I told you, the world is rosy; I argued theoretically that the world should be rosy, and now I have confirmed by observation of the actual world is in fact rosy, because look how everything is pink. John Lennon would be fooling himself if he argued that way.
Euclidean geometry is exactly like that, according to Kant. With experience and observation, we do not discover that Euclidean geometry applies to the real world. What we really observe is our own biases. We discover not facts about the world, but facts about what kind of glasses we are wearing.
John Lennon made the world rosy by putting on his glasses, and in the same way we make the world Euclidean by a hidden lens in the mind’s eye.
Think of a chili pepper. This pepper proves that Kant was right. How so? Think about it. What are the characteristics of a chili pepper?
It’s red. What is red? Is the pepper “really” red? That is to say, is its redness an objective property of reality? Well, yes and no, right? The way science accounts for colors is in terms of wave length of light. What we in our minds regard as red correspond in reality to a particular frequency of light waves.
So there’s something there corresponding to red, but our minds put a major interpretative spin on it. Red is really just a wavelength, “just as number,” so to speak. But our minds turn it into something more qualitative.
And the mind is very selective as well. The remote control of your TV sends its signal using infrared light, which the minds chooses not to see at all. Even though infrared light is exactly the same kind of thing as the color red from the pepper. They are exactly the same kind of waves, only with slightly different frequencies. “Objectively” they are very similar. But subjectively, in our minds, they couldn’t be more different. One is the color red that is one of the fundamental categories in terms of which we navigate reality, and the other is completely invisible.
So perception is very far from direct access to “objective” raw data. The mind interferes very heavily: it selects, transforms, distorts. Maybe when we think Euclidean geometry fits reality it’s only because of this. Only because things that don’t fit a Euclidean mold are as invisible to us as infrared light.
The chili pepper is also very hot to taste. Again, is that an objective property of pepper itself, or is it just a subjective matter of how it interacts with our tongues?
In fact, birds eat chili peppers and to them they are not hot. Chili peppers evolved to be hot because it’s better to be eaten by birds than to be eaten by mammals. The seeds spread better that way. So chili peppers developed this characteristic of being repellently hot to mammals like us, but but perfectly appetizing to birds.
Could it be the same with our geometrical intuitions? “Two lines cannot enclose a space,” Euclid says. The very thought is repellent! Well, maybe that’s just our subjective experience, just as we find it repellent to bite into a hot pepper. Maybe other creatures have completely different geometrical intuitions. Just as birds think pepper are not hot, maybe they also think space has five dimensions or whatever. Who knows.
Different animals can have all kinds of different intuitions and modes of perception that fits the way they navigate the world, the way they find food, and so on. Maybe Euclidean geometry is just something that happened to be convenient to us, just as night vision is convenient to a cat, or a heightened sense of smell is to a dog.
That would make geometrical experience quite subjective. Or at least subjectively contaminated, or entangled with subjective factors.
This is how Kant is able to bridge the gap between rationalism and empiricism. We can sit in a closed room and figure out in our heads geometry that then turns out to be true in the real world. Because, says Kant, what is really happening is that the mind is analyzing itself. What we discover through geometrical meditation is not objective facts, but facts about the geometrical preconceptions hardwired into our minds and the consequences of those conceptions. And these results apply to the world not because they are really out there but because our perception actively processes and converts and interprets any sensory input in Euclidean terms.
As Kant himself says: “Space is not something objective and real; instead, it is subjective and ideal, and originates from the mind’s nature as a scheme, as it were, for coordinating everything sensed externally.” “It is from the human point of view only that we can speak of space, extended objects, etc.”
In the introduction I framed the issue in a way that is flattering to Kant. I said: Everybody knew that you needed some kind of middle ground between rationalism and empiricism, but no one could figure out how to do it. Until Kant, he finally cracked the nut.
But maybe that’s the wrong way to look at it. It often is, this kind of narrative. “Everybody tried to do such-and-such but no one could do it, until finally one guy was smart enough to figure it out.” That’s not usually how history works. If we look into the details we often find that contextual factors explain why key steps happened at certain times.
It’s true that everyone knew that neither rationalism nor empiricism were perfect for hundreds of years. And Kant’s proposal is certainly a very clever way of tackling that problem. So why did it take all the way to the late 18th century before these ideas were proposed?
We already mentioned some relevant factors. Kant makes geometrical knowledge in a sense subjective. That’s a major disappointment, one might say. Most philosophers had certainly hoped to be able to defend a much grander claim. Kant “solves” the rationalism-empiricism problem only by as it were belittling geometrical knowledge, which is a very high price to pay.
The main alternative, as we have seen, was to give God a major role in epistemology. So there’s a trade-off: either you pin geometry to God and you can have it be the most amazing thing, the most perfect knowledge, or else you detach it from God and make it stand on its own legs, but then it’s a lot weaker; it’s a mere subjective human thing and no longer this almighty pinnacle of pure intellect.
The exchange rate, as it were, between these two options fluctuated over time. As God became less popular, the cost of switching to Kantianism went down.
But there’s another reason too why Kant’s theory made more sense in the 18th century than in the 17th. Namely what we said before about how Newton’s science was a blow to rationalism.
We spoke about how that was the case. Rationalism requires knowledge to be generated from within the mind. All knowledge needs to be gradually built up from the most simple intuitions, according to the rationalist point of view. In geometry, that meant ruler and compass and other tools for generating geometrical objects. In physics, it meant contact mechanics; that is to say, seeing complex physical phenomena as an aggregate of lots and lots of little collisions of bodies.
Newton’s physics cannot be reduced to contact mechanics. Or to any other simple intuition. It is in fact counterintuitive. So it cannot be generated from within the mind, through an elaboration in thought of the most undoubtable truths. This is why Newtonian physics is a problem for rationalism.
But the story is a bit more general than that. In fact, Newton’s physics can be seen as a blow to philosophy altogether.
From the rationalist point of view, philosophy comes before science. You start with general philosophical thought. “I think therefore I am”: That’s a very general philosophical truth, and you start there because it’s the most knowable. You start by asking yourself what kinds of things are knowable. From that starting point you arrive at the idea that in physics one of the most primitive knowable things is the contact mechanics of bodies.
From this point of view, philosophy is the boss of science. Philosophy is telling science what to do. Before even starting on science, you have already determined through introspection and meditation what the primitive intuitions of physics are. Any science that follows needs to conform to these predetermined rules that philosophy has established beforehand.
From a rationalist point of view, this makes sense. If knowledge fundamentally comes from within the mind, it makes sense to work from the inside out; to start with the most general philosophical core and then build on that to get to things like physics and other stuff that are more connected to the outside world. That’s a core commitment of the rationalist worldview. This is why it requires philosophy to be prior to science, and the boss of science.
Newton does it the other way around. To him, science is the boss of philosophy. This is a natural consequence of his empiricist, “reading backwards” mindset that we have emphasized before. Thought starts not with inward reflection on our basic intuitions, but in the wild jungle of complex phenomena. Science reasons as it were backwards from there to discover the basics principles, such as axioms of geometry and fundamental laws of physics.
If you continue this process one further step you get to philosophy. Just as the laws of physics are whatever is needed to explain the phenomena, so the principles of philosophy are whatever is needed to make that physics possible. So philosophy is subordinated to science. It doesn’t tell science what to do, but the other way around.
To the rationalists, philosophy set the ground rules that science must obey. To the empiricists, to Newton, philosophy merely describes what assumptions are necessary for science after science has already been established. To the rationalists, philosophy is prescriptive: it gives orders, it says how science has to be. To the empiricists, philosophy is descriptive: it’s an observer, a backseat journalist, that merely says how science is, without having any influence over it.
So we see how the basic outlooks of rationalism and empiricism imply these opposite views of the relation between science and philosophy. And Newton’s physics was extremely successful. So its success lent credibility to the empiricist outlook overall, including the demotion of philosophy.
But in fact this is still not the end of it. There is yet another respect in which Newton’s physics dealt an additional death blow to philosophy. Namely on the issue of absolute versus relative space.
Newton clashed with Descartes and Leibniz on this issue as well. It goes like this. What can we know about the spatial properties of a body, such as its position and velocity?
Descartes and Leibniz were relativists about space. Everything we could ever know about positions and velocities of bodies is relative. That is to say, you can only specify the position or speed of a body by comparing it to another body. The chair is so-and-so far from the table. The train is moving away from the station at such-and-such a speed. You cannot speak of the position of the chair or the speed of the train without comparing it to something. You need to relate it to some reference point.
Descartes and Leibniz insisted on this. Here’s how Descartes puts it: “The names ‘place’ or ‘space’ only designate its size, shape and situation among other bodies.” “So when we say that a thing is in a certain place, we understand only that it is in a certain situation in relation to other things.” Leibniz agreed. “Motion is nothing but a change in the positions of bodies with respect to one another, and so, motion is not something absolute, but consists in a relation.”
It takes two to tango, and it takes two bodies to be able to speak of position and velocity. Because you can only describe the position or velocity of the second body by using the first as a reference point.
If there was only one body in the universe, it wouldn’t make any sense to ask whether it was moving or not. Since there’s nothing to use as a reference point, the very concept of motion becomes meaningless is such a situation. According to the relativist conception of space.
This fits very well with our previous emphasis on operations in geometry. Relative positions and relative velocities correspond very well to operations. You can specify what it means for one object to be so-and-so far from another object, or moving with such-and-such a speed with respect to the other object, in terms of concrete measurements. I take a measuring tape, I stretch it from one to the other, that’s how far apart they are.
If there is only one object in the universe, there is no operation we can perform to check whether it is moving or not. So to introduce the idea of every body having some absolute state of motion, independently of any other body, is equivalent to introducing concepts by means other than operations. We know from geometry that this is dangerous, as we saw with the superright triangle and other examples.
Yet Newton does exactly this. Newtonian physics presupposes absolute space. That is to say, it assumes that every body has some definitive position and velocity, completely independently of any other body, and completely independently of what is measurable or knowable to us.
So from the Newtonian, absolutist point of view, if there is only a single object in the universe, then that object still has some definite velocity. It’s either moving or not. Whether it’s moving or not is physically undetectable. There is no way to tell, with a physical experiment, whether it is moving or not. Nevertheless, the question of whether it is “really” moving or not still makes sense and has a definite answer, according to Newton.
This notion–that any body has an “absolute” position and velocity–is necessary for Newton’s physics. Think of the law of inertia. It says: If there is no outside force acting on a body, then the body keeps going in a straight line with the same speed. Forever. Like a metal ball rolling on a marble table, when there is no friction and no obstacles, it keeps going with the same velocity. Without external influence, the state of motion remains the same.
But note that this law talks about the state of motion of a body without reference to other bodies. The law of inertia presupposes that the body has some inherent velocity, a true velocity. That’s the thing that stays the same in absence of interference. Obviously this is not dependent on some particular reference point. The body in and of itself has a state of motion associated with it. The state of motion of the body is an absolute property, not a relative one.
This clash between the absolute and relative space points of view is another clash between science and philosophy. Relative space is clearly the “best” view in terms of philosophy. The philosophical objections to absolute space are very compelling: Absolute space is unknowable. Absolute space introduces concepts that are empirically untestable, unverifiable, unoperationalisable.
The reply from the other side, from Newton’s side, is not to dispute that philosophy is on the side of relative space. Instead it is to belittle the authority of philosophical arguments. Indeed, absolute space makes no sense philosophically. But the conclusion from this is: tough break for philosophy.
Absolute space is a necessary precondition to state the law of inertia, and the law of inertia is an integral part of Newton’s extremely powerful physics, so inertia and hence absolute space must be accepted. Philosophy is just going to have to deal with it.
So this once again reinforces Newton’s point that philosophy is basically a spectator sport. Philosophy can’t tell science what to do. If philosophy clashes with science, as it does regarding absolute space, then philosophy has to give way.
Physicist Stephen Hawking famously declared that “philosophy is dead.” He had in mind 20th-century developments. That’s how many modern scientists think. But philosophy was dead once before. Newton killed philosophy.
If you want to get somewhere in science and mathematics, you can’t get caught up in pointless speculations and debates about “what it all means.” You just have to do the math, get on with it. That was the case in the 18th century, and again in the 20th century.
Another prominent modern physicist, Lee Smolin, put it as follows: “When I learned physics in the 1970s, it was almost as if we were being taught to look down on people who thought about foundational problems. When we asked about the foundational issues in quantum theory, we were told that no one fully understood them but that concern with them was no longer part of science. The job was to take quantum mechanics as given and apply it to new problems. The spirit was pragmatic; ‘Shut up and calculate’ was the mantra. People who couldn’t let go of their misgivings over the meaning of quantum theory were regarded as losers who couldn’t do the work.”
It was exactly the same thing in the 18th century. Then too scientists and mathematicians figured they were better off just ignoring philosophy. And with good reason since Newton’s physics was an obvious winner in terms of mathematics and science, but a complete non-starter philosophically according to many.
The greatest mathematician and physicist of the 18th century, Euler, realized this perfectly well. He knew that absolute space was junk philosophy but essential to science.
He knew that the law of inertia demanded absolute space. As Euler says: “For if space and place were nothing but the relation among co-existing bodies, what would be the same direction? Identity of direction, which is an essential circumstance in the general principles of motion, is not to be explicated by the relation of co-existing bodies.”
Euler also knew that there were powerful philosophical objections to absolute space. The objections of Descartes and Leibniz that I already mentioned. Let me quote here how Ernst Mach later made the same point in the late 19th century. Mach is basically reviving the 17th-century criticism of absolute space. Here’s how Mach puts it:
“Absolute space and absolute motion are pure things of thought, pure mental constructs, that cannot be produced in experience. [They have] therefore neither a practical nor a scientific value; and no one is justified in saying that he knows aught about it. It is an idle metaphysical conception. All our principles of mechanics are experimental knowledge concerning the relative positions and motions of bodies. No one is warranted in extending these principles beyond the boundaries of experience. In fact, such an extension is meaningless, as no one [can] make [any] use of it.”
Euler and others in the 18th century were aware of this problem with the notion of absolute space that is so essential to Newtonian science. They didn’t know how to solve this philosophical problem, except to ignore philosophy altogether. Euler pretty much says so. Listen to this quote:
“I do not want to enter the discussion of the objections that are made against the reality of [absolute] space and place; since having demonstrated that this reality can no longer be drawn into doubt, it follows necessarily that all these objections must be poorly founded; even if we were not in a position to respond to them.”
So Euler admits that he cannot answer the philosophical objections. Instead his solution is: forget philosophy. Philosophy became obsolete with the Newtonian revolution in science. It was out of touch.
Kant is the savior of philosophy. Kant makes philosophy relevant to science again, after a century of being obsolete. Kant’s theory is a way to bring philosophy up to date with science. It is a philosophy that is compatible with Newtonian science, unlike earlier versions of rationalism.
Against this background we can understand why Kant was willing to make mathematical knowledge subjective. That part of his theory was a huge betrayal of a major tenet of classical rationalism. But times had become desperate enough. Philosophy was the laughing stock of scientists. It had to do something, anything.
So Kant decided to bite the bullet on subjectivity in order to at least salvage something of philosophy. Save what can be saved.
Rationalism had once been a mighty kingdom, but it was bleeding territory. Newton’s science was taking the world by storm, and it seemed a real risk that rationalism would not only lose ground but might even be wiped off the map altogether.
Kant’s plan for saving rationalism shows how far it had fallen. In its glory days, rationalism would have scoffed at the notion that geometry is subjective. But now, it was that or death. Like royalty eating peasants’ porridge, rationalism had to adapt or die. Rationalism had to sacrifice the pride of its forefathers–the objective truth of geometry.
But despite this humiliating concession, Kant’s reinvention of rationalism was an astonishing success. Rationalism was back with a vengeance.
Not only was rationalism no longer obsolete or out of touch with science, it was even ahead of the game. Kant had not only stopped the rot but even brought rationalism back on the winning side. Kant’s account not only showed that some parts of classical rationalism could be saved; it also provided the best available account of how the success of Newtonian science could be explained philosophically. Where people like Euler had merely given up on philosophy because of the magnitude of the problems it faced, Kant had shown that philosophy could answer the challenge and more. Philosophy was relevant again. Philosophy was no longer dead.
 17 November 2021, 11:18 pm
17 November 2021, 11:18 pm - 43 minutes 50 secondsRationalism versus empiricism
Rationalism says mathematical knowledge comes from within, from pure thought; empiricism that it comes from without, from experience and observation. Rationalism led Kepler to look for divine design in the universe, and Descartes to reduce all mechanical phenomena to contact mechanics and all curves in geometry to instrumental generation. Empiricism led Newton to ignore the cause of gravity and dismiss the foundational importance of constructions in geometry.
Transcript
Here’s a fundamental problem in the philosophy of mathematics. You can sit in an isolated room, in an arm chair, and prove theorems about triangles, such as the angle sum of a triangle or the Pythagorean theorem. When you do this, you have the feeling that you have established these results with absolute certainty. You feel that they must be true because of how compelling the proof is. And you feel that you have established this by thought alone, by purely intellectual means.
Mathematics is unique in this respect. In other subjects, thinking is a powerful tool, but it is always supplemented by observation and experience. If you spent your whole life isolated in a locked room, you would not be able to say anything about the laws of astronomy or the anatomy of the digestive system, because without observation, with only pure thought, it is impossible to even get started in those field. But you could figure out everything about triangles. If one day you were released from your prison where you had been sitting for decades, you could go out and measure actual triangles and you would find that, indeed, their angle sum is always two right angles, the Pythagorean theorem always holds for right-angle triangles and so on. Just as you had predicted by pure thought.
This is a bit of a mystery. Because it shows that there are two sides of mathematics that are difficult to reconcile. On the one hand, the internal, mental conviction that mathematics establishes absolute truths purely by reasoning. On the other hand, the external, physical fact that mathematics works in the real world.
What is the bridge between these two worlds? It is as if there is a natural harmony between our minds and the outer world. What is the cause of that harmony?
These two poles can be called rationalism and empiricism. Rationalism takes mathematics to be fundamentally a matter of pure thought. This fits well with the sense we have when doing mathematics, when reading Euclid, that we are establishing absolute truths by sheer reasoning. But it doesn’t explain why mathematics works so well in the physical world.
We have encountered some rationalists already: Plato, Descartes. We saw how Descartes solved the problem. Mathematics is pure thought, and it works in the physical world because the Creator put mathematical ideas in our minds. As the Bible says, “God created man in his image.” That is to say, God created the world based on mathematical ideas, and then created humans and sort of pre-programmed their minds with the same kinds of ideas that he had used to create the world.
So no wonder there’s a harmony between the mental and the physical worlds: they both stem from the same source, the Creator, who used the same principles when designing both. Descartes said basically this quite explicitly, as we recall. Plato pretty much hints at the same idea. God is a mathematician. That is a central belief in Platonist thought as well. And it is a necessary thesis for the rationalists to explain why mathematics works so well.
We have already encountered some empiricist as well: Aristotle, Francis Bacon. They think knowledge ultimately comes from the world around us. From that point of view, it is no mystery that mathematics works on physical triangles. It stems from physical experience to begin with, so of course it conforms to physical experience.
The challenge for the empiricists is instead to explain the mental experience of doing mathematics; our feeling that it brings absolute truth by pure thought in a way that no other subject does. From the empiricist point of view, this feeling is a mistake, a delusion. We think we are doing pure thought, but actually mathematical thought is generalized experience. We think we can sit in a closed room, an arm chair, and figure things out about an outside world that we have never even seen. But it only feels that way.
We have seen and touched many lines and triangles and squares our entire life, since the year we were born. We have internalized this experience. It has become second nature to us. Basic truths of geometry, such as Euclid’s axioms, may feel like core intuitions that are much more pure and absolute and undoubtable than things we know from experience. But that feeling is a delusion, according to the empiricists. Our minds, our feelings have imperfect self-awareness. Just as we are not aware through introspection how our digestive system works, so we are not conscious of the psychological origins of our mathematical intuitions.
I think we can agree that rationalism and empiricism both face big challenges. The challenge for rationalism is to explain why mathematics applies to the physical world. Traditional rationalism had an answer that was very compelling at the time: the explanation in terms of God, the Creator. But nowadays we may want an atheistic answer. And then rationalism is back to square one, facing the original problem all over again, without any solution in sight.
Empiricism doesn’t have that problem, but it has other ones. If mathematics comes from experience, how can it seem so absolute and undoubtable? How can an exact science come from inexact sensory impressions? If mathematics is based on experience like everything else, why does it seem to be such a different kind of knowledge in so many respects? Those are challenges for the empiricist to answer.
It matters how you answer these questions. It shapes the kind of science that you do.
Consider for instance Kepler, the 17th-century astronomer. He was another rationalist. As Kepler says: “Nature loves [mathematical] relationships in everything. They are also loved by the intellect of man who is an image of the Creator.” That’s almost word for word how I described the rationalist position just moments ago.
Kepler felt that the world was designed with the intent that we should study the universe mathematically. As he says: “Whenever I consider in my thoughts the beautiful order [of the universe] then it is as though I had read a divine text, written onto the world itself saying: Man, stretch thy reason hither, so that thou mayest comprehend these things.”
In fact, scientific facts support this view, in Kepler’s opinion. For example, as he says, “Sun and moon have the same apparent sizes, so that the eclipses, one of the spectacles arranged by the Creator for instructing observing creatures in the orbital relations of the sun and the moon, can occur.”
That is indeed a striking fact: that the moon is exactly the right size to precisely block out the sun at the moment of a solar eclipse. From the point of view of modern science, this is a remarkable coincidence. It’s pure chance that the moon is exactly the right size.
You can understand why the explanation in terms of purpose was more compelling in Kepler’s time. Witnessing a solar eclipse is a spiritual experience. It all seems so perfect. Much too perfect to chalk it up to chance. It’s very disappointing that modern science offers nothing more than this non-explanation of such an emotionally compelling spectacle.
And not just modern science. Such views were around already in Kepler’s time. Atomism is a classical worldview that is indeed happy to attribute almost everything, eclipses included, to chance and randomness. According to Kepler’s teacher, Melanchthon, such views “wage war against human nature, which was clearly founded to understand divine things.”
So here we have again that double challenge to empiricism. If mathematics is just one type of knowledge among many that we pick up from experience, then, first of all, why does the universe show so many signs of being mathematically designed? Like the thing with the eclipses, but there are also countless other examples one could use to make this point. Empiricism has no answer to this. It thinks that’s all just a bunch of coincidences, and we are just fooling ourselves by looking for purpose and design that isn’t there.
And secondly, if empiricism is right, and mathematics is just experiential knowledge like everything else, then why does mathematical reasoning feel so uniquely compelling and convincing? As Melanchthon says, mathematics is as natural to a human being as “swimming to a fish or singing to a nightingale.” Just as animals are born with these instincts, so our minds are innately predisposed to do mathematics. Empiricism does not explain why that is the case, or why that seems to be the case.
So it’s understandable that Kepler was a convinced rationalist instead. And this conviction shaped his scientific work. Astronomers are “priests of the book of nature,” as Kepler said. So he was always looking for meaning and purpose and design.
For example, the telescope was a new invention in Kepler’s time, and it was a big moment when the moons of Jupiter were discovered. Kepler immediately looked for the purpose behind the existence of these moons. He concluded that Jupiter must be inhabited. Why else would it have moons? As Kepler says: “For whose sake, the question arises, if there are no people on Jupiter to behold this wonderfully varied display with their own eyes? We deduce with the highest degree of probability that Jupiter is inhabited.”
Another of Kepler’s attempts at uncovering divine design was his theory of planetary distances. According to Kepler, the Creator had chosen the number and position of the planets according to a very beautiful and pleasing mathematical design. Namely, a plan based on the five regular polyhedra.
Euclid discusses the regular polyhedra at length in the Elements. There are precisely five of them, as Euclid indeed proves in the very last theorem of the Elements.
Kepler figured God was as fascinated by these shapes as Euclid had been. So when God asked himself how many planets there should be in the solar system, and how far from the sun to put them, God figured that the most mathematically pleasing way would be to choose six planets, and to have the spaces between them chosen in such a way that the five regular polyhedra fit between them like a nesting doll.
Kepler’s theory in fact fit the data very well. You could calculate planetary distances from astronomical measurements, and you could calculate size proportions of the regular polyhedra from Euclid’s Elements. If you put these things side by side in two columns they come out remarkably close to one another.
So again Kepler explained things that modern science doesn’t explain at all. Why are there six planets? Why are they positioned at those particular distances form the sun? Why does the moon fit precisely on top of the sun during an eclipse?
Kepler explained all of these things. If you accept the basic outlook that it makes sense to think of the creator of the universe as a Geometer, then Kepler’s explanations are very good. This is Kepler, the best mathematical astronomer of his age. These are not some whimsical religious musings. It’s very serious science. Very good science, one might argue.
Meanwhile, modern science doesn’t explain any of these things. There is no explanation, there is no why, according to modern science, of course. It’s all just chance. The solar system was formed by a bunch of random rocks getting caught in a gravitational field. Whatever positions they took up is just random.
It’s easy for us to judge Kepler. But shouldn’t science explain more things as it develops? Not fewer things. You would think that science should take things that are not explained and explain them. Instead of taking things that are already explained and attributing them to coincidence instead. And yet that is precisely what happened when Kepler’s theories were abandoned.
In any case, this Kepler stuff is interesting for all kinds of reasons, but for our purposes, what I wanted to show was that it matters whether you are a rationalist or an empiricist. Rationalism, as we saw, almost requires the hypothesis that God was a Geometer, just as Plato and Descartes and Kepler all said. And that assumption has major implications for how you practice mathematical science. It suggests looking for deliberate design put into the world by a mind that is essentially like our mind, as far as mathematics is concerned.
So that’s one way in which the rationalism-empiricism divide strongly shaped scientific practice in the early 17th century. But that was not the end of it. Here’s another example: the contrasting ways in which Descartes and Newton approached cubic curves.
Cubic curves are the next step beyond conic sections. Conic sections are curves of degree 2. They were studied in great depth by the Greeks. Cubic curves are called cubic because that have degree 3. So they are the more complicated cousins of the conic sections. In the 17th century, this was natural direction to take geometry: to understand curves of degree 3 and higher in the same depth that the Greeks had understood conic sections.
For instance, conic sections come in three classes: ellipse, parabola, hyperbola. Can one find an analogous way of classifying cubic curves? There are going to be more classes because cubics are more complicated. But maybe with the right principle of taxonomy one can impose order among their variety in way that is as useful as the division into ellipse, parabola, and hyperbola is in the theory of conics.
Newton did precisely this. He gave a very detailed and advanced technical study in which he classified cubic curves in several different ways. He divided cubic curves into “species” as he says. That’s Newton’s own term, and it’s a vivid one.
Taxonomising curves into “species” makes Newton sound like a pioneering explorer-scientist forging into unknown jungles and studying all the strange creatures. When you find a new exotic insect, you put it under a microscope and study all its properties. How many legs does it have, how many eggs does it lay, and so on. It’s the same when studying curves. How many crossing points, how many inflections points, and so on. It’s the zoology of mathematics.
This metaphor fits very well with the epistemological ideals of empiricism. You learn by studying the great diversity of things out there. Into the jungle! That’s the call of empiricism. That’s how you learn things. By immersing yourself in the unknown.
“The best geologist is one who has seen the most rocks.” That’s another slogan of empiricism. Experience is the source of knowledge, in other words. If you want to understand rocks, you need to look at a whole lot of rocks. And if you want to understand cubic curves, you need to look at a whole lot of cubic curves, first of all. Once you have built up a store of experience, then maybe you will see some patterns starting to emerge and you can begin the process of systematising or taxonomising the “rocks.”
Empiricism is all about diving in at the deep end and figuring it out as you go. This corresponds to reading Euclid backwards. You start with the complicated stuff, the Pythagorean theorem and such things. Those kinds of things are the exotic beasts that you encounter “in the jungle.” Gradually, you seek to bring order into the chaos by finding general principles that account for the phenomena you observe.
That’s empiricism. And it’s completely backwards according to rationalism. That’s not how you learn things. You can’t start with observations, with the phenomena. Perception is unreliable. Aimless exploration unguided by the intellect is bound to be a waste of time leading nowhere.
The way to knowledge is thinking. To “meditate,” as people used to say. You have heard of Descartes’s Meditations. That’s even the title of one of his works. The source of knowledge is meditation. That is to say, deep thought where you basically close yourself off from the world. Sitting in an armchair in a closed room. That’s where you make progress in understanding, not running around in the jungle.
So Newton’s way to study cubic curves was the empiricist way. Get your machete out and start chopping your way through the thick of it. Eventually you become familiar with all these wild things you encounter, and you start to see what kinds of species there are and how they are related.
Descartes was the opposite of this. A rationalist. Descartes studied cubic curves too, but through meditation. His big book is La Géométrie (1637). He doesn’t study cubics specifically, but all algebraic curves. So curves of any degree, not just degree 3.
Already we see a typical rationalist characteristic: rationalism starts from the general; empiricism starts from the specific.
Rationalists withdraw into meditation because they do not trust individual observations. Thought is more reliable. If you sit back in an armchair and introspect about what is knowable, you are bound to come up with very general and abstract truths: I think therefore I am; the whole is greater than the part; two lines cannot enclose a space. Gradually, you have to work your way from there, step by step, to any specific fact you need to explain. Just as Euclid gradually works his way up to more and more complex and detailed material by starting with very general principles that ultimately entail all the rest.
So the rationalist in interested in all-encompassing abstract law or axioms. It is important to the rationalist that all truths can in principle be deduced from these axioms. But it’s less important to actually do this. The rationalist is most interested in the fundamental axioms or laws, because those are the source of the certainty of knowledge. The specifics derived from them merely inherit their certainty from the certainty of these foundational axioms.
So the very first principles of the entire field is where you need to focus your attention if you are a great rationalist philosopher. And that’s exactly what Descartes does in his book, La Géométrie. Even the title fits with this point of view: The Geometry; it’s a very total, definitive account of geometry as a whole, just as the rationalist epistemological ideal demands.
This is further confirmed in the very first sentence of the text: “All the problems of geometry …”––that’s how Descartes opens his book. He starts with extreme generality, just as rationalism suggests one should. He wants to find the principles that can be used to solve “all the problems of geometry,” in principle.
Descartes doesn’t care so much about the details. He is very keen to explain why his principles are sufficient to solve “all the problems of geometry,” but has very little patience for actually solving any of those problems. This is reflected in the very last sentence of his book.
Descartes writes: “I hope that posterity will judge me kindly, not only as to what I have explained, but also as to what I have intentionally omitted so as to leave to others the pleasure of discovery.”
This is a bit dishonest, of course. He did not omit the details merely out of kindness to the reader, obviously. His focus on the general and lack of interest in the specific is a consequence of his rationalist outlook.
Newton is the opposite. He loves the details; he loves getting stuck in with some obscure technical problem. In fact, his long treatise on cubic curves is full of technical details but he gives very little attention to explaining any general conclusions. It’s hard to see the forest for the trees.
That’s good empiricism, of course. Rationalism thinks you can trust specific results because they are derived from reliable general principles. The certainty of knowledge resides in the axioms, the general principles. That’s where you need to focus your attention to secure the rigour and reliability of reasoning. And that’s what Descartes does.
Empiricism looks at it the other way around. It is the details, the little things, that are the most knowable. Knowledge starts from the directly observed phenomena, with all their specificity. That’s the root of reliability and certainty. Abstract principles are trustworthy only insofar as they are inferred from a large body of facts.
It’s the same in physics. To Newton, the empiricist, the starting points are specific facts. The orbital time of Jupiter, the speed of Saturn. Specific observable facts. You have to start there and then infer general laws like the law of gravity by showing that it fits a long list of facts. It is the specific facts that give credibility to the general law.
Not so to Descartes. The introspective, meditative, rationalist way of doing physics is to figure out first what properties of moving bodies are the most undoubtable. What are the things that are like Euclid’s axioms, but for mechanics?
Descartes did physics exactly this way. In his view, the most undoubtable core principles of physics are the laws of collision of two bodies. If one body bumps into another, what happens? Well, if one is twice as heavy but they have the same speed, then so-and-so happens; if one is twice as heavy but the other is twice as fast, then so-and-so happens; etc. Those are the kinds of principles that Descartes thought one could establish through pure thought and meditation.
Descartes saw this as analogous to Euclid’s geometry. Euclid’s axioms are about lines and circles: the basic building blocks of all geometrical figures. More complex figures are built up from there by combinations of lines and circle, or ruler and compass. In the same way, in physics, complex phenomena can be regarded as ultimately generated by the simple root phenomenon of the collision of two bodies.
Indeed, modern science kind of agrees about that part. If you exhale on a cold day, you breath forms a cloud that moves in complex ways. It seems to flow or float, but really it’s just lots and lots of tiny molecules crashing into each other millions of times, and that gives rise to this kind of flowing pattern that you see on a larger scale.
So simple generative principles can be enough to account for all kinds of things behind their immediate reach, though elaborate repeated composition. Just as lines and circles kind of “give birth” to all geometry, including very complicated shapes that aren’t just round or straight.
Actually, lines and circles are not enough to generate all geometry. They can’t generate cubic curves for example. Descartes is very interested in this issue. And indeed, in his book La Géométrie, he supplements the ruler and compass with another basic generative principle for drawing curves. A kind of linkage principle. You can build a sort of machine that consist of multiple rulers and pegs interlinked in certain ways, and as you push one part of the machine the other parts move in specific ways because of how all the parts are interconnected. An ordinary compass is sort two rulers nailed together. In the same way you can make more elaborate devices composed of more rulers. This gives rise to “new compasses,” as Descartes calls them. And these are sufficient to encompass “all the problems of geometry,” according to Descartes.
In a way it might seem contradictory that it was the rationalists, like Descartes and Leibniz, who were so concerned with the making of geometrical figures with concrete devices. Shouldn’t a proper rationalist hate physical instruments, like Plato did?
But there is no contradiction. Descartes cared about geometrical instruments for theoretical reasons. As I just emphasised, constructions in geometry go naturally with the general rationalist idea of the mind generating all knowledge from within itself. It’s a form of self-reliance. It doesn’t need anything from the outside world.
And earlier we have spoken about how constructions are connected to the epistemological foundations of geometry. Maker’s knowledge. Constructions are the most knowable thing, and the most secure form of geometrical knowledge, protected against many threats of paradoxes and contradictions. So that’s another way in which constructions go well with rationalism, which is of course very much concerned with what are the most undoubtably knowable things.
So these instruments like the ruler and compass and the generalisations of them that Descartes conceived are theoretical, not practical. There’s a funny anecdote that sums this up in the Brief Lives by Aubrey—a late 17th-century collection of biographical stories, maybe not super reliable exactly but this story could very well be true. Here’s what this biographer Aubrey says:
“[Descartes] was so learned that all learned men made visits to him, and many of them would desire him to show them his instruments. He would drawe out a little drawer under his table, and show them a paire of Compasses with one of the legges broken: and then, for his ruler, he used a sheet of paper folded double.”
Quite amusing, and it fits with what I said about the constructions being theoretical.
So we see that the idea of drawing curves with instruments in geometry is analogous to the idea of explaining all of physics in terms of collisions of little bodies. They are both simple, intuitable principles that generate the entire world of phenomena.
From a rationalist point of view, you need such principles. You start in the simple and pure world of meditation and you need to reason your way to the complicated and messy outside world. So you need a bridge that goes from the simple to the complex. Contact mechanics is such a bridge in physics, and ruler and compass is such a bridge in geometry.
But this is only necessary if you are a rationalist. If you insist on starting with pure intuition and thought, then you need such a bridge to the phenomena and the outside world.
But if you are an empiricist you take the outside world—the jungle—for granted as given, as a starting point, so you don’t need to explain how it can be generated by repeated composition of simple principles.
Indeed, Newton rejects both contact mechanics and geometrical constructions at the same time, for precisely this reason.
The fact that these two things are intimately related is not lost on Newton. This is why he starts his big masterpiece on physics by talking about the construction of line and circle in geometry. A very weird way to start a physics treatise to modern eyes, but it makes perfect sense if we keep in mind the background of Descartes and rationalism and everything I just outlined.
I’m referring to Newton’s Principia of 1687. Descartes was long dead by then, but his ideas about the foundations of physics were as relevant as ever. Leibniz, who was a contemporary of Newton, was a rationalist like Descartes. Like Descartes, Leibniz attached great importance to contact mechanics in physics and constructions in geometry.
So when Newton’s Principia came out, Leibniz was very upset that Newton had abandoned the principle of contact mechanics, which was so essential to the entire rationalist worldview. Let me quote Leibniz on this point. Here’s what he said: “A body is never moved naturally except by another body that touches and pushes it. Any other kind of operation on bodies in either miraculous or imaginary.”
Newtonian gravity is precisely one such “other operation”; something that cannot be explained in terms of particles bumping into one another. This is why Leibniz condemns very fiercely the notion of gravity as a foundational principle of physics: “I maintain that the attraction of bodies is a miraculous thing, since it cannot be explained by the nature of bodies.”
That is to say, Newton’s law of gravity cannot be explained or arrived at from a rationalist point of view. Newton in fact agreed. If anything, he makes this point in even stronger terms than Leibniz. Here’s what he says: “It is inconceivable that inanimate brute matter should, without the mediation of something else, which is not material, operate upon and affect other matter without mutual contact, as it must be, if gravitation be essential and inherent in it. That gravity should be innate, inherent, and essential to matter, so that one body may act upon another at a distance through a vacuum without the mediation of anything else, is to me so great an absurdity, that I believe no man who has in philosophical matters a competent faculty of thinking can ever fall into it.”
Very strong words there from Newton. And we can understand why. He wants to discard the rationalist outlook entirely. He is not interested in winning broad support for his theory by trying to argue that it sort of fits with rationalism somehow. He could have given that a shot. He clashed with many influential people: Descartes, Huygens, Leibniz. He could have tried to go a diplomatic route and try to come up with reasons for why his way of doing science was compatible with their rationalist commitments. But he chose not to. This is why he comes on so strongly in these quotes about how gravity is rationally inconceivable and so on.
In this way, Newton moves the conflict into the area of rationalism versus empiricism generally, instead of arguing about the interpretation or meaning of gravity specifically. “With the cause of gravity I meddle not,” says Newton, since “I have so little fancy to things of this nature.”
So what Newton wants to justify is not gravity specifically, but a the empiricist way of doing science generally, in which you don’t care about such questions at all. Questions such as how to give a rationalistic account of gravity, or explaining how a meditating mind in an armchair could arrive at the necessity of the law of universal gravitation. Those questions should simply be ignored, says Newton. Which makes sense from an empiricist points of view, but is sheer madness from a rationalist point of view.
So Newton bites the bullet on the cause of gravity. He says: yeah, I know my physics completely clashes with the core beliefs and methodology of rationalism, but rationalism is wrong anyway.
Now, as I said, the role of contact mechanics in physics is analogous to the role of constructions in geometry. Newton knows this, and this is why, to justify his physics, he starts by talking about how to interpret the role of constructions in geometry. Here is what he says right at the beginning of the Principia:
“The description of right lines and circles, upon which Geometry is founded, belongs to Mechanics. Geometry does not teach us to draw these lines, but requires them to be drawn. For it requires that the learner should first be taught to describe these accurately, before he enters upon Geometry; then it shews how by these operations problems may be solved. To describe right lines and circles are problems, but not geometrical problems. The solution of these problems is required from Mechanics; and by geometry the use of them, when so solved, is shewn. And it is the glory of Geometry that from those few principles, fetched from without, it is able to produce so many things. Therefore Geometry is founded in mechanical practice.”
So that’s a clearly empiricist account of geometry. Not only because it obviously grounds geometry in the physical world, in physical practice and experience. But also because it takes away the idea that the axioms need to be justified by being intuitive and undoubtable. That was important to the rationalists, but Newton does away with that.
This is how Newton can justify that he “meddle not with the cause of gravity.” Geometry likewise doesn’t “meddle” with the construction of curves, but merely postulates their description—in fact, geometry postulates these things precisely “because it knows not how to teach the mode of effection,” just as physics does not know how to teach the cause of gravity.
So Newton has twisted Euclid into support for his physics. This is why the preface to the Principia is about constructions in geometry, such as the ruler and compass of Euclid. If geometry doesn’t really know how to generate these curves, but only takes them for granted and goes from there, then physics can do the same with gravity.
So Newton and Leibniz clashed along such lines. And not only them. One could argue that there’s a geographical element to this divide. Empiricism is to some extent a British movement more generally: not only Newton but also Francis Bacon, John Locke, Wallis—just to name some people we have already encountered before. Meanwhile, Leibniz’s rationalistic tendencies in his science and mathematics were shared by his leading colleagues in Continental Europe, such as Descartes and Huygens.
By way of summary, let me read a passage by Newton on his scientific method, and I will insert comments on how what he says fits exactly with what we have discussed. The passage begins:
“As in mathematics, so in natural philosophy, …”
Already very interesting. In other words, Newton is announcing that his scientific method is based on the method of mathematics; the method of Euclid basically. Ok, so what is this this methodological principle that is common to both mathematics and science? The sentence continues:
“… the investigation of difficult things by the method of analysis, ought ever to precede the method of composition.”
Analysis corresponds to reading Euclid backwards. To analyse is to break down into smaller pieces. Composition corresponds to reading Euclid forwards. To compose is to put simpler pieces together to form more complex results. Newton continues:
“Analysis consists in making experiments and observations, and in drawing general conclusions from them by induction. By this way of analysis we may proceed from compounds to ingredients, and from motions to the forces producing them; and in general, from effects to their causes, and from particular causes to more general ones, till the argument end in the most general.”
“General” indeed: a key words here. From observations, that is to say from specific facts, one infers more general underlying principles. Empiricism goes from the specific to the general; rationalism the other way around.
It is also nice that Newton mentions that analysis goes “from compounds to ingredients”: this is precisely the chemistry or cooking metaphor that we used before when discussing how to read Euclid backwards.
Newton continues:
“This is the method of analysis, and the synthesis consists in assuming the causes discovered, and established as principles, and by them explaining the phenomena proceeding from them, and proving the explanations.”
That is to say, reading Euclid forwards is of course also essential. The method of analysis that the empiricist uses does not dispense with this directions of Euclid; it merely reveals that a preliminary stage is necessary to understand its meaning. It is through the preliminary analysis, the backwards direction of reasoning, that one arrives at the principles—not by direct intuition, as the rationalists would have it. Then the forward direction, the composition or synthesis, proves that these principles really work; that is to say, that they are sufficient to prove everything. That part is the same to both rationalists and empiricists. The key difference is how they account for where the principles or axioms came from.
So those are Newton’s own words, corresponding very closely to the story I have told. Of course Newton and Leibniz and all these guys were acutely aware of all of this. In this way they were much more philosophically conscious than most scientists of later ages. And clearly it shaped their science very profoundly, as I have shown by several examples. So that’s all the more reason to keep pursuing these questions. As indeed we will.
 18 September 2021, 6:27 am
18 September 2021, 6:27 am - 33 minutes 47 secondsCultural reception of geometry in early modern Europe
Euclid inspired Gothic architecture and taught Renaissance painters how to create depth and perspective. More generally, the success of mathematics went to its head, according to some, and created dogmatic individuals dismissive of other branches of learning. Some thought the uncompromising rigour of Euclid went hand in hand with totalitarianism in political and spiritual domains, while others thought creative mathematics was inherently free and liberal.
Transcript
Gothic architecture is known for its pointed arches. Unlike round arches like a classical Roman aqueduct for example. Those are semi-circular, but Gothic arches are steeper, pointier. Gothic buildings, cathedrals, have these arches everywhere: windows, doorways, and so on.
Gothic arches consist of two circular arcs. You can make it like this. First make a rectangular shape. Like a plain window or door. A boring old rectangle. Now let’s spice it up. Take out your compass, and put it along the top side of the rectangle. Draw two circular arcs going up above the rectangle. Use the top side of the rectangle as the radius, and its two endpoints as the two midpoints of the two arcs you are drawing. The two arcs make a pointed extension of the rectangle. Now you have your Gothic window.
If you have your Euclid in fresh memory you will recognize at once that this is precisely the type of construction involved in Proposition 1 of the Elements. Coincidence? No, I don’t think so. The Gothic style of architecture arose in Europe in the early 12th century, within a decade or two of the first Latin translation of Euclid’s Elements. If that’s not cause and effect, it‘s an incredible coincidence.
There is little direct documentation about this, but, I am quoting now from Otto von Simson’s book The Gothic Cathedral, “at least one literary document survives that explains the use of geometry in Gothic architecture: the minutes of architectural conferences held in 1391 in Milan. The question debated at Milan is not whether the cathedral is to be built according to a geometrical formula, but merely whether the figure to be used is to be the square or the equilateral triangle. The minutes of one particularly stormy session relate an angry dispute between the French expert, Jean Mignot, and the Italians. Overruled by them on a technical issue, Mignot remarks bitterly that his opponents have set aside the rules of geometry by alleging science to be one thing and art another. Art, however, he concludes, is nothing without science, ars sine scientia nihil est. This argument was considered unassailable even by Mignot’s opponents. They hasten to affirm that they are in complete agreement as regards this theoretical point and have nothing but contempt for an architect who presumes to ignore the dictates of geometry.”
So the geometrical ethos was very strong indeed. This hardline view probably softened a bit over time. Renaissance art is more expressive, emotive, more alive, one might say, than this rigid late medieval stuff. That’s if we fast-forward two hundred years from these Gothic conferences about how art is nothing without geometry.
Then you have people like Michelangelo who said: “the painter should have compasses in his eyes, not in his hands.” I suppose it means that art should go a little more by feeling and intuition, and not be completely dictated by mathematics. But you still have “compasses in your eyes,” so there’s still a very significant role for geometry, it seems.
It is also revealing, perhaps, that Michelangelo thought it was important to point this out at all. I guess there were a lot of artists with compasses in their hands running around back then. Why else would Michelangelo feel the need to criticise that practice?
In fact, geometry proved useful to art again, in new ways, in the Renaissance. At this time artists discovered (or perhaps rediscovered) the geometrical principles of perspective.
Accurate representation of depth in a painting follows simple geometrical principles. The key construction is that of a tiled floor. Like a chessboard type of pattern of floor tiles, but seen in perspective, so tiles that are further away appear smaller in the picture. There are a lot of tiled floors in Renaissance art, because they are great for conveying a sense of depth.
You draw it like this. Draw two horizontal lines: one is where the floor starts, and one is the horizon. Divide the floor line into many equal pieces, representing the size of the tiles. Connect all these points to a fixed point on the horizon. This is because all the parallel rows of the floor will appear to converge in one point, just as whenever you are looking at parallel lines that go off into the distance, such as railroad tracks for instance, they appear to meet at the horizon.
Next, draw a second horizontal line representing the other edge of the front row of floor tiles. Now here comes the magic step. Draw the diagonal of the first tile, and extend it. Where this line cuts the other lines you have already drawn, those are all corners of other tiles.
This is because of the geometrical principle that a straight line, no matter how you look at it, from whatever angle, will always still be straight. A lot of stuff looks funny in perspective: big things can look small, parallel lines appear to meet, perfectly round things appear to be oval, and so on. Perspective distorts shapes in all kind of ways. But straight lines remain straight lines. That is an invariant in all of this. This is why the diagonal of the first floor tile is also the diagonal of successive tiles. Since that makes a straight line in the real world, it must make a straight line in the picture as well.
Once you have this diagonal it is easy to complete the rest of the floor. It looks great. It creates a photorealistic sense of depth. No wonder so many artists chose to set their scenes in locales that just happened to have lots of tiled floors.
But the insight is much deeper than this. It’s not really about tiled floors; it’s about the correct perspective representation of depth and size generally. Even if you don’t want a tiled floor in your finished picture, it’s still very useful to draw one in pencil on your canvas as a reference grid. You can use the tiles as a guide to transfer and compare sizes at different depths and distances. Then later you can paint it over with some trees or whatever, so nobody can see the grid anymore; you just used it behind the scenes to get the proportions right.
The Greeks probably knew about this stuff, but basically no paintings from antiquity have been preserved. But we know they were skilled artists. One guy is said to have painted grapes so realistically that birds came and pecked at it.
It seems the Greeks knew the geometrical principles of perspective and used it to creates scenes for the theatre for example. As Vitruvius says, “by this deception a faithful representation of the appearance of buildings might be given in painted scenery, so that, though all is drawn on a vertical flat facade, some parts may seem to be withdrawing into the background, and others to be standing out in front.”
This wasn’t just a party trick to the Greeks. It also had philosophical implications. To Plato they raised profound epistemological conundrums. He was concerned that optical illusion painting has “powers that are little short of magical,” “because they exploit this weakness in our nature,” bypassing “the rational part of the soul.” The solution to this problem, as Plato saw it, was a solid mathematical education. Since “sense perception seems to produce no sound result” with these illusory paintings, “it makes all the difference whether someone is a geometer or not.”
“The power of appearance often makes us wander all over the place in confusion, often changing our minds about the same thing and regretting our actions and choices with respect to things large and small.” “The art of measurement,” by contrast, “would make the appearances lose their power” and “give us peace of mind firmly rooted in the truth.”
Those are all Plato’s words. A rousing case for mathematics! But Plato perhaps drew his conclusions a step too far, rejecting categorically the role of observational data in science: “there’s no knowledge of sensible things, whether by gaping upward or squinting downward.” Science must be based on “the naturally intelligent part of the soul,” not observation. For example, “let’s study astronomy by means of problems, as we do geometry, and leave the things in the sky alone.” With such attitudes, perhaps it is no wonder that the Greeks excelled more in mathematics than in the sciences. But indeed the threat of optical illusions is a legitimate argument in Plato’s defense.
When the principles of perspective were rediscovered in Renaissance Italy, they were again at the heart of scientific developments, but this time on the side of empirical science. Galileo looked at the moon through a telescope and concluded that it had mountains and craters. Of course the image one sees through a telescope is flat. But mountains and craters are revealed by the shadows they cast.
This is not necessarily very easy to see, or not necessarily a very evident conclusion. Some scholars have argued that the artistic tradition, and its extensive study of perspective and shadows, was a necessary training for the eye to be able to correctly interpret the telescope data.
Is it a coincidence that Galileo the telescopic astronomer came from the same land as the great Italian Renaissance painters? Galileo was born and raised in Tuscany, right where so many of these masters had worked. Maybe only someone immersed in this artistic culture had the right eyes to interpret the heavens. A far-fetched theory, in my opinion, but it’s a nice story.
Let’s put aside the art stuff now and look at another theme in how mathematics was received in the early modern world. Namely, the status of mathematics in relation to other fields. Geometry carried a certain authority. This led to many tensions.
Let’s jump right into the action, with an eyewitness report from 1703. “There has been much canvassing and intrigue made use of, as if the fate of the Kingdome depended on it.” “On the eve of Newton’s election as president [of the Royal Society], matters had deteriorated to such an extent that various fellows could be restrained only with difficulty from a public exchange of blows (or, in one case, the drawing of swords).”
Yikes. So what was this conflict on which “the fate of the Kingdome” depended? It was a battle between the mathematical and the non-mathematical sciences within the Royal Society in London.
The “philomats” who identified with Newton thought the non-mathematical sciences were hardly science at all. Botany, geology, stuff like that. They just collect data and write down obvious things. There’s no real thinking involved, no advanced theoretical progress, no genius.
Here’s how they put it, when they made the case that Isaac Newton, the great mathematician, ought to be the new president of the society to ensure its intellectual quality: “That Great Man [Newton] was sensible, that something more than knowing the Name, the Shape and obvious Qualities of an Insect, a Pebble, a Plant, or a Shell, was requisite to form a Philosopher, even of the lowest rank, much more to qualifie one to sit at the Head of so great and learned a Body.”
So science is divided into two camps: mathematical geniuses like Newton, and then people who just know the names of a bunch of insects.
As you can imagine, the other side saw it rather differently. They identified with Francis Bacon, who had complained about “the daintiness and pride of mathematicians, who will needs have this science almost domineer over Physic. For it has come to pass, I know not how, that Mathematics and Logic, which ought to be but the handmaids of Physic, nevertheless presume on the strength of the certainty which they possess to exercise dominion over it.”
So mathematicians have an inflated ego. They are so full of themselves that they think they have the right to tell others how to think.
Here’s how this point was put in 1700: “The World is become most immoderately fond of Mathematical Arguments, looking upon every thing as trivial, that bears no relation to the Compasse, and establishing the most distant parts of Humane Knowledge, all Speculations, whether Physical, Logical, Ethical, Political, or any other upon the particular results of number and Magnitude. In any other commonwealth but that of Learning such attempts towards an absolute monarchy would quickly meet with opposition. It may be a kind of treason, perhaps, to intimate thus much; but who can any longer forbear, when he sees the most noble, and most usefull portions of Philosophy lie fallow and deserted for opportunities of learning how to prove the Whole bigger than the Part.”
So mathematics corrupts mind and soul by fostering delusions of grandeur, and by focusing on obscure technical questions instead of on what is really important.
Roger Ascham made a similar point in 1570: “Some wits, moderate enough by nature, be many times marred by over much study and use of some sciences, namely arithmetic and geometry. These sciences sharpen men’s wits over much. Mark all mathematical heads, which be wholly and only bent to those sciences, how solitary they be themselves, how unapt to serve in the world.”
Meanwhile, the mathematicians, for their part, thought that an exclusive focus on the merely practical is anti-intellectual and beneath a true thinker. Others scientists may use basic mathematics, but the real accomplishment is to understand it.
Mathematician William Oughtred put it like this: “The true way of Art is not by Instruments, but by demonstration. It is a preposterous course of vulgar Teachers, to beginne with Instruments, and not with the Sciences, and so to make their Schollers onely doers of tricks, and as it were jugglers.”
Very relatable for a modern mathematics teacher. Students are so dependent on calculators that they are “onely doers of tricks.” That’s what you get when mathematics is not respected as an end in itself, but only as a tool for what is practically useful.
There’s an interesting twist to this story though. Part of what these opponents of mathematics were attacking was the pedantic focus on theoretical subtleties. Instead of tackling real problems, mathematicians sit around and muse about nuances of definition and postulates that only matter for very subtle foundational debates, not for actual problem solving. A valid critique, you might say, after reading Euclid with all his foundational pedantry.
But here’s the twist: Many mathematicians didn’t like that stuff either. Many mathematicians in the 17th century felt that the Greek geometrical style was much too formal. They recognized the value of the Euclidean style for foundational investigations, but they felt that creative mathematics must be much more free and loose.
Here’s how Clairaut put it in the 18th century:
“[Euclid’s] geometry had to convince stubborn sophists who prided themselves on refusing [to believe] the most evident truths. It was necessary then that geometry have the help of forms of reasoning to shut the idiots up. But times have changed. All reasoning which applied to that which good sense knows in advance is a pure loss and serves only to obscure truth and disgust the reader.”
This fits pretty well with what we have said about the Greek context. Euclid’s special style of geometry arose in a critical philosophical climate. Mathematicians had to anticipate attacks from philosophers who wanted to undermine the entire notion that geometrical reasoning was a rigorous way of finding truth.
Without this external pressure from philosophy, mathematicians may have been happy with a much more informal style, as they were in other cultures and societies. And as indeed they became again in the 17th century.
Almost all mathematicians in the 17th century were very happy to take a freewheeling approach for example when exploring a lot of stuff related to what we call calculus today. For example, John Wallis, a leading mathematician, did work on infinite series that was based on daring, unrigorous extrapolations and generalisations, which he considered “a very good Method of Investigation which doth very often lead us to the early discovery of a General Rule.” In fact, “it need not any further Demonstration,” according to Wallis.
This is very unlike Euclid or anything you find in Greek sources. It’s explorative trial and error, and a readiness to trust the patterns and rules you discover without the minutiae of carefully writing out meticulous proofs of every little thing.
When mathematicians chose this approach, they did not think of themselves as going against the ancient tradition. Instead they imagined—and they were probably right, of course—that Greek mathematics too would have been developed this way, in an informal way.
Euclid’s style of mathematics is very powerful for certain foundational purposes, but of course Euclid’s proofs do not reflect how people initially discovered these things. There must have been an exploratory side to Greek mathematics that is not revealed in surviving sources.
Euclid’s Elements is the end product of a long process of discovery and exploration. That process would not have been conducted in the pedantic and overly polished style of the finished Elements. It is necessary to start with a much freer creative phase. Then its fruits can be systematized and analyzed in the manner of Euclid.
Torricelli, for example, expressed a view typical among 17th-century mathematicians: “For my part I would not dare to assert that this Geometry of Indivisibles is a thoroughly new invention. Rather, I would have believed that the old geometers used this one method in the discovery of the most difficult theorems, although they would have produced another way more acceptable in their demonstrations, either for concealing the secret of the art or lest any opportunity for contradiction be proffered to envious detractors.” Many mathematicians agreed with Torricelli on this point.
The Greek historian Herodotus says about Persian political leaders that they “deliberate while drunk, and decide while sober.” That’s how you have to do mathematics too. First you need to generate ideas. For this you have to be “drunk,” that is to say, try out wild ideas, be uninhibited. Then you have to go over the same material again while “sober”: that is to say, you scrutinize everything critically, discarding and correcting all the mistakes you made while “drunk.”
The documentation we have for Greek mathematics is only the “sober” part. But there must have been a “drunk” part too. The sober part is what gives mathematics its distinctive precision and exactness and reliability. But the sober part alone is sterile. It needs the fertile input of daring ideas from the drunk part. Creative mathematics requires both.
Note that if you want to create new mathematics, then this is essential to realize. So working mathematicians, research mathematicians, will absolutely agree with his.
But many people in the 17th century wanted to use the example of mathematics to support various agendas, without having any interest in discovering new mathematics. From that point of view, it is possible to ignore the drunk phase. If you are merely preserving and admiring past mathematics, and you don’t need creativity, you don’t need new ideas, then you can stick entirely to the sober mode, the Euclidean mode, and maintain that that alone is the essence of mathematics.
This matters if you want to use the authority and status of mathematics to legitimate other, non-mathematical agendas. Indeed, it suited some people very well in the 17th century to emphasise the “soberness” of Euclid. They wanted mathematics to be like that, because they had political or philosophical ideals that fit that image.
Amir Alexander’s book Infinitesimal has some nice examples of this. Let’s look at those. I mentioned Wallis as an example of a creative mathematician who very much embraced the “drunk” style of mathematics. His arch enemy was Hobbes, who, by contrast, appealed to the authority and rigour of Euclidean geometry as a model for reasoning as well as political organisation.
As Amir Alexander says: “Wallis and Hobbes both believed that mathematical order was the foundation of the social and political order, but beyond this common assumption, they could agree on practically nothing else. Hobbes advocated a strict and rigorous deductive mathematical method, which was his model for an absolutist, rigid, and hierarchical state. Wallis advocated a modest, tolerant, and consensus-driven mathematics, which was designed to encourage the same qualities in the body politic as a whole.”
Wallis’s vision of mathematics was very agreeable to the experimental scientists of the Royal Society. “Experimentalism is a humbling pursuit, very different from the brilliance and dash of systematic philosophers such as Descartes and Hobbes. It ‘teaches men humility and acquaints them with their own errors’. And that is precisely what the founders of the Royal Society liked about it. Experimentalism ‘removes all haughtiness of mind and swelling imaginations’, teaching men to work hard, to acknowledge their own failures, and to recognize the contributions of others.”
“Mathematics, [the Royal Society founders] believed, was the ally and the tool of the dogmatic philosopher. It was the model for the elaborate systems of the rationalists, and the pride of the mathematicians was the foundation of the pride of Descartes and Hobbes. And just as the dogmatism of those rationalists would lead to intolerance, confrontation, and even civil war, so it was with mathematics. Mathematical results, after all, left no room for competing opinions, discussions, or compromise of the kind cherished by the Royal Society. Mathematical results were produced in private, not in a public demonstration, by a tiny priesthood of professionals who spoke their own language, used their own methods, and accepted no input from laymen. Once introduced, mathematical results imposed themselves with tyrannical power, demanding perfect assent and no opposition. This, of course, was precisely what Hobbes so admired about mathematics, but it was also what Boyle and his fellows feared: mathematics, by its very nature, they believed, leads to claims of absolute truth, dogmatism, threats of tyranny.”
But note that this image of mathematics as totalitarian and absolutist is linked to its sober phase. By playing up the liberal, drunk way of doing mathematics, one changes its political implications.
So that’s how things played out in England. Conservatives appealed to Euclid’s rigour to justify hardline reactionary politics, while creative mathematicians saw the freedom of creation and discovery in mathematics as suggesting that society as a whole should have a high tolerance for unconventional ideas and novel approaches.
The situation in Italy was quite analogous. The Jesuits were the intellectual leaders of the Catholic world in the 17th century. They ran hundreds of colleges across Europe, notable as much for their “sheer educational quality” as for their doctrinal role “in the fight to defeat Protestantism.”
The Jesuit colleges placed great emphasis on Euclidean mathematics, which to them “represented a deeper ideological commitment. Geometry, being rigorous and hierarchical, was, to the Jesuit, the ideal science. The mathematical sciences that followed—astronomy, geography, perspective, music—were all derived from the truths of geometry. Consequently, [the Jesuit] mathematical curriculum demonstrated how absolute eternal truths shape the world and govern it,” thereby serving as a model for their religious doctrine and worldview. “Euclidean geometry thus came to be associated with a particular form of social and political organization, which the Jesuits strived for: rigid, unchanging, hierarchical, and encompassing all aspects of life.”
For this reason, “the Jesuits reacted with fury to the rise of infinitesimal methods”—which is “drunk” mathematics. “The mathematics of the infinitely small was everything that Euclidean geometry was not. Where geometry began with clear universal principles, the new methods began with a vague and unreliable intuition that objects were made of a multitude of minuscule parts. Most devastatingly, whereas the truths of geometry were incontestable, the results of the method of indivisibles were anything but,” thereby undermining “the Jesuit quest for a single, authortized, and universally accepted truth.”
Thus infinitesimal mathematics was dangerous to the Jesuits not for intrinsic mathematical reasons but because it was associated with diversity of thought unchecked by authority. As one Jesuit leader declared: “Unless mind are contained within certain limits, their excursions into exotic and new doctrines will be infinite, [which would lead to] great confusion and perturbation to the Church.”
One God, one Bible, one Euclid. Set in stone for all eternity. That’s what these guys wanted, and that’s why they liked Euclid. And that’s why, “in a fierce decades-long campaign, the Jesuits worked relentlessly to discredit the doctrine of the infinitely small and deprive its adherents of standing and voice in the mathematical community.” You have to stifle this dangerous new “drunk” mathematics, in which people think for themselves, explore diverse perspectives, and look for new truths (as if there was such a thing!).
So, in summary, mathematics had many possible connotations that could be exploited to various ends. It’s like when someone becomes a celebrity, everyone wants them to endorse their product or sign their petition and so on. A sponsored post on their Instagram is prime real estate. Mathematics had become a celebrity in the 17th century. It had status, for better or for worse. And everyone wanted a piece of it. Coke or Pepsi, PC or Mac—who would get the coveted endorsement of mathematics? Mathematics never sold out or picked a side, but it’s illuminating to see the pitches the PR departments of all these various movements made on its behalf.
 10 July 2021, 8:00 am
10 July 2021, 8:00 am - 49 minutes 29 secondsMaker’s knowledge: early modern philosophical interpretations of geometry
Philosophical movements in the 17th century tried to mimic the geometrical method of the ancients. Some saw Euclid—with his ruler and compass in hand—as a “doer,” and thus characterised geometry as a “maker’s knowledge.” Others got into a feud about what to do when Euclid was at odds with Aristotle. Descartes thought Euclid’s axioms should be justified via theology.
Transcript
Everybody has seen the painting The School of Athens, the famous Renaissance fresco by Rafael. It shows all the great thinkers of antiquity engaged in lively intellectual activity. Plato and Aristotle are debating the relative merits of the world of ideas and the world of the senses, both gesticulating to emphasize their point. Others are absorbed in other debates and lectures, somebody’s reading, somebody’s writing.
But here’s something most people don’t notice in this painting. There is one and only one person in this entire pantheon who is actually making something. Everybody is thinking, arguing, reading, writing. Except Euclid. Euclid is drawing with his compass. He is producing the subject matter he is studying. He is active with his hands. He’s practically a craftsman among all these philosophers.
In the ancient world, the mathematician is the maker. Geometry is the most hands-on of all the branches of philosophy and higher learning.
Today the cliche is that a math nerd is almost comically feeble in anything having to do with physical action.
But ancient geometry was in the thick of the action. You had to roll up your sleeves to do geometry. Even in theoretical geometry you would constantly draw, construct, work with instruments. It was a short step to engineering. The greatest ancient mathematician, Archimedes, is almost as famous for his feats in engineering. Such as mechanical devices for lifting and moving heavy objects, and for transporting water. Archimedes and other mathematicians were also at the front lines of war, building catapults and many other warfare machines according to precise calculations. They were architects. The Hagia Sophia in Istanbul for example, was designed by a mathematician, Isidore, who had written an appendix to Euclid’s Elements.
In early modern modern times, like the 17th century, this link between mathematics and concrete action was well understood and appreciated.
Francis Bacon was sick of traditional philosophy because “it can talk, but it cannot generate.” This frustration led him to the radical counterproposal: to know is to do. “What in operation is most useful, that in knowledge is most true.” And on the other hand “to study or feign inactive principles of things is the part of those who would sow talk and nourish disputations.” So we have to condemn much traditional philosophy and turn more to action, to doing.
Perhaps the most important difference between ancient mathematics and ancient philosophy is precisely this. That mathematics is active, while philosophy merely “sows talk and nourishes disputations.” Perhaps that is the explanation for why mathematics proved so fruitful, still thousands of years later, both for intricate theory, such as planetary motions, and for practice, such as engineering, navigation, and so on. Try doing that with Aristotle’s doctrine of causes or Plato’s theory of the soul. Those things are great for “sowing disputations” but if doing is the goal then you can’t get much mileage out of them.
Thomas Hobbes, another famous 17th-century philosopher, very much agreed with this analysis. Hobbes famously declared that “Geometry is the only science that it hath pleased God hitherto to bestow on mankind.” How so? What makes geometry different from all other branches of philosophy and science?
Constructions, of course. Hobbes is very explicit about this. “If the first principles contain not the generation of the subject, there can be nothing demonstrated as it ought to be.” This is what makes mathematics different. Its principles contain the generation of the subject: Euclid’s postulates correspond to ruler and compass, and these are tools that generate the figures that geometry is about.
All philosophical and scientific theories are based on some assumptions or axioms. But they are not generative axioms. They are not a recipe for producing everything the theory talks about from nothing.
In this light we can readily appreciate for instance Hobbes’s otherwise peculiar-sounding claim that political philosophy, rather than physics or astronomy, is the field of knowledge most susceptible to mathematical rigour. Here’s how he puts it:
“Of arts, some are demonstrable, others indemonstrable; and demonstrable are those the construction of the subject whereof is in the power of the artist himself, who, in his demonstration, does no more but deduce the consequences of his own operation. The reason whereof is this, that the science of every subject is derived from a precognition of the causes, generation, and construction of the same; and consequently where the causes are known, there is place for demonstration, but not where the causes are to seek for. Geometry therefore is demonstrable, for the lines and figures from which we reason are drawn and described by ourselves; and civil philosophy is demonstrable, because we make the commonwealth ourselves.”
As bizarre as this may sound to modern ears, it makes perfect sense when we keep in mind the all-important role of constructions in classical geometry.
Indeed there are many things that only the person who made it truly understand. At this time, the 17th century, various mechanical devices were becoming more common. Such as pocket watches and all kinds of other machines based on gears and cogwheels and so on. The person who made it knows what all the parts are for, but an outsider cannot see this very easily at all. Today another example might be computer programs. The person who wrote it knows how it works, what it can do, how it could be changed, what might cause it to fail, and so on. It would be very difficult for someone else to get a similar sense of how it all works, even if they had access to the code, or they could pop the hood and look at the gears so to speak. Only the maker truly knows: “maker’s knowledge” is a slogan often repeated in the 17th century.
Hobbes took this idea and built a general philosophy from it. His general philosophical program can be read as a direct generalisation of the constructivist precept to the domain of general philosophy. Here’s how Hobbes defines philosophy: “Philosophy is such knowledge of effects or appearances as we acquire by true [reasoning] from their causes or generation.” This is basically a direct equivalent in more general terms of the principle that constructions are the source of mathematical knowledge and meaning.
Indeed, Hobbes explicitly draws out this parallel: “How the knowledge of any effect may be gotten from the knowledge of the generation thereof, may easily be understood by the example of a circle: for if there be set before us a plain figure, having, as near as may be, the figure of a circle, we cannot possibly perceive by sense whether it be a true circle or no. [But if] it be known that the figure was made by the circumduction of a body whereof one end remained unmoved” then the properties of a circle become evident. You understand a circle because you make it, in other words.
Another way of putting it is that “The subject of Philosophy, or the matter it treats of, is every body of which we can conceive any generation.” Just as, classically, the domain of geometry is the set of all constructible figures.
Concepts that are not constructively defined can easily be contradictory or meaningless: a common problem outside of geometry. As Hobbes says: “senseless and insignificant language cannot be avoided by those that will teach philosophy without having first attained great knowledge in geometry.”
Again, as we have discussed before, anchoring geometrical entities in physical reality is a warrant of consistency. Hobbes makes this point as well. “Nature itself cannot err”; that is to say, physical experiences “are not subject to absurdity.”
It is notable that Hobbes and other 17th-century thinkers who invoked geometry did not have in mind simple school geometry and some superficial remarks in Plato or Aristotle. Rather, they were referring to the rich picture of the geometrical method that emerges from a thorough study of advanced Greek geometry and technical writers. When they call upon geometry they mean not some simplistic idea of axiomatic-deductive method but a rich methodology only conveyed implicitly in the finest ancient works of advanced geometry.
This is why the constructive aspect shines through so clearly. It’s importance is evident if you study the mathematicians and build your idea of philosophy of mathematics from there. You’re not going to learn anything about that by reading Plato and Aristotle.
Hobbes is very clear about this. As he says, his philosophy of geometry is “to an attentive reader versed in the demonstrations of mathematicians without any offensive novelty.” Indeed, one must be “an attentive reader,” because one must draw out the philosophical implications left implicit in these sources. And one must be “versed in the demonstrations of mathematicians,” meaning the technical Greek authors. As Hobbes calls them, those “very skillful masters in the most distant ages: above all in geometry Euclid, Archimedes, Apollonius, Pappus, and others from ancient Greece.” This is why Hobbes, in one of his works, “thought it fit to admonish the reader that he take into his hands the works of Euclid, Archimedes, Apollonius, and others.”
Many other 17th-century philosophers picked up the same themes. Some took it to the epistemological extreme of saying that anything other than concrete, specific experience is strictly unknowable. Gassendi, for instance, did not hesitate to take this leap: “Things not yet created and having no existence, but being merely possible, have no reality and no truth.” “The moment you pass beyond things that are apparent, or fall under the province of the senses and experience, in order to inquire about deeper matters, both mathematics and all other branches of knowledge become completely shrouded in darkness.” Mathematical objects must be “considered in actual things”; indeed, “as soon as numbers and figures are considered abstractly then they are nothing at all.” Those are all quotes from Gassendi, and his point of view makes sense. He merely spells out the consequence of taking concrete construction to be essential to knowledge, just as the mathematical tradition suggests.
Other philosophers agreed too. Vico put it like this: “We are able to demonstrate geometrical propositions because we create them; were it possible for us to supply demonstrations of propositions of physics, we would be capable of creating them ex nihilo as well.” So once again the link between creation and knowledge is all-important, and geometry is the key example of this.
Paolo Sarpi made much the same point: “We know for certain both the existence and the cause of those things which we understand fully how to make [just as] in mathematics someone who composes [that is to say, demonstrates synthetically, in the manner of Euclid] knows because he makes.”
It’s striking how many of these early modern thinkers who were well versed in the Greek tradition seized upon the constructive element as the essence of the more geometrico, “the manner of the geometers.”
But there were of course other perspectives on mathematics as well. A lot of people read too much Aristotle and not enough Archimedes. Then as now, one might add. Anyway, these Aristotelians didn’t like mathematics much, and they tried to undermine its authority.
Here is their main point of attack: Mathematical proofs, such as those in Euclid, show that the theorem is true, but not why it is true. In other words, mathematics does not demonstrate “from causes,” as a true science should, according to Aristotle.
Here’s one typical expression of this argument, from Aristotelian philosopher Pereyra in the 16th century:
“My opinion is that the mathematical disciplines are not proper sciences. To have science is to acquire knowledge of a thing through the cause on account of which the thing is. However, the most perfect kind of demonstration must depend upon those things which are proper to that which is demonstrated; indeed, those things which are accidental and in common are excluded from perfect demonstrations.”
Euclid’s geometry is not a “science” in this sense, according to this point of view. For example, Pereyra, says, consider the theorem that the angle sum of any triangle is two right angles (Euclid’s Proposition 32). “The geometer proves [this theorem] on account of the fact that the external angle which results from extending the side of that triangle is equal to two angles of the same triangle which are opposed to it. Who does not see that this middle is not the cause of the property which is demonstrated? [The external angle] is related in an altogether accidental way to [the angle sum of the triangle]. Indeed, whether the side is produced and the external angle is formed or not, or rather even if we imagine that the production of the one side and the bringing about of the external angle is impossible, nonetheless that property will belong to the triangle; but, what else is the definition of an accident than what may belong or not belong to the thing without its corruption?”
So in other words, Euclid’s proof of the angle sum theorem does not reveal the actual reason why the theorem is true. Instead it proves the result via a non-essential thing, the external angle sticking out from the triangle. This external part was obviously added by the geometer quite gratuitously; it’s not essential to the very nature of the triangle. So it’s a kind of artificial trick to add this extra angle and base the proof on it. Truly explanatory and causal demonstrations should not be based on artificial tricks but on what is truly essential to the situation.
Schopenhauer later ranted against Euclid along similar lines. That’s in the 19th century. These ideas were more important and influential in the 16th century, when Aristotelianism was a dominant philosophy. But it’s fun to quote Schopenhauer anyway, because he expresses the same ideas in a charming way. Here’s what he says:
“Perception is the primary source of all evidence, and the shortest way to this is always the surest, as every interposition of concepts means exposure to many deceptions. If we turn with this conviction to mathematics, as it was established as a science by Euclid, and has remained as a whole to our own day, we cannot help regarding the method it adopts, as strange and indeed perverted. We ask that every logical proof shall be traced back to an origin in perception; but mathematics, on the contrary, is at great pains deliberately to throw away the evidence of perception which is peculiar to it, and always at hand, that it may substitute for it a logical demonstration. This must seem to us like the action of a man who cuts off his legs in order to go on crutches.”
“Instead of giving a thorough insight into the nature of the triangle, [Euclid] sets up certain disconnected arbitrarily chosen propositions concerning the triangle, and gives a logical ground of knowledge of them, through a laborious logical demonstration, based upon the principle of contradiction. We are very much in the position of a man to whom the different effects of an ingenious machine are shown, but from whom its inner connection and construction are withheld. We are compelled by the principle of contradiction to admit that what Euclid demonstrates is true, but we do not comprehend why it is so. We have therefore almost the same uncomfortable feeling that we experience after a juggling trick, and, in fact, most of Euclid’s demonstrations are remarkably like such feats. The truth almost always enters by the back door, for it manifests itself per accidens through some contingent circumstance. Often a reductio ad absurdum shuts all the doors one after another, until only one is left through which we are therefore compelled to enter. Often, as in the proposition of Pythagoras, lines are drawn, we don’t know why, and it afterwards appears that they were traps which close unexpectedly and take prisoner the assent of the astonished learner. The proposition of Pythagoras teaches us a qualitas occulta of the right-angled triangle. In our eyes this method of Euclid in mathematics can appear only as a very brilliant piece of perversity.”
So Schopenhauer agrees with the 16th-century Aristotelians that Euclid’s proofs are not explanatory. Instead they proceed by some kind of trick. Euclid is constantly setting logical mousetraps that force the reader to accept the conclusion even though nothing has truly been explained.
It’s interesting though that Schopenhauer uses the example of a machine that is shown to someone who doesn’t know how it was made and therefore is baffled by it and cannot understand how it works. The people of the constructivist tradition we discussed earlier of course used the same image to prove the opposite point: namely that in geometry we are the makers of the machines we use and precisely for that reason that we have genuine knowledge and understanding of it. The people who looked at it that way were basing themselves on mathematical sources. Schopenhauer and the 16th-century Aristotelian who hated mathematics so much were also the ones who knew the least about it. They had not studied the technical Greek writers like Archimedes, Apollonius, and Pappus. Some of these technical sources had not even been translated into Latin yet at the time the Aristotelians were writing in the 16th century. And by the time of Schopenhauer they had been forgotten again among philosophers.
But these Aristotelian guys in the 16th-century also had further interesting arguments to support their point. For example, consider Euclid’s area theorems for parallelograms and triangles in Propositions 35 and 37. The theorems say that same base and same height implies the same area. The first theorem says this for parallelograms and the other one for triangles. The proof of the second theorem is based on the first one: a triangle is just half a parallelogram, so since we already have the result for parallelograms it follows almost immediately that it is also true for triangles.
But we could just as well have done it the other way around: we could have proved the theorems first for triangles, and the infer the result for parallelograms by saying that parallelograms are basically just double triangles.
Euclid chose to start with the parallelogram and then do the triangle, but this was essentially an arbitrary choice. It doesn’t reflect any causal relation. The two theorems are equivalent. It’s not that one of them is more fundamental and therefore explains or causes the other. Neither of the two theorems is more of a cause than the other. So Euclid’s procedure doesn’t fit Aristotle’s decree that demonstrations should proceed from causes.
These guys, like I said, didn’t keep in mind the whole construction business. They were not aware of that because they had not read much mathematics. Later, Leibniz, who knew both the mathematical and the philosophical traditions very well, argued that the construction perspective solves the problem that the Aristotelians raised. Here’s what Leibniz says:
“[Geometry] does demonstrate from causes. For it demonstrates figures from motion; from the motion of a point a line arises, from the motion of a line a surface, from the motion of a surface a body. Thus the constructions of figures are motions, and the properties of figures, being demonstrated from their constructions, therefore come from motion, and hence, from a cause.”
So basing geometry on constructions imposes a natural order—a causal hierarchy, as it were—on its theorems whence Aristotle’s ideal of demonstrative understanding can be maintained. According to Leibniz anyway.
Let’s have a look at Descartes as well. He also had interesting ideas about what made mathematics such a special type of knowledge, and how its success could be emulated in other fields.
In his Discourse on Method of 1637, Descartes explained his philosophical program and how he arrived at it. In an autobiographical introduction he explains:
“I was most keen on mathematics, because of its certainty and the incontrovertibility of its proofs. Considering that of all those who had up to now sought truth in the sphere of human knowledge, only mathematicians have been able to discover any proofs, that is, any certain and incontrovertible arguments, I did not doubt that I should begin as they had done.”
Those are the words of Descartes, famous for doubting everything; his very method has been called the method of doubt. Yet as he himself says: “I did not doubt” that I should follow the mathematicians.
You just had to extend the mathematical method to other areas as well, to philosophy in general. As Descartes says:
“Believing as I did that its only application was to the mechanical arts, I was astonished that nothing more exalted had been built on such sure and solid foundations.”
Just imagine the amazing things that could be achieved if other fields were as successful as mathematics. This was a common sentiment. Here’s how Hobbes put the same point:
“The geometricians have very admirably performed their part. For whatsoever assistance doth accrue to the life of man, whether from the observation of the heavens, or from the description of the earth, from the notation of times, or from the remotest experiments of navigation; finally, whatsoever things they are in which this present age doth differ from the rude simpleness of antiquity, we must acknowledge to be a debt which we owe merely to geometry. If the moral philosophers had as happily discharged their duty, I know not what could have been added by humane Industry to the completion of that happiness, which is consistent with humane life.”
So the goal of philosophy is to be as good as mathematics. So let’s see what Descartes considers to be the foundations of mathematics. He formulates a method for how to philosophise in general, and he intends for this to be a generalization of the mathematical method.
So you might say his methodological program is part descriptive and part prescriptive. It is descriptive because it describes how geometry works; it’s an analysis meant to capture what made Euclid so great. And at the same time it is prescriptive in that it gives orders as to how one should philosophise. Namely, whatever Euclid did in geometry, that philosophers should do in every field, such as physics, ethics, theology, and so on.
Here’s what Descartes says about the axioms or starting points of a theory. We discussed before whether the axioms should necessarily be obvious. Descartes comes down very firmly on that issue.
“The first [principle of my method] was never to accept anything as true that I did not incontrovertibly know to be so; that is to say, carefully to avoid both prejudice and premature conclusions; and to include nothing in my judgements other than that which presented itself to my mind so clearly and distinctly, that I would have no occasion to doubt it.”
So we should start only from the most obvious things, in other words. Things that are so clear that they cannot be doubted. Things known by immediate intuition, in other words. That’s supposed to correspond to the axioms of Euclid.
So Descartes has a lot of faith in innate intuition. As Descartes says, there are “basic roots of truth implanted in the human mind by nature, which we extinguish in ourselves daily by reading and hearing many varied errors.” So this inner “natural light” is more reliable than book learning.
So we should, Descartes says, “conduct thoughts in a given order, beginning with the simplest and most easily understood objects, and gradually ascending, as it were step by step, to the knowledge of the most complex.” And for the sake of this stepwise process, it is necessary to “divide all the difficulties under examination into as many parts as possible.”
You can see how philosophy is going to look a lot like Euclid if people follow these rules that Descartes lays down.
It is interesting that Descartes also specifically says that one should “posit an order even on those [things] which do not have a natural order or precedence.” This is a kind of reply to the Aristotelian point we mentioned above.
The Aristotelians were arguing that when two theorems are equivalent—such as the areas theorems for triangles and parallelograms—then it is artificial and unscientific to impose a particular order that makes one logically prior to the other, as Euclid does. Because then you haven’t given a causal explanation, as Aristotle says one should.
Descartes turns the tables on this. Instead of criticising Euclid when his method seems to go against philosophical sense, he makes Euclid the boss of philosophy. Whatever Euclid does, that’s good method. So if Euclid imposes an artificial logical order on equivalent theorems, then that’s what one should do in philosophy, Descartes concludes.
It goes against Aristotle—so what? Those people I quoted from the 16th century, a hundred years before Descartes, they thought Aristotle had more authority than Euclid, so they used Aristotle to criticise Euclid. Now, a hundred years later with Descartes, it is the other way around. Descartes would rather use Euclid to criticise Aristotle.
A lot had happened in those hundred years. A lot of new science: Copernicus, Galileo, Kepler, etc. Science had made terrific progress by using Euclid and ignoring Aristotle.
By the time of Descartes, the Aristotelians were dinosaurs. Descartes didn’t pull any punches when making this point: he condemned the Aristotelians as “less knowledgeable than if they had abstained from study.”
This new hierarchy, where mathematics has greater authority than philosophy was soon widely accepted. John Locke, the famous philosopher, put it like this half a century later: “in an age that produces such masters as the great Huygenius and the incomparable Mr. Newton, it is ambition enough to be employed as an under-labourer in clearing the ground a little, and removing some of the rubbish that lies in the way to knowledge.” So philosophy is just an under-labourer to mathematical science. The real geniuses, the real creative forces are mathematicians such as Huygens and Newton. Philosophers take on a subordinated role. The task of the philosopher is to explain to others how to follow the lead of the mathematical sciences. This is why Locke calls himself a mere under-labourer.
So that was the general methodological influence of mathematics on Descartes. But Descartes was not content with merely adopting the Euclidean method in philosophy. He also wants to justify this method; to explain why it is so reliable. He does this in his Principles of Philosophy of 1644.
In the very first sentence of this book, Descartes says: “whoever is searching for truth must, once in his life, doubt all things.” As we just saw, in his earlier work he had said that he did not doubt the method of the mathematicians. Now he’s going to fix this gap.
Let’s say you did doubt the mathematical method, the method of Euclid. According to Descartes, as we saw, the foundations of the method was intuition. Euclid starts from axioms such as “if equals are added to equals, the results will be equal.” Intuitively, these basic truths feel completely undoubtable. We are so convinced that they must be true, even though we cannot prove these things.
You might argue: there will always be something we cannot prove. In a deductive system, one thing is deduced from another, but you have to start somewhere. If I tried to prove Euclid’s axioms, I would have to deduce them from something. Whatever those somethings are, they will become the new axioms. So then they have to be assumed. We can never escape this cycle. We always have to assume something.
Unless. Unless we find axioms that are somehow logically self-justifying. This is the idea of the consequentia mirabilis that we discussed before. Axioms can be self-justifying if it is incoherent to try to refute them. If asserting that the axioms is false actually implies accepting the axiom, then the axiom is self-justifying. That way we can find an end to the problem of infinite regress; the problem of always having to prove everything from something else in a never-ending cycle.
This is going to be Descartes solution. He will give an axiom of that type, and then derive the Euclidean axioms from it. Then he will have closed the loop: there are no loose ends, nothing unjustified, anymore.
Here’s the axiom: I think therefore I am. This is the undeniable truth which cannot be denied because denying it would be contradictory.
Here’s how Descartes puts it: “We can indeed easily suppose that there is no God, no heaven, no material bodies; and yet even that we ourselves have no hands, or feet, in short, no body; yet we do not on that account suppose that we, who are thinking such things, are nothing: for it is contradictory for us to believe that that which thinks, at the very time when it is thinking, does not exist. And, accordingly, this knowledge, I think, therefore I am, is the first and most certain to be acquired by and present itself to anyone who is philosophizing in correct order.”
Ok, so that’s the axiom that cannot be denied because to deny it would be contradictory. How are you supposed to prove Euclid’s axioms from there? That seems difficult. How am I supposed from prove geometrical statements from “I think therefore I am”? Well, Descartes has an answer.
“The knowledge of remaining things [including geometry] depend on a knowledge of God,” because the next things the mind feels certain of are basic mathematical facts, but it cannot trust these judgments unless it knows that its creator is not deceitful. “The mind discovers [in itself] certain common notions [such as the axioms of Euclid], and forms various proofs from these; and as long as it is concentrating on these proofs it is entirely convinced that they are true. Thus, for example, the mind has in itself the ideas of numbers and figures, and also has among its common notions, that if equals are added to equals, the results will be equal, and other similar ones; from which it is easily proved that the three angles of a triangle are equal to two right angles, etc.”
But the mind “does not yet know whether it was perhaps created of such a nature that it errs even in those things which appear most evident to it.” Therefore “the mind sees that it rightly doubts such things, and cannot have any certain knowledge until it has come to know the author of its origin.”
So mathematics depends on intuition, and intuition is something implanted into the mind. So God made us have these intuitions. So justifying our innate intuitions depends on the nature of God.
Here is Descartes’s proof that “a supremely perfect being exists”: “That which is more perfect is not produced by a cause which is less perfect. There cannot be in us the idea or image of anything, of which there does not exist somewhere, some Original, which truly contains all its perfections. And because we in no way find in ourselves those supreme perfections of which we have the idea; from that fact alone we rightly conclude that they exist, or certainly once existed, in something different from us; that is, in God.”
“It follows from this that all the things which we clearly perceive are true, and that the doubts previously listed are removed,” since “God is not the cause of errors,” owing to his perfection, because “the will to deceive certainly never proceeds from anything other than malice, or fear, or weakness; and, consequently, cannot occur in God.” “Thus, Mathematical truths must no longer be mistrusted by us, since they are most manifest.”
So, in summary: Euclid’s axioms are true because we innately feel them to be true, and this intuition was implanted into us by God. Our intuition is reliable because God is not a deceiver because he is a perfect being. God must be perfect, because we have the idea of perfection, and we could only get that idea from actual perfection. Since we can conceive of perfection, there must be perfection, there must be a perfect being, a perfect God. That God has hardwired truths such as the Euclidean axioms into our minds. And they must be right because God wouldn’t be perfect anymore if he tricked us by implanting false beliefs in our minds.
That’s Descartes’s argument. I think it’s interesting how we can tell this entire story as driven almost completely by the analysis of Euclid. This whole thing about God and so on it almost like an afterthought, or a minor stepping-stone. The real goal is to justify the geometrical method or explain why Euclid’s axioms should be believed. All this philosophy and theology stuff—I think therefore I am, the existence of God—those are just supporting characters or secondary concerns. Or at least that’s one way of reading Descartes.
In any case, in Descartes as in the previous philosophers we have discussed today, we have seen the very profound influence of ancient geometry. Euclid was still setting the course for philosophy, two thousand years after his death. All the more reason to study him further.
 10 May 2021, 9:54 am
10 May 2021, 9:54 am - 51 minutes 12 seconds“Let it have been drawn”: the role of diagrams in geometryThe use of diagrams in geometry raise questions about the place of the physical, the sensory, the human in mathematical reasoning. Multiple sources of evidence speak to how these dilemmas were tackled in antiquity: the linguistics of diagram construction, the state of drawings in the oldest extant manuscripts, commentaries of philosophers, and implicit assumptions in … Continue reading “Let it have been drawn”: the role of diagrams in geometry10 March 2021, 11:24 am
- More Episodes? Get the App
Your feedback is valuable to us. Should you encounter any bugs, glitches, lack of functionality or other problems, please email us on [email protected] or join Moon.FM Telegram Group where you can talk directly to the dev team who are happy to answer any queries.